nosenote1.dvi

Size: px

Start display at page:

Download "nosenote1.dvi"

かずまさちづ
7 years ago
Views:

2 i

3 Fermi-Pasta-Ulam 8. Fermi-Pasta-Ulam Fermi-Pasta-Ulam Fermi-Pasta-Ulam....4 Fermi-Pasta-Ulam....5 FPU

.3...................... 9.... 9.3 Fermi-Pasta-Ulam....4 Fermi-Pasta-Ulam....5 FPU......................5.... 3.

4 (Molecular Dynamics (MD)) Newton Liouville Euler Euler ( Runge-Kutta Runge-Kutta... 38

3... 5 4.4 Liouville........................ 6 5 7 5......................... 7 5.... 8 5.3... 8 5.4....................... 9 5.5......................... 9 5.6....................... 3 5.

5 Runge-Kutta Runge-Kutta = Runge-Kutta Runge-Kutta Runge-Kutta MD Verlet leap frog Verlet ( ) (6.8) (6.8) Verlet MD MD MD

.3 (6.8)..................... 5 6..4 (6.8)... 54 6..5 Verlet................... 54 6..6...................... 55 6.3... 56 6.4... 56 7 MD 57 7......................... 57 7. MD................... 58 7.

6 Car-Parrinello MD Nosé-Hoover Nos e-poincar e Nos e-poincar e Nos e Nos e-poincar e ζ, s Q Q

5 Nos e-poincar e..................... 7 8.5. Nos e-poincar e Nos e.. 7 8.5. Nos e-poincar e... 73 8.

7 ... C V C V C V T T fitting

8 ....3 Fe Fe O 3 H H = J S i S j (.) {i,j} S i i e Fe 3+ 5/ H I = J {i,j} S iz S jz (.) T c

9 3 T c =0 J j S i S j J j S i S j (.3) T c / M (T c T ) / (.4)..4

10 4 La x Sr x CuO 4 Tc = 38K Tc La Y T c YBa Cu 3 O 7 Tc = 93K..5 )

11 5 CP-PACS GRAPE LSI (Kohn) Car-Parrinello : MD MC ENIAC 948 EDSAC 958

12 6 (LSI 3.5 ) 964 IBM CRAY ( Fermi-Pasta-Ulam 953 Alder

13 7 KdV (963) 970 C(t) = v i (0) v i (t) (.5) 970 Rahman-Stillinger 985 Car-Parrinello ns c.f. Ar ns

14 8 Fermi-Pasta-Ulam. Fermi-Pasta-Ulam Los Alamos No.940(955) Fermi p Fermi-Pasta-Ulam Fermi subsection S(E,V,N) } {{ } = k ln W (E,V,N) } {{ } (.) q p Γ=(p, q) H(q, p) =E( ) p i H(q, p) = m i i } {{ } + Φ(q) } {{ } (.)

15 Fermi-Pasta-Ulam 9.. H = E + E = E (.3) H = E +(E E ), (E E )..3 Fermi.

16 Fermi-Pasta-Ulam 0 x i x 0 x N+ x 0 0, x N+ 0 ẍ n = (x n x n )+(x n+ x n ) = x n+ + x n x n (.4) H = n ẋ n + N+ n= (x n x n ) (.5) y m = N x n = N N n= N m= ( ) nπ x n sin N + m ( ) mπ y m sin N + n (.6) (.7) H = m ω m = sin (ẏ m + ωmym) = A mωm (.8) m mπ, (m =,,,N) (.9) (N +) ÿ m = ω my m (.0) y m = A m cos(ω m t + δ m ) (.)

17 Fermi-Pasta-Ulam x n = N N m= N ( ) mπ sin N + n A m cos(ω m t + δ m ) (.).3 Fermi-Pasta-Ulam U(x) = n (x n x n ) + α 3 (x n x n ) 3 (.3) n + β (x n x n ) 4 4 n + H = n ẋ n + n y m H = m ẏ m + m (x n x n ) + α 3 ω my m + α 3 (x n x n ) 3 (.4) n A k,l,m y k y l y m (.5) k,l,m ÿ m = ωmy m α A k,l,m y k y l k,l } {{ } (.6)

18 Fermi-Pasta-Ulam α H m A mω m (.7).4 Fermi-Pasta-Ulam FPU.5 FPU

19 Fermi-Pasta-Ulam 3.5. ( ) KAM n i ω i 0 (.8) i N = n FPU FPU.5. (solitary wave) (soliton)

20 Fermi-Pasta-Ulam 4 t R =0.44 N 3/ α B t L (.9) B : α : t L = N κ/m =N : (.0) KdV FPU 3 KdV 967 U(x) = N [ e b(x n x n ) + b(x n x n ) ] (.) n= N n= [+ ] b (x n x n ) b3 6 (x n x n ) 3 +

21 A A A A = A + A (3.) A N ( ) δa = (A A ) N (3.) δa A N (3.3) P (A)

22 3 6 Γ =(r, r,, r N, p, p,, p N ) (3.4) f(γ) A = = = dγa(γ)f(γ) daa dγδ(a(γ) A)f(Γ) } {{ } A(Γ) A daap (A) (3.5) A(Γ) =A (E, V, N) (,, ) (3.6) f(γ) =cδ(h(γ) E) (3.7) W = δ(h(γ) E)dΓ (3.8) H(Γ) = i p i m i +Φ(r,, r N ) (3.9)

23 3 7 W (E,V,N) k S(E,V,N) } {{ } = k ln W (E,V,N) (3.0) H(Γ) =E H(Γ) =E 3.. (T,V,N) T F H(Γ) f(γ) =Ce kt (3.) H(Γ) Z = e kt dγ (3.) F = kt ln Z (3.3) f(γ)

24 : Ising 944 Onsager H = J i<j S iz S jz µ B H i A iz (3.4) XY 96 H = J i<j S i S j, S =(S x,s y, 0) (3.5) 6-vertex 967 Lieb 8-vertex 97? Baxter vertex

25 Bethe (Molecular Dynamics (MD)) (E,V,N) 3.4. Monte Carlo method)(mc) i E i E j

26 3 0 E j <E i i + E j E j >E i e kt (E j E i ) 0 ξ ξ e kt (E j E i ) >ξ E i+ = E i e kt (E j E i ) <ξ E i+ = E j. i j j i T ji P i = T ji e E i kt. = Tij e E j kt = Tij P j (3.6) = = 5 N N

27 3 ξ. MD MC E = H C V = (H H ) NkT (3.7) δa(t)δa(j + τ) dτ (3.8) 00

28 3 3. LJ ( (σ ) ( σ ) ) 6 φ(r) =4ɛ r r (3.9) LJ

29 3 4 MD Newton m i d r i dt = F i = Φ r i (4.) 4.. L = K Φ= i m i ṙ i Φ(r, r, ) (4.) S = Ldt = L(ṙ, r, t)dt (4.3) δs =(S + δs) S = δldt ( ) L L = δr + r ṙ δṙ dt ( L = r d ) L δrdt = 0 (4.4) dt ṙ

30 4 4 d L dt ṙ = L r (4.5) 4..3 r i q i Legendre p i = L q i, (p i = m i q i ) (4.6) H(q, p) = i p i q i L(q, q) = i p i pi m i i p i m i +Φ(q) = i p i m i +Φ(q) =E ( ) (4.7) dq i dt = H p i dp i dt = H q i Newton dq i dt = p i m i dp i dt = Φ (4.8) q i m i d q i dt = Φ q i

31 Γ H q q.. q N H H Γ =, p Γ = q N H p.. p N H p N (4.9) dγ dt = } {{ } =J H Γ (4.0) ( ) t ( ) t dh H dt = dγ H Γ dt = J H Γ Γ = ( H q, H p ) ( ) 0 H q = 0 H p ( H q, H ) H p p H q = 0 (4.) 4.3. (q 0, p 0 ) (q(t), p(t)) (q(t), p(t)) t (q 0, p 0 ) (4.)

32 Liouville f(γ) f t + ( ) Γ Γf = 0 (4.3) df dt = f t + Γ f Γ (4.4) f t + Γ f ( ) Γ + Γ Γ f = 0 (4.5) ( ) df dt = Γ Γ f (4.6) Γ Γ = i = i ( df dt = Γ Γ ( qi + ṗ ) i q i p i ( H H ) =0 q i p i p i q i ) f = 0 (4.7)

33 t = t 0 r i (t 0 ), v i (t 0 ) r i (t), v i (t) m d r i dt = F i (5.) y =(y (t),y (t), ) (5.) dy dt = f(y,t) (5.3) dr i dt = p i m, dp i dt = F i t t t n = n t t n = n t y n = y(t n ) (5.4)

34 5 8 y n+ = G(y n+, y n, y n, ) (5.5) G y n+ (explicit) G y n+ (implicit) y n+ = G(y n+, y n ), y n (5.6) y n+ = G(y n+, y n,, y } {{ n k+ ), } k (5.7) k t n y n, y n, y n, (5.8) 5. y(t) y n O(( t) k ) (5.9) k CPU 5.3 self-start

35 5 9 ( t) k Euler Rnge-Kutta 4 Verlet 5 Gear (truncation error) (round-off error) 3 t 5.5 λ>0 λ<0 dx dt = λx (5.0)

36 5 30 dx dt = f(x,t) (5.) x 0 (t) x(t) =x 0 (t)+δx(t) } {{ } (5.) dx dt = dx 0 dt + d(δx) dt = f(x 0 (t)+δx(t),t)=f(x 0,t)+ f δx + (5.3) x 0 d(δ x) dt = f x δx + (5.4) x=x 0 δx f x 0 dδx i dt = λ i δx i (5.5) dx dt = λx (5.6) λ>0 λ <0 dx x = λdt ln x = λt + c x = Ceλt (5.7)

37 Euler x(t + t) =x(t)+ẋ(t) t +ẍ(t) ( t)! + (5.8) ẋ x(t + t) x(t) ẋ(t) = t dx x(t + t) x(t) = f(x, t) dt t = f(x(t),t) Euler x(t + t) =x(t)+ tf(x(t),t) (5.9) x n+ = x n + tf(x n,t) (5.0) x n+ = x n + tλx n =(+λ t)x n x n =(+λ t) n x 0 (5.) t = n t t 0

38 5 3 Euler ok ɛ n =(+λ t) n x 0 e λt x 0 =(+λ t) t/ t x 0 e λt x 0 +λ t =+λ t +! (λ t) +! (λ t) = e λ t! (λ t) ( ( + λ t) t/ t = e λ t! (λ t) ) t/ t = e ( λt ) t/ t! (λ t) e λ t [ ( ] ɛ n = e λt x 0 t/ t! (λ t) e ) λ t [ = e λt x 0 ]! (λ t) e λ t t t + = e λt x 0 ( ) λ te λ t + (5.) t 0 ɛ n 0 dx dt = f(x, t) (5.3) f(x, t) f(y, t) <L x y (5.4) L t 0 f ok f

39 5 33 ɛ n+ = x n+ x(t + t) ɛ n = x n x(t) ɛ n+ = ɛ n +(x n+ x n ) (x(t + t) x(t)) t+ t ( ) dx dx = ɛ n + λ tx n dt dt, = λx dt = ɛ n + λ tx n λ = ɛ n + λ t 0 t t+ t t x(t )dt ( xn x(t + t ) ) dt x(t + t )=x(t)+x (t)t + x (t)t + = ɛ n + λɛ n t λx (t) ( t) + =(+λ t)ɛ n + O ( ( t) ) (5.5) ɛ n+ ( + λ t)ɛ n (5.6) +λ t > +λ t < dx dt = f(x,t) (5.7) ɛ n+ = + t f x 0 λ i λ i < ( + t f ) ɛ n + O( ( ( t) ) (5.8) x 0 Euler + tλ <

40 5 34 x n x(t) x(t) t (5.9) Euler ( + λ t) t/ t e λt e λt ( + λ t) t/ t ok = e λt ( + λ t) t/ t e λt = e tλ t+ t 3 λ3 ( t) (5.30) +λ t < e λ t λ t = x + iy ( + x) + y <e x (5.3) e (λ ɛ)t < ( + λ t) t/ t < e λt e ɛt (5.3) 5.6. Euler dq dt = p, dp dt = q q n+ q n p n+ p n = p n, = q n t t ( ) ( )( ) qn+ t qn = p n+ t p n ( ) ( )n ( ) qn+ t q0 = p n+ t p 0 = ( ( +( t) ) n/ cos nθ sin nθ sin nθ cos nθ )( q0 p 0 ) (5.33)

41 5 35 tan θ = t θ = t 3 ( t)3 + 5 ( t)5 ( ) λ t det =0 t λ ( λ) +( t) =0 λ =± i t = +( t) e ±iθ (5.34) t + t x(t) =x(t + t t) = x(t + t) x t+ t t + x(t + t) =x(t)+x (t + t) t = x(t)+f(t + t, t + t) t dx dt = λx x n+ = x n + f(x n+,t+ t) t, (5.35) x n+ = x n + λx n+ t x n+ = λ t < λ t x n = ( λ t) n x 0 (5.36)

42 dq dt = p, dp dq q n+ q n t = p n, q n+ = q n + p n t p n+ p n t = q (5.37) = q n+ p n+ = p n q n+ t = p n (q n + p n t) t = p n q n t p n ( t) ( ) ( qn+ t = p n+ t ( t) ( ) t det =0 t ( t) )( qn p n ( λ) ( t) ( λ)+( t) =0 λ ( ( t) ) λ +=0 λ = ( ) ( t) ± i t ( t) 4 ( ) cos θ = ( t) θ, sin = t λ = cos θ ± i sin θ = e ±iθ, λ = (5.38) A = q n + tq n p n + ( ( t) ) p n = q 0 + tq 0 p 0 + ( ( t) ) p 0 (5.39) )

43 ( dx dt x n+ x n t x ( t) dx dt x n+ x n t 6 x ( t) x n+ x n t = λx n x 0,x x 0,x 0 x n+ = x n +λ tx n (5.40) x n+ λ tx n x n = 0 (5.4) x n = α n α λ tα =0 α ± = λ t ± +(λ t) x n = Aα+ n + Bα n (5.4) α ± < α + α = α +,α

44 Runge-Kutta 5.7. Runge-Kutta dy dx Runge-Kutta p k i k i = xf(x n,y n ) = f(x, y) (5.43) k j = xf(x n + ν j t, y n + µ j k j ) y n+ = y n + α k + α k + + α p k p (5.44) α,,α p,ν,,ν p,µ,,µ p f ( t) k p k p = k =4 Runge-Kutta 5.7. Runge-Kutta p =4 α = α 4 = 6, α = α 3 = 3 ν = ν 3 =, ν 4 =, µ = µ 3 =, µ 4 = (5.45)

45 5 39 Runge-Kutta k = xf(x n,y n ) ( k = xf x n + x, y n + ) k ( k 3 = xf x n + x, y n + ) k k 4 = xf(x n + x, y n + k 3 ) y n+ = y n + 6 (k +k +k 3 + k 4 ) (5.46) f(x, y) y Runge-Kutta = Runge-Kutta y n+ = y n + α xf(x n,y n ) +α } {{ } xf(x n + ν x, y n + µ k ) } {{ } =k =k dy = f(x, y) dx d y dx = f x + f y d 3 y dx = d 3 dy dx = f x + f y f dx 8f x + f y f)=f xx + f xy f + f yy f + fy f ( = y n + α xf(x n,y n )+α x f(x n,y n )+f x ν x + f y µ k + f xx(ν x) + f xy ν xµ k + ) f yy(µ k ) + + ( = y n +(α + α ) xf +( x) α fx ν + f y µ f ) + ( x)3 α ( fxx ν +f xy ν µ f + f yy µ f ) + (5.47)

46 5 40 y n+ = y n + xf + ( x) (f x + f y f) + ( x)3 (f xx + f xy f + f yy f + fy f)+ (5.48) 6 α + α =, α ν =, α µ = (5.49) ( x) α α = Runge-Kutta α = α, ν = µ = α (5.50) y n+ = y n + x[ f(x n,y n )+f(x n + x, y n + xf(x n,y n )) ] (5.5) α = ( y n+ = y n + xf x n + x, y n + ) xf(x n,y n ) (5.5) Runge-Kutta dy = y Runge-Kutta x =0,y= dx y = e x

47 5 4 k = x = x ( k = xf x n + x, y n + ) ( k = x + x ) k 3 = x (+ ( x + x )) k 4 = x( + k 3 )= x (+ x + ( x) + 4 ) ( x)3 (5.53) y = y (k +k +k 3 + k 4 ) =+ [ ( x + x + x ) 6 + x (+ x + ( x) ( + x + ( x)3 4 + x ) + ( x) 4 )] =+ x + ( x) + 6 ( x)3 + 4 ( x)4 (5.54) y n+ = y n ( + x +! ( x) + 3! ( x)3 + 4! ( x)4 ) (5.55) + x +! ( x) + 3! ( x)3 + 4! ( x)4 < (5.56) R-K Runge-Kutta Runge-Kutta ( x) 5 (local error) x = n x ( x) 4 (global error)

48 Runge-Kutta self-start Runge-Kutta MD 5.8 (Predictor-Corrector Gear MD Nordsieck Nordsieck, Math. Comp.6, (96) Gear Gear: Numerical Initial Value Problems in Ordinary Differential Equations, Prentice Hall (97) 5.8. dx dt = f(x, t) x(t),x (t),,x (k) (t)

49 5 43 X P (t + t) =P X(t) (5.57) x(t)! x() ( t) X(t) =! x() ( t), P = k! x(k) ( t) k P P ij = j C i, (j i), P ij =0(j<i) x P () (t + t) f ( x P (t + t),t+ t ) (5.58) δ = t [ f ( x P (t + t),t+ t ) x P () (t + t) ] X(t + t) =X P (t + t)+δc (5.59) C 0 C k C C =. (5.60) C 0 C C C 3 C 4 C

50 C 0 C C C 3 C 4 C 5 C X n = x(n t), Y n = tx (n t), Z n = ( t) x (n t) (5.6) X n+ = X n + Y n + Z n + Y n+ = Y n +Z n + C δ C 0 {}}{ C δ Z n+ = Z n + C 3 δ δ = tf(x n + Y n + Z } {{ n,t+ t) (Y } n +Z n ) } {{ } x P (t+ t) = tx P (t+ t) (5.6) C =.0 p = t δx n+ = δx n + δy n + δz n + C (pδx n+ δy n δz n ) (5.63) f X n+ δy n+ = δy n +δz n + C }{{} (pδx n+ δy n δz n )=pδx n+ ( pδx n ) = δz n+ = δz n + C 3 (pδx n+ δy n δz n ) (5.64) δy n = pδx n δy n ( ) ( ) δxn+ δxn = A δz n+ δz n ( + p C A = p C 3 p C p + C (p ) C p + C 3(p ) C p ) (5.65)

51 5 45 e p = exp ( t f x) λi λ i < p =0 λ = ( + p C det(a λe) = det λ + ) C (p ) p C p C 3 p C + C 3(p ) λ =0 p C p ( C p)( λ) +( λ) ( p + C 3 (p ) ) pc 3 = 0 (5.66) λ =+a p + a p + ( =+p + p + p C + C 3 3 ) p 3 6 C 3 + (5.67) p =0 λ =0 p =0 ( λ) C 3 ( λ) =( λ)( λ C 3 )=0 λ =, λ = C 3 =0 C 3 = (5.68) p =0 λ = e P C 3C + C 3 3 =0 3C = = 5 4, C = 5 λ =+p + p + p3 6 + O(p4 ) λ = p 7 44 p p3 + O(p 4 ) (5.69) p =0 0 C 3,C 4, C e P C 4,C 5, C,C

52 dx dt = x t =0,x= x = et,x = e t,x = e t X 0 = t ( t) X P = t = ( t) δ = t ( f(x P (t + t)) x P () (t + t) ) + t + ( t) t( + t) ( t) [ = t + t + ] ( t) ( + t) = ( t)3 5 + t + X = X P + δ = ( t) ( t)3 t ( + t + ( t)) ( ( t) + t) + ( t)+ ( t) + ( 33 3 X = t ( ( t) ( t)+ ( t) x 3 = + (3 t)+ (3 t) }{{} + 4n 3 ( t) ( t) ( t) 3 3 ( t) ( t) ) + 6 ) (3 t) 3 + (5.70) 6 ( t) 3 Gear t = n t ( t) y (p) = f ( y, y (),,y (p q),t ) (5.7) p q (p q) k

53 5 47 y ( t) k+q p p =,q=, ( t) k

54 q n+ = q n + p n t p n+ = p n q n+ t (6.) 6.. Strömer MD Verlet q(t + t) =q(t)+ q t +! q( t) + 3! q(3) ( t) 3 + 4! q(4) ( t) 4 + q(t t) =q(t) q t +! q( t) 3! q(3) ( t) 3 + 4! q(4) ( t) 4 + q(t + t)+q(t t) =q(t)+ q(t)( t) + q(4) ( t) 4 + (δt) q(t) = q(t + t) q(t)+q(t t) ( t) = F m

55 6 49 q(t), q(t t) t + t t q(t + t) =q(t) q(t t)+ F (q(t),t) ( t) (6.) m v(t) = = q(t + t) q(t t) t q(t) q(t + t) + F (q(t),t) t (6.3) t m t =0 q(0), v(0) t q( t) =q(0) v(0) t + F (q(0), 0) ( t) m (6.4) 6..3 Verlet leap frog dq dt = p m, dp dt = F (6.5) ( p t + t ) ( = p t t ) + F ( q(t) ) t q(t + t) =q(t)+ p ( ) t + t t (6.6) m q(t + t) =q(t)+ p ( ) t + t t m q(t) =q(t t)+ p ( ) t t t m

56 6 50 q(t + t) =q(t) q(t t)+ p ( t + t ) ( ) p t t t m =q(t) q(t t)+ F (q(t),t) ( t) (6.7) m v(t) = p ( t + t ) ( ) + p t t m (6.8) 6..4 Verlet ( ) q(t + t) =q(t)+v(t) t + F ( q(t) ) ( t) ( m v(t + t) =v(t)+ t ( ) F q(t) m + F ( )) q(t + t) m ( ) ( ) q(t) q(t + t) v(t) v(t + t) (6.9) 6..5 (Symplectic) dq i dt = H p i, dp i dt = H q i (6.0)

57 6 5 Z(p, q) dz dt = ( Z ṗ p i + Z ) q i i q i i = ( Z H Z ) H p i i p i q i q i {Z, H} ( ) [ ( H = H ) ] Z = D H Z (6.) p i i p i q i q i } {{ } D H dz dt = D HZ, d Z dt = D H(D H Z)=D HZ, Z(t) =e DHt Z(0) = Z(0) + Z (0)t +! Z (0)t + (6.) e DHt H t { t } Z(t) = exp D H (t )dt Z(0) (6.3) 0 Z(t + t) =e DH t Z(t) (6.4) e DH t 6.. e D Ht e D a t e D a t e D ka k t (6.5)

58 6 5 H(p, q) =T (p)+v (q) D H = D T + D V (6.6) e D H t = e (D T +D V ) t e D T t e D V t (6.8) (6.9) (6.7) A, B, C (6.7) (6.8) e D T t e D V t e D T t (6.9) e A e B = e C (6.0) C C = A + B + {[ ] [ ]} [A, B]+ A, [A, B] + [A, B],B + (6.) A, B [A, B] = AB BA Baker-Campbell- Hausdorff 6..3 (6.8) e (D T +D V ) t e D T t e D V t D V = ( V V ) = p i i q i q i p i i D T = ( T T ) = T p i i q i q i p i p i i V q i e D V t D V p i = V q i,d V p i = 0 p i = e D V t p i = ( +D V t + D V ( t) + p i (6.) q i (6.3) ) p i = p i V q i t (6.4)

59 6 53 e D T t q i = e D V t q i = q i, (D V q i = 0) (6.5) p i = e D T t p i = p i, (D T p i = 0) (6.6) q i = e D T t q i = q i + T t (6.7) p i ( D T p i = T ), D p T q i = 0 i p i q i p i (t + t) =p i (t) V q t, (6.8) i t q i (t + t) =q i (t)+ T q t, (6.9) i t+ t e D V t e D T t e D T t e D V t = e D V t e D T t (6.30) Z(p, q )=e D V t Z(p, q) Z(p, q) =e D V t Z(p, q ) (6.3) A(p, q )=e D V t A(p, q)e D V t (6.3) A(p, q )f(p, q )=e D V t A(p, q)e D V t f(p, q ) = e D V t ( A(p, q)f(p, q) ) = A(p, q )f(p, q ) (6.33) A(p, q )e D V t = ( e D V t A(p, q)e D V t ) e D V t = e D V t A(p, q) (6.34) A(p, q) =e D T t

60 (6.8) e D T t e D V t = e D V t e D T t = e D H t (6.35) H H D H = D V + D T + [D V,D T ] t + ( t) {[ D V, [D V,D T ] ] + [ D T, [D T,D V ] ]} + (6.36) [D A,D B ]=D {B,A} = D {A,B} (6.37) H = V + T t{v,t} + ({ ( t) V,{V,T} } + { {V,Y },T }) + = H t V T + ( V T V q i i p i ( t) + T ) V T + q i,j i p i p j q j p i q i q j p j = H tv qt p + ( t)( V q T pp V q + T p V qq T p ) + (6.38) H, H 6..5 Verlet Verlet p n+ = p n + F n t q n+ = q n + m p n+ t p n = (p n+ + p n ) t = p n + F n t = p n F n q n+ = q n + p n m t + F n m p n+ p n ( t)

61 6 55 e D V t,e D T t,e D t V. e D V t q = q n p = p n+ = p n + F n t (6.39). e D T t q = q n+ = q n + m p n+ t = q + p m t p = p (6.40) 3. e D t V q = q = q n + p n m t + F n ( t) m p = p n+ = p + F (q ) t = p t n+ + F n+ (6.4) Verlet e D H t e D V t e D T t e D V t (6.4) e D V t e D T t e D V t e D T t e D V t t e D V e D T t e D V t t e D V = e D V t e D T t e D V t = e D H t (6.43) H = H ++ 4 ( t)( T p V qq T p V q T pp V q ) + O ( ( t) 4 ) (6.44) 6..6 S ( t) =e D V t e D T t e D V t (6.45)

62 6 56 S 4 ( t) =S (d t)s (d t)s (d t) (6.46) d,d d = /3, d = /3 /3 (6.47) S 6 ( t) =S (d t)s (d t)s (d 3 t)s (d 4 t)s (d 3 t)s (d t)s (d t) (6.48) d,d,d 3,d

63 57 7 MD 7. MD (E,V,N) (extensive) A = A + A, A N (7.) (intensive) B = B E T V p N µ

64 7 MD MD MD Car-Parrinello 7.. MD ) Green-Kubo ) MD 3) 98 Evans et al. sllod 0 4) 98 Evans et al.

65 7 MD ) 97 Woodcoch ad hoc scaling ) Langevin 978 Schneider-Stoll 3) 980 Andersen T Boltzman 4) 98 ) 5) 984 6) 985 Hoover Nos e-hoover 7) 990 Bulgac-Kusnezov Nos e-hoover 8) 999 Bond et al. 5) Nos e-poincar e

66 7 MD ) 980 Andersen ) 980 Parrinello-Rahman 3) 990 Cleveland, Wentzcovich 7..4 ) 990 Çagin, MontGomery Pettitt fractional 7..5 Car-Parrinello ) 985

67 6 8 MD 8. N N N N 8. N N N = MD. (Constraint Method) K = K i m iv 3 i = NkT (8.). (Stochastic Method) a

68 8 MD 6 Langevin m i d q i dt = Φ dq mγ i i +R i (8.) q i } {{ dt} R i (t) α R j (t) β =m i γ i ktδ αβ δ ij δ(t t ) (8.3) b Anderson Bolzmann 3. (Extended system method) Andersen N N = 4. Berendsen et. al. J. Chem. Phys. 8, 3684(984) E

69 8 MD 63 p 8.3 (a) Woodcock, Chem. Phys. Lett. 0, 57 (97) MD ad hoc scaling leap frog algorithm v i ( t + t K ( t + t ) ( = v i t + t ) = i m i ( v i ) + F i(t) t (8.4) m ( t + t )) = 3 NkT (8.5) 3 NkT ( v i t + t ) ( = sv i t + t ) (8.6) K ( t + t ) = i s = m i T T = ( (v i t + t )) = s 3 NkT = 3 NkT (8.7) i m i 3 NkT ( ( )) v i t + t (8.8)

70 8 MD 64 (b) Gauss W. G. Hoover et. al. Phys. Rev. Lett. 48, 88 (98) D. J. Evans J. Chem. Phys. 78, 397 (983) K = p i i m i Gauss R(q, q,t) = 0 (8.9) R t + R R q + q = 0 q q (8.0) d q m i i dt = Φ + F ic (8.) q i F ic R = Φ R R m i q i q i q i t m i q i R (8.) q i F ic F ic R q i K = F ic R q i (8.3) R = m i q i 3 NkT 0 i (8.4) R = m i q q i = p i i (8.5)

71 8 MD 65 p i dq i dt = p i m i (8.6) dp i dt = Φ ζp q i i (8.7) Ṙ = i q i q i = 0 (8.8) (8.7) (8.8) q i ζ i ( Φ ) ζp q i = 0 (8.9) i ζ = i q i q Φ i g i p i m = dφ dt gkt (8.0) Γ =(q, p) f(p) f t + ( ) Γf = 0 (8.) Γ f t + Γ f ( ) Γ + Γ Γ f = 0 (8.) df dt = f t + Γ f Γ df dt = ( f q q i + f ) ṗ i i p i i ( ) [ ( = Γ Γ f = q q i + ) ] ṗ i p i f (8.3) i dq i dt = H, p i i ( q i H p i i dp i dt = H (8.4) q i ) H = 0 (8.5) p i q i

72 8 MD 66 Liouville df dt = 0 (8.6) dq i dt = p i m i, dp i dt = Φ q i ζp i (8.7) i ( q q i + ) ṗ i p i = i i df dt p i ( ζp i ) = 3Nζ i ζ p i p i } {{ } ζ = (3N )ζ (8.8) ) dφ =(3N )ζf = (3N gkt dt f (8.9) g =3N i f e 3N g f e Φ kt Φ(q) kt (8.30) p i = g 3N kt = kt (8.3) m i ( ) p i f(q, p) δ 3N Φ(q) kt e kt (8.3) m i i

73 8 MD S. Nosé Mol. Phys. 5,55 (984); J. Chem. Phys. 8,5,(984); Prog. Theor. Phys. Suppl. 03, (99) 8.4. p i H N = m i i s +Φ(q) } {{ } p H 0 ( s,q) + gkt ln s Q } {{ } + p s (8.33) ln s e H/kT p i = 3 NkT (8.34) m i i v = dq dt v = q q = q (8.35) t s t = t s }{{} t = t (8.36) s s t v = q t = d q t = sv, ( ) (8.37)

74 8 MD 68 H N q = q p = p s v = sv dt = dq i dt = H N = p i p i m i s (8.38) dp i dt = H N = Φ q i q i (8.39) ds dt = H N p s dp s dt = H N s = p s Q = s ( i p i m i s gkt ) dt s (8.40) (8.4) 8.4. H N Z = dpdqdsdp s δ ( i p i m i s +Φ(q)+ p s + gkt ln s E Q ) (8.4) p = p s, q = q dpdq = s 3N dp dq (8.43) (s, p s, p, q ) ( ) Z = ds dp s dp dq s 3N δ H 0 (p, q )+ p s + gkt ln s E Q E p s Q H 0(p, q ) s 0 = exp gkt (8.44) (8.45) δ(f(s)) = δ(s s 0) f (s )

75 8 MD 69 f(s) =0 s Z = dp s dp dq ds s 3N δ(s s 0 ) gkt = dp s dp s 0 dq gkt exp (3N +) ( E p s Q H 0(p, q ) gkt ) g =3N + = dp s ekt = dp s ekt ( ) E p s Q ( E p s Q dp ) H 0 (p, q ) dq e kt Z C (8.46) Z C (MC) ( p ) ( p ) ds dp s dp dqa A s, q s, q δ(h N E) = MC ds dp s dp dqδ(h N E) ( ) E p s H 0 (p, q ) dp s ekt Q dp dq A(p, q )e kt = dp s ekt ( ) E p s Q dp H 0 (p, q ) dq e kt = A(p, q ), (8.47) C ( p ) A s, q T ( ) p(t) = lim A T T s(t), q(t) dt (8.48) 0 ( p ) = A s, q A(p, q ) MC C (8.49)

76 8 MD 70 Q Q Q Nosé-Hoover H N = i p i m i s +Φ(q)+ p s + gkt ln s (8.50) Q dq i dt = dt s, q = q, p = p s (8.5) = s dq i dt dt = s H = p i p i m i s = bp i (8.5) m i dp i = s d ( pi ) = dp i dt dt s dt ds s dt p i = Φ ds p i q i dt s = Φ ds p q i }{{} s dt i (8.53) =ζ ζ = ds (8.54) s dt dζ = s d ( ) dt dt s sds = s d ( ) ps dt dt Q ( ) = s p i Q m i i s gkt 3 s ( ) = (p i) gkt (8.55) Q m i i

77 8 MD 7 Nosé-Hoover dq i dt = p i (8.56) m i dp i dt = Φ ζp q i (8.57) ( i ) dζ dt = (p i ) gkt (8.58) Q m i i dζ dt = gkt ( ) T (t) T0 Q (8.59) T (t) T 0 T (t) >T 0 dζ dt > 0 ζ ζ>0 (8.57) T (t) <T Γ=(p, q,ζ) f(γ) f t + Γ ( ) Γf = 0 (8.60) df dt = f t + Γ f ( ) Γ = Γ Γ f (8.6) Γ Γ = ṗ p i = ( ζp i i p i )= 3Nζ i i (8.6) df =3Nζf dt (8.63) H T = H 0 (p, q)+ Qζ (8.64)

78 8 MD 7 dh T = ( H0 q dt q i + H ) 0 ṗ i i p i + Qζ ζ i ( Φ pi + p i q i m i m i = i = gktζ ( Φ )) ( ) p i ζp q i + Qζ gkt i m i i Q (8.65) g =3N df dt = dh T gkt dt 3Nf = dh T kt dt f (8.66) g =3N lim T df dt = dh T kt f e H T kt dt f (8.67) H 0 (p, q) Qζ kt kt (8.68) = e T ( p ) A T 0 s, q dt = lim T = T T 0 T 0 ( p ) s A s, q s ( p ) dt A s, q s T s dt = A(p, q ) C (8.69) 8.5 Nos e-poincar e 8.5. Nos e-poincar e Nos e Nosé-Hoover

79 8 MD 73 Bulgac and Kusnezov, Phys. Rev. A4, 5045 (990) Nosé H N H N Nosé-Poincaré 8.5. Nos e-poincar e H N Nosé-Poincaré Bond et al. J. comp. phys. 5,4 (999) H NP = s [ H N H N (t =0) ] (8.70) dq i dt = H NP = s H N p i p i (8.7) dp i dt = H NP = s H N q i q i (8.7) ds dt = H NP = s H N p s p s (8.73) dp s dt = H NP = s H N s s [ H N H N (t =0) ] } {{ } (8.74) =0 dq i dt = H N dt = dt p i s 8.6 ζ, s Q Q K( )

80 8 MD 74 ζ, s 8.6. Q Q Q s = s ( i ) (p i ) m i s gkt (8.75) s = s + δs (8.76) ( Q d δs ( p i δs ) ) gkt dt s m i i s s ( ) p i = δs (8.77) m i s 4 i p i = gkt m i i s (8.78) Q d δs = gkt δs dt s (8.79) ω = gkt Q s (8.80) ω = gkt Q (8.8) 8.6. Q Q

81 8 MD 75 Q d δs = gk ( T (t) T ) s dt [ ] (T (t) T ) = gk δs = gkaδs (8.8) δs } {{ } ω = sgka Q = gk C V ω (8.83) A = T (t) T δs (δt) (δs) (δt) C = (δt) + (δt) MC (δt) = (δt) C (δt) MC = T g T ( gk ) = kt g C V C V (δs) = s C V g k kt A = g k = gkt (8.84) C V s C V s C V Di Tolla and Ronchetti, Phys. Rev. E48, 76 (993) s

Nosé Hoover 1.2 ( 1) (a) (b) 1:

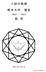
Nosé Hoover 1.2 ( 1) (a) (b) 1: 1 watanabe@cc.u-tokyo.ac.jp 1 1.1 Nosé Hoover 1. ( 1) (a) (b) 1: T ( f(p x, p y, p z ) exp p x + p y + p ) z (1) mk B T p x p y p = = z = 1 m m m k BT () k B T = 1.3 0.04 0.03 0.0 0.01 0-5 -4-3 - -1 0