0 2 SHIMURA Masato
|
|
|
- あきとし たかぎ
- 5 years ago
- Views:
Transcription
1 0 2 SHIMURA Masato jcd02773@nifty.com Worked Example Script Worked Example References 18 A Script 18
2 * h = x a f x) = f a) + x a 1! f a) + x a)2 2! f a) + x a)3 3! f a) + f x + h), f x h) f x) = f a) + h 1! f a) + h2 2! f a) + h3 3! f a) + f x + h) = f x) + f x)h + f x) h2 2 + f x) h3 6 *1 J r32 f x h) = f x) f x)h + f x) h2 2 f x) h3 6
3 h h 2 f x) = d f dx = 1 f x + h) f x)) h f x) = d f dx = 1 f x) f x h)) h f x) = d f dx = 1 f x + h) f x h)) 2h 2 f x) = d f dx = 1 f x + h) f x h)) 2h f x) = d2 f dx = 1 f x + h) 2 f x) + f x h)) 2 h2 1.2 [ ] [ ] Johan Peter Gustav Le jenue Dirichlet ) n=5) n 14
4 y = px)y + qx)y + rx), x [a, b] y a) = α, yb) = β 2 2 {y = px)y + qx)y + rx)} x = x i 1 i N 1) y i+1 2w i + y i 1 h 2 + Oh 2 ) = p i y i+1 y i 1 2h + q i y i + r i + Oh 2 ) p i, q i, r i y w w i+1 2w i + w i 1 h 2 = p i w i+1 w i 1 2h + q i w i + r i yx0) = α, yx N ) = β 2 y w 2 w i+1 2w i + w i 1 h 2 = p i w i+1 w i 1 2h w 0 = α + q i w i + r i, 1 i N 1 w N =β 2.2 x 0, x N ) x 1, x 2, x 3,, x N 1 x i = a + ih h = b a, h h Aw=b
5 h ) 2 p i w i h 2 q i )w i h ) 2 p i w i+1 = h 2 r i w 0, w 1, w 2 1 h ) 2 p 1 w h 2 q 1 )w h ) 2 p 1 w 2 = h 2 r i w 0 = α 2 + h 2 q 1 )w h ) 2 p 1 w 2 = h 2 r i h ) 2 p 1 α i = N 1 1 h ) 2 p N 1 w N h 2 q N 1 )w N 1 = h 2 r N h ) 2 p N 1 β w 1, w 2, w 3,, w N 1 Aw=b d 1 u 1 l 2 d 2 u 2 A = l 3 d 3 u 3 with l N 3 d N 3 u N 3 l N 2 d N 2 u N 2 l N 1 d N 1 d i = 2 + h 2 q i u i = 1 + h 2 p i
6 l i = 1 h 2 p i w 1 h 2 r h 2 p 1)α w 2 h 2 r 2 w = and b = w N 2 w N 1 h 2 r N 2 h 2 r N h 2 p N 1)β A, l i, d i, u i h p i, q i 2.3 Aw = b w 0 = α 1 h ) 2 p i w i h 2 q i )w i h ) 2 p i w i+1 = h 2 r i, 1 i N 1 A N + 1)N + 1) w N = β
7 1 0 d 1 u 1 l 2 d 2 u 2 A = l 3 d 3 u 3 l N 3 d N 3 u N 3 l N 2 d N 2 u N 2 l N 1 d N w 0 w 1 α h 2 r h 2 p 1)α w 2 h 2 r 2 w = and b = w N 2 h 2 r N 2 w N 1 w N h 2 r N h 2 p N 1)β β 2.4 Worked Example Demonstration Problem 2 Bradie Example 8.2 EXAMPLE. f ormula u = x + 1)u + 2u + 1 x 2 )e x boundary condition u0) = 1, u1) = 0 h 1 4. x i x i = i 4 1 h ) 2 p i w i h 2 q i )w i h ) 2 p i w i+1 = h 2 r i
8 . h h h h x 0 = 0 x 1 =.25 x 2 = 0.5 x 3 = 0.75 x 4 = Dirichlet boundary condition 1 unknown unknown unknown 0. p 1 = x i + 1) = 1 + i 4 ) q i = 2 r i = 1 x 2 i )e x i = 1 i 4 )2 )e i 4 i.y NB. Demonstration problem 2 NB. u =-x+1)u +2u+1-xˆ2)eˆ-x NB. h=1r4,n=4,u0,1)=-1,0 fp2=: 3 : ->: i. >: y) % y NB. p fq2=: 2: NB. q fr2=: 3 : 1 - y0ˆ2 )* ˆ - y0=. i. >: y) % y NB. r Script 1 0) fp2;fq2;fr2) calc_mat_2nddif 1r4;4;_1 0 i. # y N x 0, x 1,, x n, x n+1 0 Newman,Robin. Dirichlet 1-1,0
9 1 0 w r32 17r8 37r w r16 17r8 19r16 0 w 2 = r32 17r8 39r32 w w 4 0. x fp2;fq2;fr2) y h;n; ) ex. 1r4;4; 1 0 fp2;fq2;fr2) calc_mat_2nddif 1r4;4;_ _1 _1 _27r32 17r8 _37r _ _ _13r16 17r8 _19r16 0 _ _ _25r32 17r8 _39r32 _ _ plot h = 1r10 x = 0.1 ]a=.fp2;fq2;fr2) calc_mat_2nddif 1r10;10;_1 0 line,marker plot 0.1*>:i.11 );{:"1 ; {: a
10 Demonstration Problem 1 Bradie Example 8.1 EXAMPLE. f ormula u + π 2 u = 2π 2 sinπx) boundary condition u0) = u1) = 0 1 h 4. N x i = 1 4 px) = 0 qx) = π 2 rx) = 2π 2 sinπx) NB. Bradie P668 NB. Dirichlet boundary condition NB. Demonstration proberm 1 fp1=: 0: NB. p fq1=: 3 : 1p2*1 NB. * 1 is alternate of 1p2: NB. q fr1=: 3 : _2p2 * 1 o. 1p1 * i. >: y) % y NB. r 0: 0 1p2*1 1 1 o. sin.
11 clean L:0 fp1;fq1;fr1) calc_mat_2nddif 1r4;4; _ _ _ _ _ _ diff mat wi ans plot 2.5 Script 1 : 0) NB. Usage: fp2;fq2;fr2) calc_mat_deriv 1r4;4;_1 0 calc_mat_2nddif =: 1 : 0 u L : 0 3 fp2;fq2;fr2 f=: u L:0 IX l i, d i, u i w i 1, w i, w i+1
12 X0=:_1- -: H) * ;{. f X1=: 2+ Hˆ2) * ;1{f X2=: _1+ -: H) * ;{. f w i 1, w i, w i+1 XX=: IX adjust_length_sub X0;X1;X2 4 adjust_length_sub 1 ; 2; n."0 1 " _1 _2 _3."0 1 ] i M0=. }.}: : : XX), ;"1),.IX-2)#<IX#0 NB. drop topa1x) and lastanx) line MX=. 1, IX#0),M0,IX#0),1 NB. add top and last line M1=. 0,- i.<: IX),0)."0 1 MX NB. twisted Dirichlet MX=. 1, IX#0),M0,IX#0),1 y Y0=. -Hˆ2)* ;{:f NB. y YX=. {.U),}. }: Y0),{:U YX %. M1 M1;YX,. YX %. M1 NB. calc differential equation
13 3 Carl Neumann ) 50 C. Dirichlet u0, t) = ul, t) = 0 u Neumann u u 0, t) = l, t) = 0 x x u x u Robin x + hu = T Newman/Robin. h h h h x f x 0 = α x 1 x 2 x 3 Computa tional domain x0 = α : * 2 y a) = α y b) = β α 1 ya) + α 2 y a) = α 3 β 1 ya) + β 2 y a) = β 3 *2
14 y = px)y + qx)y + rx), x [a, b] computational template 1 h ) 2 p i w i h 2 q i )w i h ) 2 p i w i+1 = h 2 r i w F x = x 0 1 h ) 2 p 0 w f h 2 q 0 )w h ) 2 p 0 w 1 = h 2 r 0 α 1 ya) + α 2 y a) = α 3 = α 1 w 0 + α 2 w 1 w f 2h = α 3 w f x = α : w f = w 1 2h α 2 α 3 α 1 w 0 ) [ 2 + h 2 q hp 0 )h α ] 1 w α 0 2w 1 = h 2 r hp 0 )h α 3 2 α 2 α 1 = 0 x = b [ 2w N 1 + x = b 2 + h 2 q 0 )w 0 2w 1 = h 2 r hp 0 )hα 2 + h 2 q N +2 hp N )h β 1 β 2 ] w N = h 2 r N + 2 hp N )h β 3 β 2 2w N h 2 q N )w N = h 2 r N 2 hp N )hβ
15 Aw = b A N + 1)N + 1) A = a 11 a 12 l 1 d 1 u 1 l 2 d 2 u 2 l N 1 d N 1 u N 1 a N+1,N b 1 h 2 r 1 h 2 r 2 b = h 2 r N 1 b N+1 a N+1,N+1 d i = u 1 = l 1 = 2 + h 2 q i 1 + h 2 p i 1 h 2 p i 4 2 y = px)y + qx)y + rx), x [a, b] Dirichlet ya) = α yb) = β Neumann y a) = α y b) = β Robin α 1 ya) + α 2 y a) = α 3 β 1 yb) + β 2 y b) = β 3
16 A = a 11 a 12 l 1 d 1 u 1 l 2 d 2 u 2 l N 1 d N 1 u N 1 a N+1,N b 1 h 2 r 1 h 2 r 2 b = h 2 r N 1 b N+1 a N+1,N+1 d i = u 1 = l 1 = 2 + h 2 q i 1 + h 2 p i 1 h 2 p i 9 DD DN DR ND NN NR RD RN RR D = Dirichlrt, N = Newman, R = Robin a 11 a 12 a 11 = 1 Dirichlet BC x = a d 0 Neumann BC x = a d 0 + 2hl 0 α 1 /α 2 Robin BC x = a { 0 Dirichlet BC x = a a 12 = 2 othewise
17 a N+1,N+1 a N+1,N+1 = 1 Dirichlet BC x = b d N Neumann BC x = b d N 2hu N β 1 /β 2 Robin BC x = b a N+1,N { 0 Dirichlet BC x = b a N+1,N = 2 othewise b 1 b 1 = α Dirichlet BC x = a h 2 r 0 + 2hl 0 α Neumann BC x = a h 2 r 0 + 2hl 0 α 3 /α 2 Robin BC x = a b N+1 b N+1 = β Dirichlet BC x = b h 2 r N 2hu N β Neumann BC x = b h 2 r N 2hu N β 3 /β 2 Robin BC x = b 4.1 Worked Example Bradie Example 8.3 u + u = sin3x), x [0, π 2 ] Robin x = 0 u0) + u 0) = 1 Newman x = π 2 u π 2 ) = 1 unifurm partition [0, π 2 ] h = iπ 8, f or i = 0, 1, 2, 3, 4 p i = 0 q i = 1 r i = sin 3iπ 8 ) Robin x = 0, α 1 = α 2 = 1, α 3 = 1 Newman x = π 2, β = 1
18 d π π 4 2 w d 1 w 1 π 8 )2 sin 3π 8 ) 1 d 1 w 2 = π 8 )2 sin 6π 8 ) 1 d 1 w 3 π 8 )2 sin 9π 8 ) 2 d w 4 π 8 )2 + π 4 π ) 2 d = 2 8 fp3;fq3;fr3) calc_2nddiff_all 1r8p1;4; 1 1 _1 ; 1 0 0; RN _ _ _ _1 0 0 _ _ _ _1 0 _ _ _ _ _ y ANS) Example 8.1 OK) clean L:0 fp1;fq1;fr1) calc_2nddiff_all 1r4;4;0 0 0; 0 0 0; DD _ _
19 0 _ _ _ _ ANS 5 References Brain Bradie [A friendly Introduction to Numeric Analsis] Pearson 2006 A Script calc_mat_2nddif =: 1 : 0 NB. Usage: fp2;fq2;fr2) calc_mat_2nddif 1r4;4;_1 0 NB. another // mat_lp 1r10; 10;_1 0) H IX U =. y NB. h ; xi.n); u0,1) f=. u L:0 IX NB. add N+1 is done at fpx side X0=._1- -: H) * ;{. f X1=. 2+ Hˆ2) * ;1{f NB. if. 1=# X1 do. X1=: IX # X1 end. X2=. _1+ -: H) * ;{. f NB. below is expand singletonbecause error occure at 1,.1 2 3) XX=. IX adjust_length_sub X0;X1;X2 NB. - y Y0=. -Hˆ2)* ;{:f NB. y YX=. {.U),}. }: Y0),{:U NB. except topy0) and lasyyn)line and append Dirichlet boundary condition NB. ----make extended diff box M0=. }.}: : : XX), ;"1),.IX-2)#<IX#0 NB. drop topa1x) and lastanx) line MX=. 1, IX#0),M0,IX#0),1 NB. add top and last line M1=. 0,- i.<: IX),0)."0 1 MX NB. twisted NB. ---calc matrix M1;YX,. YX %. M1 NB. calc differential equation ) adjust_length_sub=: 4 : 0
20 NB. expand to same length NB. alternative expand_box NB. y is X0;X1;X2 NB.x is IX LEN=. ; # L:0 y INDEX=. +/ INDEX0=. LEN e. >: x select. INDEX case. 3 do. XX=. : ;"1),. y NB. all vector case. 0 do. XX=. : ;"1),. >:x) # L:0 y NB. all scaller fcase. do. TMP=. >: x) # L:0 -. INDEX0) # y NB. expand scallor TMP=.INDEX0 # y), TMP NB. marge IND=. indt_sub INDEX0) i NB. expand index XX=. : ;"1),. IND { TMP NB. back to origin order end. XX ) NB. find_index indt_sub=: 3 : I. y),i.-.y NB Newman/Robin boundary condition NB. Bradie Example 8.3 fp3=: 0: fq3=: _1 fr3=: 3 : 1 o. 3r8p1 * i. >: y NB. sin NB. alpha1=alpha2=1, alpha3=_1 NB. beta=1 calc_2nddiff_all =: 1 : 0 NB. clean L:0 fp1;fq1;fr1) cnr 1r4;4;0 0 0; 0 0 0; DD OK NB. fp3;fq3;fr3) cnr 1r8p1;4; 1 1 _1 ; 1 0 0; RN H IX ALPHA BETA TYPE =: y NB. h ; xi.n); 6 disimalalpha beta 1 2 3)
21 NB. TYPE is DD, DN, DR... RN, RR NB. 9type f=: u L:0 IX NB. x X0=:_1- -: H) * ;{. f NB. Li X1=: 2+ Hˆ2) * ;1{f NB. Di X2=: _1+ -: H) * ;{. f NB. Ui NB. below is expand singletonbecause error occure at 1,.1 2 3) XX=: IX adjust_length_sub X0;X1;X2 NB. - calc x and y Y0=: -Hˆ2) * ;{: f NB. {: f is r //-hˆ2*r NB. -hˆ2* r0 <----- body of y NB. over item is last BX0=: {. Y0) + +: H * {. X0 NB. -2hˆ2 r0) + 2h* l_0 <-- top b1 BXN=: {: Y0) - +: H * {: X2 NB. -2hˆ2*r_n) - 2h* u_n <--bn NB NB. ----matrix-calc a11 a12 and b NB. A11=. A12=. ANL=. ANR=. B1=. BN=. 0 NB. reset IND0=: I. DNR e. {. TYPE NB. top line of a,b NB. Newman if. 0= ;IND0 do. NB. Dirichlet A11=. 1 A12=. 0 B1=. {. ALPHA end. if. 1 = ; IND0 do. A11=. {. X1 NB. keypoint = d0 A12=. _2 NB. neighbore B1=. BX0 * {. ALPHA end. NB. alpha NB. Robin if. 2 = ; IND0 do. A11=. {.X1) + +: H * X0 * %/ 2{. ALPHA NB. alpha1/alpha2 A12=. _2 B1=. BX0 * %/ 2 1 { ALPHA NB. alpha3/alpha2 NB. Dirichlet end. NB. ----matrix-calc an-1 an2 and bn IND1=: I. DNR e. {: TYPE NB. last line of a,b
22 NB. Newman if. 0= ; IND1 do. NB. Dirichlet ANR=. 1 ANL=. 0 BN=. {. BETA end. if. 1 = ; IND1 do. ANR=. {: X1 ANL=. _2 BN=. BXN * {. BETA end. NB. Robin if. 2=;IND1 do. ANR=. {: X1) - +: H * {: X2) * {. BETA) % 1{. BETA NB. beta1/beta2 ANL=. _2 BN=. BXN * %/ 2 1 { BETA NB. beta 3/beta2 end. NB YX=: B1,}.}:Y0),BN NB. drop last// NB. y main NB. ----make extended diff box M0=:}. }: : : XX), ;"1),.IX-2)#<IX#0 NB. except top and last line NB. MX=: A1,_2,<:IX)#0),M0,<:IX)#0), _2,AN MX=:A11,A12,<:IX)#0), M0, <:IX)#0),ANL,ANR RIND=: 0,-&i. <: IX),0) NB. rotate index M1=: RIND."0 1) MX NB. twist except top&last NB. --main = calc matrix M1 ; YX,.YX %. M1 NB. main = calc differential equation )
: 1g99p038-8
 16 17 : 1g99p038-8 1 3 1.1....................................... 4 1................................... 5 1.3.................................. 5 6.1..................................... 7....................................
16 17 : 1g99p038-8 1 3 1.1....................................... 4 1................................... 5 1.3.................................. 5 6.1..................................... 7....................................
1 1.1 p(x n+1 x n, x n 1, x n 2, ) = p(x n+1 x n ) (x n ) (x n+1 ) * (I Q) 1 ( 1 Q 1 Q n 0(n ) I + Q + Q 2 + = (I Q) ] q q +/. * q
![1 1.1 p(x n+1 x n, x n 1, x n 2, ) = p(x n+1 x n ) (x n ) (x n+1 ) * (I Q) 1 ( 1 Q 1 Q n 0(n ) I + Q + Q 2 + = (I Q) ] q q +/. * q 1 1.1 p(x n+1 x n, x n 1, x n 2, ) = p(x n+1 x n ) (x n ) (x n+1 ) * (I Q) 1 ( 1 Q 1 Q n 0(n ) I + Q + Q 2 + = (I Q) ] q q +/. * q](/thumbs/93/113048245.jpg) Masato Shimura JCD02773@nifty.ne.jp 2008 7 23 1 2 1.1....................................... 2 1.2..................................... 2 2 3 2.1 Example...................................... 3 2.2 Script...........................................
Masato Shimura JCD02773@nifty.ne.jp 2008 7 23 1 2 1.1....................................... 2 1.2..................................... 2 2 3 2.1 Example...................................... 3 2.2 Script...........................................
春期講座 ~ 極限 1 1, 1 2, 1 3, 1 4,, 1 n, n n {a n } n a n α {a n } α {a n } α lim n an = α n a n α α {a n } {a n } {a n } 1. a n = 2 n {a n } 2, 4, 8, 16,
 春期講座 ~ 極限 1 1, 1 2, 1 3, 1 4,, 1 n, n n {a n } n a n α {a n } α {a n } α lim an = α n a n α α {a n } {a n } {a n } 1. a n = 2 n {a n } 2, 4, 8, 16, 32, n a n {a n } {a n } 2. a n = 10n + 1 {a n } lim an
春期講座 ~ 極限 1 1, 1 2, 1 3, 1 4,, 1 n, n n {a n } n a n α {a n } α {a n } α lim an = α n a n α α {a n } {a n } {a n } 1. a n = 2 n {a n } 2, 4, 8, 16, 32, n a n {a n } {a n } 2. a n = 10n + 1 {a n } lim an
1 SHIMURA Masato polynomial irr.xirr EXCEL irr
 1 SHIMURA Masato 2009 12 8 1 2 1.1................................... 2 1.2 polynomial......................... 4 2 irr.xirr EXCEL 5 2.1 irr............................................. 5 2.2 d f, pv...........................................
1 SHIMURA Masato 2009 12 8 1 2 1.1................................... 2 1.2 polynomial......................... 4 2 irr.xirr EXCEL 5 2.1 irr............................................. 5 2.2 d f, pv...........................................
dy = sin cos y cos () y () 1 y = sin 1 + c 1 e sin (3) y() () y() y( 0 ) = y 0 y 1 1. (1) d (1) y = f(, y) (4) i y y i+1 y i+1 = y( i + ) = y i
 007 8 8 4 1 1.1 ( ) (partial differential equation) (ordinary differential equation) 1 dy = f(, y) (1) 1 1 y() (1) y() (, y) 1 dy = sin cos y cos () y () 1 y = sin 1 + c 1 e sin (3) 1 1 5 y() () y() y(
007 8 8 4 1 1.1 ( ) (partial differential equation) (ordinary differential equation) 1 dy = f(, y) (1) 1 1 y() (1) y() (, y) 1 dy = sin cos y cos () y () 1 y = sin 1 + c 1 e sin (3) 1 1 5 y() () y() y(
1 4 1 ( ) ( ) ( ) ( ) () 1 4 2
 7 1995, 2017 7 21 1 2 2 3 3 4 4 6 (1).................................... 6 (2)..................................... 6 (3) t................. 9 5 11 (1)......................................... 11 (2)
7 1995, 2017 7 21 1 2 2 3 3 4 4 6 (1).................................... 6 (2)..................................... 6 (3) t................. 9 5 11 (1)......................................... 11 (2)
ax 2 + bx + c = n 8 (n ) a n x n + a n 1 x n a 1 x + a 0 = 0 ( a n, a n 1,, a 1, a 0 a n 0) n n ( ) ( ) ax 3 + bx 2 + cx + d = 0 4
 20 20.0 ( ) 8 y = ax 2 + bx + c 443 ax 2 + bx + c = 0 20.1 20.1.1 n 8 (n ) a n x n + a n 1 x n 1 + + a 1 x + a 0 = 0 ( a n, a n 1,, a 1, a 0 a n 0) n n ( ) ( ) ax 3 + bx 2 + cx + d = 0 444 ( a, b, c, d
20 20.0 ( ) 8 y = ax 2 + bx + c 443 ax 2 + bx + c = 0 20.1 20.1.1 n 8 (n ) a n x n + a n 1 x n 1 + + a 1 x + a 0 = 0 ( a n, a n 1,, a 1, a 0 a n 0) n n ( ) ( ) ax 3 + bx 2 + cx + d = 0 444 ( a, b, c, d
 ATM M.Shimura JCD02773@nifty.ne.jp 2003 12 13 JAPLA2003 1 queue ATM ATM queue 1.1 ATM No (Sec (Sec 1 13 37 60 26 28 99 1 25 40 39 143 202 14 88 190 27 1 184 2 170 37 40 130 317 15 121 72 28 48 115 3 101
ATM M.Shimura JCD02773@nifty.ne.jp 2003 12 13 JAPLA2003 1 queue ATM ATM queue 1.1 ATM No (Sec (Sec 1 13 37 60 26 28 99 1 25 40 39 143 202 14 88 190 27 1 184 2 170 37 40 130 317 15 121 72 28 48 115 3 101
70 : 20 : A B (20 ) (30 ) 50 1
 70 : 0 : A B (0 ) (30 ) 50 1 1 4 1.1................................................ 5 1. A............................................... 6 1.3 B............................................... 7 8.1 A...............................................
70 : 0 : A B (0 ) (30 ) 50 1 1 4 1.1................................................ 5 1. A............................................... 6 1.3 B............................................... 7 8.1 A...............................................
,. Black-Scholes u t t, x c u 0 t, x x u t t, x c u t, x x u t t, x + σ x u t, x + rx ut, x rux, t 0 x x,,.,. Step 3, 7,,, Step 6., Step 4,. Step 5,,.
 9 α ν β Ξ ξ Γ γ o δ Π π ε ρ ζ Σ σ η τ Θ θ Υ υ ι Φ φ κ χ Λ λ Ψ ψ µ Ω ω Def, Prop, Th, Lem, Note, Remark, Ex,, Proof, R, N, Q, C [a, b {x R : a x b} : a, b {x R : a < x < b} : [a, b {x R : a x < b} : a,
9 α ν β Ξ ξ Γ γ o δ Π π ε ρ ζ Σ σ η τ Θ θ Υ υ ι Φ φ κ χ Λ λ Ψ ψ µ Ω ω Def, Prop, Th, Lem, Note, Remark, Ex,, Proof, R, N, Q, C [a, b {x R : a x b} : a, b {x R : a < x < b} : [a, b {x R : a x < b} : a,
R/S.5.72 (LongTerm Strage 1965) NASA (?. 2? (-:2)> 2?.2 NB. -: is half
 SHIMURA Masato JCD2773@nifty.ne.jp 29 6 19 1 R/S 1 2 9 3 References 22 C.Reiter 5 1 R/S 1.1 Harold Edwin Hurst 188-1978 England) Leicester, Oxford 3 196 Sir Henry Lyons ( 1915 1946 Hurst Black Simaika
SHIMURA Masato JCD2773@nifty.ne.jp 29 6 19 1 R/S 1 2 9 3 References 22 C.Reiter 5 1 R/S 1.1 Harold Edwin Hurst 188-1978 England) Leicester, Oxford 3 196 Sir Henry Lyons ( 1915 1946 Hurst Black Simaika
a b GE(General Erectrics) 9 4 irr (JAPLA 2009/12) Example1 120 P = C r + C 2 (1 + r) C t 1 (1 + r) t 1 + C t + F (1 + r) t 10
 1 SHIMURA Masato 2010 9 27 1 1 2 CF 6 3 10 *1 irr irr irr(inner rate of return)function is able to written only few lines,and it is very powerful and useful for simulate unprofitable business model. 1
1 SHIMURA Masato 2010 9 27 1 1 2 CF 6 3 10 *1 irr irr irr(inner rate of return)function is able to written only few lines,and it is very powerful and useful for simulate unprofitable business model. 1
.1 A cos 2π 3 sin 2π 3 sin 2π 3 cos 2π 3 T ra 2 deta T ra 2 deta T ra 2 deta a + d 2 ad bc a 2 + d 2 + ad + bc A 3 a b a 2 + bc ba + d c d ca + d bc +
 .1 n.1 1 A T ra A A a b c d A 2 a b a b c d c d a 2 + bc ab + bd ac + cd bc + d 2 a 2 + bc ba + d ca + d bc + d 2 A a + d b c T ra A T ra A 2 A 2 A A 2 A 2 A n A A n cos 2π sin 2π n n A k sin 2π cos 2π
.1 n.1 1 A T ra A A a b c d A 2 a b a b c d c d a 2 + bc ab + bd ac + cd bc + d 2 a 2 + bc ba + d ca + d bc + d 2 A a + d b c T ra A T ra A 2 A 2 A A 2 A 2 A n A A n cos 2π sin 2π n n A k sin 2π cos 2π
v er.1/ c /(21)
 12 -- 1 1 2009 1 17 1-1 1-2 1-3 1-4 2 2 2 1-5 1 1-6 1 1-7 1-1 1-2 1-3 1-4 1-5 1-6 1-7 c 2011 1/(21) 12 -- 1 -- 1 1--1 1--1--1 1 2009 1 n n α { n } α α { n } lim n = α, n α n n ε n > N n α < ε N {1, 1,
12 -- 1 1 2009 1 17 1-1 1-2 1-3 1-4 2 2 2 1-5 1 1-6 1 1-7 1-1 1-2 1-3 1-4 1-5 1-6 1-7 c 2011 1/(21) 12 -- 1 -- 1 1--1 1--1--1 1 2009 1 n n α { n } α α { n } lim n = α, n α n n ε n > N n α < ε N {1, 1,
漸化式のすべてのパターンを解説しましたー高校数学の達人・河見賢司のサイト
 https://www.hmg-gen.com/tuusin.html https://www.hmg-gen.com/tuusin1.html 1 2 OK 3 4 {a n } (1) a 1 = 1, a n+1 a n = 2 (2) a 1 = 3, a n+1 a n = 2n a n a n+1 a n = ( ) a n+1 a n = ( ) a n+1 a n {a n } 1,
https://www.hmg-gen.com/tuusin.html https://www.hmg-gen.com/tuusin1.html 1 2 OK 3 4 {a n } (1) a 1 = 1, a n+1 a n = 2 (2) a 1 = 3, a n+1 a n = 2n a n a n+1 a n = ( ) a n+1 a n = ( ) a n+1 a n {a n } 1,
2S III IV K A4 12:00-13:30 Cafe David 1 2 TA 1 appointment Cafe David K2-2S04-00 : C
 2S III IV K200 : April 16, 2004 Version : 1.1 TA M2 TA 1 10 2 n 1 ɛ-δ 5 15 20 20 45 K2-2S04-00 : C 2S III IV K200 60 60 74 75 89 90 1 email 3 4 30 A4 12:00-13:30 Cafe David 1 2 TA 1 email appointment Cafe
2S III IV K200 : April 16, 2004 Version : 1.1 TA M2 TA 1 10 2 n 1 ɛ-δ 5 15 20 20 45 K2-2S04-00 : C 2S III IV K200 60 60 74 75 89 90 1 email 3 4 30 A4 12:00-13:30 Cafe David 1 2 TA 1 email appointment Cafe
 i 6 3 ii 3 7 8 9 3 6 iii 5 8 5 3 7 8 v...................................................... 5.3....................... 7 3........................ 3.................3.......................... 8 3 35
i 6 3 ii 3 7 8 9 3 6 iii 5 8 5 3 7 8 v...................................................... 5.3....................... 7 3........................ 3.................3.......................... 8 3 35
2012 A, N, Z, Q, R, C
 2012 A, N, Z, Q, R, C 1 2009 9 2 2011 2 3 2012 9 1 2 2 5 3 11 4 16 5 22 6 25 7 29 8 32 1 1 1.1 3 1 1 1 1 1 1? 3 3 3 3 3 3 3 1 1, 1 1 + 1 1 1+1 2 2 1 2+1 3 2 N 1.2 N (i) 2 a b a 1 b a < b a b b a a b (ii)
2012 A, N, Z, Q, R, C 1 2009 9 2 2011 2 3 2012 9 1 2 2 5 3 11 4 16 5 22 6 25 7 29 8 32 1 1 1.1 3 1 1 1 1 1 1? 3 3 3 3 3 3 3 1 1, 1 1 + 1 1 1+1 2 2 1 2+1 3 2 N 1.2 N (i) 2 a b a 1 b a < b a b b a a b (ii)
18 ( ) I II III A B C(100 ) 1, 2, 3, 5 I II A B (100 ) 1, 2, 3 I II A B (80 ) 6 8 I II III A B C(80 ) 1 n (1 + x) n (1) n C 1 + n C
 8 ( ) 8 5 4 I II III A B C( ),,, 5 I II A B ( ),, I II A B (8 ) 6 8 I II III A B C(8 ) n ( + x) n () n C + n C + + n C n = 7 n () 7 9 C : y = x x A(, 6) () A C () C P AP Q () () () 4 A(,, ) B(,, ) C(,,
8 ( ) 8 5 4 I II III A B C( ),,, 5 I II A B ( ),, I II A B (8 ) 6 8 I II III A B C(8 ) n ( + x) n () n C + n C + + n C n = 7 n () 7 9 C : y = x x A(, 6) () A C () C P AP Q () () () 4 A(,, ) B(,, ) C(,,
i 18 2H 2 + O 2 2H 2 + ( ) 3K
 i 18 2H 2 + O 2 2H 2 + ( ) 3K ii 1 1 1.1.................................. 1 1.2........................................ 3 1.3......................................... 3 1.4....................................
i 18 2H 2 + O 2 2H 2 + ( ) 3K ii 1 1 1.1.................................. 1 1.2........................................ 3 1.3......................................... 3 1.4....................................
(1) (2) (1) (2) 2 3 {a n } a 2 + a 4 + a a n S n S n = n = S n
 . 99 () 0 0 0 () 0 00 0 350 300 () 5 0 () 3 {a n } a + a 4 + a 6 + + a 40 30 53 47 77 95 30 83 4 n S n S n = n = S n 303 9 k d 9 45 k =, d = 99 a d n a n d n a n = a + (n )d a n a n S n S n = n(a + a n
. 99 () 0 0 0 () 0 00 0 350 300 () 5 0 () 3 {a n } a + a 4 + a 6 + + a 40 30 53 47 77 95 30 83 4 n S n S n = n = S n 303 9 k d 9 45 k =, d = 99 a d n a n d n a n = a + (n )d a n a n S n S n = n(a + a n
x x x 2, A 4 2 Ax.4 A A A A λ λ 4 λ 2 A λe λ λ2 5λ + 6 0,...λ 2, λ 2 3 E 0 E 0 p p Ap λp λ 2 p 4 2 p p 2 p { 4p 2 2p p + 2 p, p 2 λ {
 K E N Z OU 2008 8. 4x 2x 2 2 2 x + x 2. x 2 2x 2, 2 2 d 2 x 2 2.2 2 3x 2... d 2 x 2 5 + 6x 0 2 2 d 2 x 2 + P t + P 2tx Qx x x, x 2 2 2 x 2 P 2 tx P tx 2 + Qx x, x 2. d x 4 2 x 2 x x 2.3 x x x 2, A 4 2
K E N Z OU 2008 8. 4x 2x 2 2 2 x + x 2. x 2 2x 2, 2 2 d 2 x 2 2.2 2 3x 2... d 2 x 2 5 + 6x 0 2 2 d 2 x 2 + P t + P 2tx Qx x x, x 2 2 2 x 2 P 2 tx P tx 2 + Qx x, x 2. d x 4 2 x 2 x x 2.3 x x x 2, A 4 2
 3 m = [n, n1, n 2,..., n r, 2n] p q = [n, n 1, n 2,..., n r ] p 2 mq 2 = ±1 1 1 6 1.1................................. 6 1.2......................... 8 1.3......................... 13 2 15 2.1.............................
3 m = [n, n1, n 2,..., n r, 2n] p q = [n, n 1, n 2,..., n r ] p 2 mq 2 = ±1 1 1 6 1.1................................. 6 1.2......................... 8 1.3......................... 13 2 15 2.1.............................
J6 M.Shimura (1) 1 2 (2) (1824) ( (1842) 1 (1) 1.1 C.Reiter dwin require ad
 J6 M.Shimura JCD02773@nifty.ne.jp 2011 12 13 1 (1) 1 2 (2)- 12 3 14 4-20 5 24 6 33 60 (1824) ( 100 1760 1849 (1842) 1 (1) 1.1 C.Reiter dwin require addons/graphics/fvj3/dwin2.ijs 1 xy find_maxmin 4 5 calc_each_poly
J6 M.Shimura JCD02773@nifty.ne.jp 2011 12 13 1 (1) 1 2 (2)- 12 3 14 4-20 5 24 6 33 60 (1824) ( 100 1760 1849 (1842) 1 (1) 1.1 C.Reiter dwin require addons/graphics/fvj3/dwin2.ijs 1 xy find_maxmin 4 5 calc_each_poly
(3) (2),,. ( 20) ( s200103) 0.7 x C,, x 2 + y 2 + ax = 0 a.. D,. D, y C, C (x, y) (y 0) C m. (2) D y = y(x) (x ± y 0), (x, y) D, m, m = 1., D. (x 2 y
 [ ] 7 0.1 2 2 + y = t sin t IC ( 9) ( s090101) 0.2 y = d2 y 2, y = x 3 y + y 2 = 0 (2) y + 2y 3y = e 2x 0.3 1 ( y ) = f x C u = y x ( 15) ( s150102) [ ] y/x du x = Cexp f(u) u (2) x y = xey/x ( 16) ( s160101)
[ ] 7 0.1 2 2 + y = t sin t IC ( 9) ( s090101) 0.2 y = d2 y 2, y = x 3 y + y 2 = 0 (2) y + 2y 3y = e 2x 0.3 1 ( y ) = f x C u = y x ( 15) ( s150102) [ ] y/x du x = Cexp f(u) u (2) x y = xey/x ( 16) ( s160101)
数値計算:有限要素法
 ( ) 1 / 61 1 2 3 4 ( ) 2 / 61 ( ) 3 / 61 P(0) P(x) u(x) P(L) f P(0) P(x) P(L) ( ) 4 / 61 L P(x) E(x) A(x) x P(x) P(x) u(x) P(x) u(x) (0 x L) ( ) 5 / 61 u(x) 0 L x ( ) 6 / 61 P(0) P(L) f d dx ( EA du dx
( ) 1 / 61 1 2 3 4 ( ) 2 / 61 ( ) 3 / 61 P(0) P(x) u(x) P(L) f P(0) P(x) P(L) ( ) 4 / 61 L P(x) E(x) A(x) x P(x) P(x) u(x) P(x) u(x) (0 x L) ( ) 5 / 61 u(x) 0 L x ( ) 6 / 61 P(0) P(L) f d dx ( EA du dx
.3. (x, x = (, u = = 4 (, x x = 4 x, x 0 x = 0 x = 4 x.4. ( z + z = 8 z, z 0 (z, z = (0, 8, (,, (8, 0 3 (0, 8, (,, (8, 0 z = z 4 z (g f(x = g(
 06 5.. ( y = x x y 5 y 5 = (x y = x + ( y = x + y = x y.. ( Y = C + I = 50 + 0.5Y + 50 r r = 00 0.5Y ( L = M Y r = 00 r = 0.5Y 50 (3 00 0.5Y = 0.5Y 50 Y = 50, r = 5 .3. (x, x = (, u = = 4 (, x x = 4 x,
06 5.. ( y = x x y 5 y 5 = (x y = x + ( y = x + y = x y.. ( Y = C + I = 50 + 0.5Y + 50 r r = 00 0.5Y ( L = M Y r = 00 r = 0.5Y 50 (3 00 0.5Y = 0.5Y 50 Y = 50, r = 5 .3. (x, x = (, u = = 4 (, x x = 4 x,
II A A441 : October 02, 2014 Version : Kawahira, Tomoki TA (Kondo, Hirotaka )
 II 214-1 : October 2, 214 Version : 1.1 Kawahira, Tomoki TA (Kondo, Hirotaka ) http://www.math.nagoya-u.ac.jp/~kawahira/courses/14w-biseki.html pdf 1 2 1 9 1 16 1 23 1 3 11 6 11 13 11 2 11 27 12 4 12 11
II 214-1 : October 2, 214 Version : 1.1 Kawahira, Tomoki TA (Kondo, Hirotaka ) http://www.math.nagoya-u.ac.jp/~kawahira/courses/14w-biseki.html pdf 1 2 1 9 1 16 1 23 1 3 11 6 11 13 11 2 11 27 12 4 12 11
agora04.dvi
 Workbook E-mail: kawahira@math.nagoya-u.ac.jp 2004 8 9, 10, 11 1 2 1 2 a n+1 = pa n + q x = px + q a n better 2 a n+1 = aan+b ca n+d 1 (a, b, c, d) =(p, q, 0, 1) 1 = 0 3 2 2 2 f(z) =z 2 + c a n+1 = a 2
Workbook E-mail: kawahira@math.nagoya-u.ac.jp 2004 8 9, 10, 11 1 2 1 2 a n+1 = pa n + q x = px + q a n better 2 a n+1 = aan+b ca n+d 1 (a, b, c, d) =(p, q, 0, 1) 1 = 0 3 2 2 2 f(z) =z 2 + c a n+1 = a 2
> > <., vs. > x 2 x y = ax 2 + bx + c y = 0 2 ax 2 + bx + c = 0 y = 0 x ( x ) y = ax 2 + bx + c D = b 2 4ac (1) D > 0 x (2) D = 0 x (3
 13 2 13.0 2 ( ) ( ) 2 13.1 ( ) ax 2 + bx + c > 0 ( a, b, c ) ( ) 275 > > 2 2 13.3 x 2 x y = ax 2 + bx + c y = 0 2 ax 2 + bx + c = 0 y = 0 x ( x ) y = ax 2 + bx + c D = b 2 4ac (1) D >
13 2 13.0 2 ( ) ( ) 2 13.1 ( ) ax 2 + bx + c > 0 ( a, b, c ) ( ) 275 > > 2 2 13.3 x 2 x y = ax 2 + bx + c y = 0 2 ax 2 + bx + c = 0 y = 0 x ( x ) y = ax 2 + bx + c D = b 2 4ac (1) D >
D xy D (x, y) z = f(x, y) f D (2 ) (x, y, z) f R z = 1 x 2 y 2 {(x, y); x 2 +y 2 1} x 2 +y 2 +z 2 = 1 1 z (x, y) R 2 z = x 2 y
 5 5. 2 D xy D (x, y z = f(x, y f D (2 (x, y, z f R 2 5.. z = x 2 y 2 {(x, y; x 2 +y 2 } x 2 +y 2 +z 2 = z 5.2. (x, y R 2 z = x 2 y + 3 (2,,, (, 3,, 3 (,, 5.3 (. (3 ( (a, b, c A : (x, y, z P : (x, y, x
5 5. 2 D xy D (x, y z = f(x, y f D (2 (x, y, z f R 2 5.. z = x 2 y 2 {(x, y; x 2 +y 2 } x 2 +y 2 +z 2 = z 5.2. (x, y R 2 z = x 2 y + 3 (2,,, (, 3,, 3 (,, 5.3 (. (3 ( (a, b, c A : (x, y, z P : (x, y, x
II (10 4 ) 1. p (x, y) (a, b) ε(x, y; a, b) 0 f (x, y) f (a, b) A, B (6.5) y = b f (x, b) f (a, b) x a = A + ε(x, b; a, b) x a 2 x a 0 A = f x (
 II (1 4 ) 1. p.13 1 (x, y) (a, b) ε(x, y; a, b) f (x, y) f (a, b) A, B (6.5) y = b f (x, b) f (a, b) x a = A + ε(x, b; a, b) x a x a A = f x (a, b) y x 3 3y 3 (x, y) (, ) f (x, y) = x + y (x, y) = (, )
II (1 4 ) 1. p.13 1 (x, y) (a, b) ε(x, y; a, b) f (x, y) f (a, b) A, B (6.5) y = b f (x, b) f (a, b) x a = A + ε(x, b; a, b) x a x a A = f x (a, b) y x 3 3y 3 (x, y) (, ) f (x, y) = x + y (x, y) = (, )
1 8, : 8.1 1, 2 z = ax + by + c ax by + z c = a b +1 x y z c = 0, (0, 0, c), n = ( a, b, 1). f = n i=1 a ii x 2 i + i<j 2a ij x i x j = ( x, A x), f =
 1 8, : 8.1 1, z = ax + by + c ax by + z c = a b +1 x y z c = 0, (0, 0, c), n = ( a, b, 1). f = a ii x i + i
1 8, : 8.1 1, z = ax + by + c ax by + z c = a b +1 x y z c = 0, (0, 0, c), n = ( a, b, 1). f = a ii x i + i
( )
 18 10 01 ( ) 1 2018 4 1.1 2018............................... 4 1.2 2018......................... 5 2 2017 7 2.1 2017............................... 7 2.2 2017......................... 8 3 2016 9 3.1 2016...............................
18 10 01 ( ) 1 2018 4 1.1 2018............................... 4 1.2 2018......................... 5 2 2017 7 2.1 2017............................... 7 2.2 2017......................... 8 3 2016 9 3.1 2016...............................
名古屋工業大の数学 2000 年 ~2015 年 大学入試数学動画解説サイト
 名古屋工業大の数学 年 ~5 年 大学入試数学動画解説サイト http://mathroom.jugem.jp/ 68 i 4 3 III III 3 5 3 ii 5 6 45 99 5 4 3. () r \= S n = r + r + 3r 3 + + nr n () x > f n (x) = e x + e x + 3e 3x + + ne nx f(x) = lim f n(x) lim
名古屋工業大の数学 年 ~5 年 大学入試数学動画解説サイト http://mathroom.jugem.jp/ 68 i 4 3 III III 3 5 3 ii 5 6 45 99 5 4 3. () r \= S n = r + r + 3r 3 + + nr n () x > f n (x) = e x + e x + 3e 3x + + ne nx f(x) = lim f n(x) lim
Part () () Γ Part ,
 Contents a 6 6 6 6 6 6 6 7 7. 8.. 8.. 8.3. 8 Part. 9. 9.. 9.. 3. 3.. 3.. 3 4. 5 4.. 5 4.. 9 4.3. 3 Part. 6 5. () 6 5.. () 7 5.. 9 5.3. Γ 3 6. 3 6.. 3 6.. 3 6.3. 33 Part 3. 34 7. 34 7.. 34 7.. 34 8. 35
Contents a 6 6 6 6 6 6 6 7 7. 8.. 8.. 8.3. 8 Part. 9. 9.. 9.. 3. 3.. 3.. 3 4. 5 4.. 5 4.. 9 4.3. 3 Part. 6 5. () 6 5.. () 7 5.. 9 5.3. Γ 3 6. 3 6.. 3 6.. 3 6.3. 33 Part 3. 34 7. 34 7.. 34 7.. 34 8. 35
webkaitou.dvi
 ( c Akir KANEKO) ).. m. l s = lθ m d s dt = mg sin θ d θ dt = g l sinθ θ l θ mg. d s dt xy t ( d x dt, d y dt ) t ( mg sin θ cos θ, sin θ sin θ). (.) m t ( d x dt, d y dt ) = t ( mg sin θ cos θ, mg sin
( c Akir KANEKO) ).. m. l s = lθ m d s dt = mg sin θ d θ dt = g l sinθ θ l θ mg. d s dt xy t ( d x dt, d y dt ) t ( mg sin θ cos θ, sin θ sin θ). (.) m t ( d x dt, d y dt ) = t ( mg sin θ cos θ, mg sin
2000年度『数学展望 I』講義録
 2000 I I IV I II 2000 I I IV I-IV. i ii 3.10 (http://www.math.nagoya-u.ac.jp/ kanai/) 2000 A....1 B....4 C....10 D....13 E....17 Brouwer A....21 B....26 C....33 D....39 E. Sperner...45 F....48 A....53
2000 I I IV I II 2000 I I IV I-IV. i ii 3.10 (http://www.math.nagoya-u.ac.jp/ kanai/) 2000 A....1 B....4 C....10 D....13 E....17 Brouwer A....21 B....26 C....33 D....39 E. Sperner...45 F....48 A....53
z f(z) f(z) x, y, u, v, r, θ r > 0 z = x + iy, f = u + iv C γ D f(z) f(z) D f(z) f(z) z, Rm z, z 1.1 z = x + iy = re iθ = r (cos θ + i sin θ) z = x iy
 f f x, y, u, v, r, θ r > = x + iy, f = u + iv C γ D f f D f f, Rm,. = x + iy = re iθ = r cos θ + i sin θ = x iy = re iθ = r cos θ i sin θ x = + = Re, y = = Im i r = = = x + y θ = arg = arctan y x e i =
f f x, y, u, v, r, θ r > = x + iy, f = u + iv C γ D f f D f f, Rm,. = x + iy = re iθ = r cos θ + i sin θ = x iy = re iθ = r cos θ i sin θ x = + = Re, y = = Im i r = = = x + y θ = arg = arctan y x e i =
No2 4 y =sinx (5) y = p sin(2x +3) (6) y = 1 tan(3x 2) (7) y =cos 2 (4x +5) (8) y = cos x 1+sinx 5 (1) y =sinx cos x 6 f(x) = sin(sin x) f 0 (π) (2) y
 No1 1 (1) 2 f(x) =1+x + x 2 + + x n, g(x) = 1 (n +1)xn + nx n+1 (1 x) 2 x 6= 1 f 0 (x) =g(x) y = f(x)g(x) y 0 = f 0 (x)g(x)+f(x)g 0 (x) 3 (1) y = x2 x +1 x (2) y = 1 g(x) y0 = g0 (x) {g(x)} 2 (2) y = µ
No1 1 (1) 2 f(x) =1+x + x 2 + + x n, g(x) = 1 (n +1)xn + nx n+1 (1 x) 2 x 6= 1 f 0 (x) =g(x) y = f(x)g(x) y 0 = f 0 (x)g(x)+f(x)g 0 (x) 3 (1) y = x2 x +1 x (2) y = 1 g(x) y0 = g0 (x) {g(x)} 2 (2) y = µ
 1. A0 A B A0 A : A1,...,A5 B : B1,...,B12 2. 5 3. 4. 5. A0 (1) A, B A B f K K A ϕ 1, ϕ 2 f ϕ 1 = f ϕ 2 ϕ 1 = ϕ 2 (2) N A 1, A 2, A 3,... N A n X N n X N, A n N n=1 1 A1 d (d 2) A (, k A k = O), A O. f
1. A0 A B A0 A : A1,...,A5 B : B1,...,B12 2. 5 3. 4. 5. A0 (1) A, B A B f K K A ϕ 1, ϕ 2 f ϕ 1 = f ϕ 2 ϕ 1 = ϕ 2 (2) N A 1, A 2, A 3,... N A n X N n X N, A n N n=1 1 A1 d (d 2) A (, k A k = O), A O. f
17 ( ) II III A B C(100 ) 1, 2, 6, 7 II A B (100 ) 2, 5, 6 II A B (80 ) 8 10 I II III A B C(80 ) 1 a 1 = 1 2 a n+1 = a n + 2n + 1 (n = 1,
 17 ( ) 17 5 1 4 II III A B C(1 ) 1,, 6, 7 II A B (1 ), 5, 6 II A B (8 ) 8 1 I II III A B C(8 ) 1 a 1 1 a n+1 a n + n + 1 (n 1,,, ) {a n+1 n } (1) a 4 () a n OA OB AOB 6 OAB AB : 1 P OB Q OP AQ R (1) PQ
17 ( ) 17 5 1 4 II III A B C(1 ) 1,, 6, 7 II A B (1 ), 5, 6 II A B (8 ) 8 1 I II III A B C(8 ) 1 a 1 1 a n+1 a n + n + 1 (n 1,,, ) {a n+1 n } (1) a 4 () a n OA OB AOB 6 OAB AB : 1 P OB Q OP AQ R (1) PQ
 25 7 18 1 1 1.1 v.s............................. 1 1.1.1.................................. 1 1.1.2................................. 1 1.1.3.................................. 3 1.2................... 3
25 7 18 1 1 1.1 v.s............................. 1 1.1.1.................................. 1 1.1.2................................. 1 1.1.3.................................. 3 1.2................... 3
OABC OA OC 4, OB, AOB BOC COA 60 OA a OB b OC c () AB AC () ABC D OD ABC OD OA + p AB + q AC p q () OABC 4 f(x) + x ( ), () y f(x) P l 4 () y f(x) l P
 4 ( ) ( ) ( ) ( ) 4 5 5 II III A B (0 ) 4, 6, 7 II III A B (0 ) ( ),, 6, 8, 9 II III A B (0 ) ( [ ] ) 5, 0, II A B (90 ) log x x () (a) y x + x (b) y sin (x + ) () (a) (b) (c) (d) 0 e π 0 x x x + dx e
4 ( ) ( ) ( ) ( ) 4 5 5 II III A B (0 ) 4, 6, 7 II III A B (0 ) ( ),, 6, 8, 9 II III A B (0 ) ( [ ] ) 5, 0, II A B (90 ) log x x () (a) y x + x (b) y sin (x + ) () (a) (b) (c) (d) 0 e π 0 x x x + dx e
_TZ_4797-haus-local
 1.1.................................... 3.3.................................. 4.4......................... 8.5... 10.6.................... 1.7... 14 3 16 3.1 ()........................... 16 3. 7... 17
1.1.................................... 3.3.................................. 4.4......................... 8.5... 10.6.................... 1.7... 14 3 16 3.1 ()........................... 16 3. 7... 17
熊本県数学問題正解
 00 y O x Typed by L A TEX ε ( ) (00 ) 5 4 4 ( ) http://www.ocn.ne.jp/ oboetene/plan/. ( ) (009 ) ( ).. http://www.ocn.ne.jp/ oboetene/plan/eng.html 8 i i..................................... ( )0... (
00 y O x Typed by L A TEX ε ( ) (00 ) 5 4 4 ( ) http://www.ocn.ne.jp/ oboetene/plan/. ( ) (009 ) ( ).. http://www.ocn.ne.jp/ oboetene/plan/eng.html 8 i i..................................... ( )0... (
入試の軌跡
 4 y O x 4 Typed by L A TEX ε ) ) ) 6 4 ) 4 75 ) http://kumamoto.s.xrea.com/plan/.. PDF) Ctrl +L) Ctrl +) Ctrl + Ctrl + ) ) Alt + ) Alt + ) ESC. http://kumamoto.s.xrea.com/nyusi/kumadai kiseki ri i.pdf
4 y O x 4 Typed by L A TEX ε ) ) ) 6 4 ) 4 75 ) http://kumamoto.s.xrea.com/plan/.. PDF) Ctrl +L) Ctrl +) Ctrl + Ctrl + ) ) Alt + ) Alt + ) ESC. http://kumamoto.s.xrea.com/nyusi/kumadai kiseki ri i.pdf
6. Euler x
 ...............................................................................3......................................... 4.4................................... 5.5......................................
...............................................................................3......................................... 4.4................................... 5.5......................................
1.2 y + P (x)y + Q(x)y = 0 (1) y 1 (x), y 2 (x) y 1 (x), y 2 (x) (1) y(x) c 1, c 2 y(x) = c 1 y 1 (x) + c 2 y 2 (x) 3 y 1 (x) y 1 (x) e R P (x)dx y 2
 1 1.1 R(x) = 0 y + P (x)y + Q(x)y = R(x)...(1) y + P (x)y + Q(x)y = 0...(2) 1 2 u(x) v(x) c 1 u(x)+ c 2 v(x) = 0 c 1 = c 2 = 0 c 1 = c 2 = 0 2 0 2 u(x) v(x) u(x) u (x) W (u, v)(x) = v(x) v (x) 0 1 1.2
1 1.1 R(x) = 0 y + P (x)y + Q(x)y = R(x)...(1) y + P (x)y + Q(x)y = 0...(2) 1 2 u(x) v(x) c 1 u(x)+ c 2 v(x) = 0 c 1 = c 2 = 0 c 1 = c 2 = 0 2 0 2 u(x) v(x) u(x) u (x) W (u, v)(x) = v(x) v (x) 0 1 1.2
15 P3 Pm C.Reiter dwin C.Reiter Fractal Visualization and J 4th edition fvj4 J 2D gl2 J addon Appendix (hokusai olympic0.ijs dwin * 1 coinsert *
 SHIMURA Masato JCD02773@nifty.ne.jp 2017 2 23 1 2 2 6 3 9 4 15 A J 21 2 3 45 1 15 P3 Pm 1 1.1 C.Reiter dwin C.Reiter Fractal Visualization and J 4th edition fvj4 J 2D gl2 J addon Appendix (hokusai olympic0.ijs
SHIMURA Masato JCD02773@nifty.ne.jp 2017 2 23 1 2 2 6 3 9 4 15 A J 21 2 3 45 1 15 P3 Pm 1 1.1 C.Reiter dwin C.Reiter Fractal Visualization and J 4th edition fvj4 J 2D gl2 J addon Appendix (hokusai olympic0.ijs
0 1-4. 1-5. (1) + b = b +, (2) b = b, (3) + 0 =, (4) 1 =, (5) ( + b) + c = + (b + c), (6) ( b) c = (b c), (7) (b + c) = b + c, (8) ( + b)c = c + bc (9
 1-1. 1, 2, 3, 4, 5, 6, 7,, 100,, 1000, n, m m m n n 0 n, m m n 1-2. 0 m n m n 0 2 = 1.41421356 π = 3.141516 1-3. 1 0 1-4. 1-5. (1) + b = b +, (2) b = b, (3) + 0 =, (4) 1 =, (5) ( + b) + c = + (b + c),
1-1. 1, 2, 3, 4, 5, 6, 7,, 100,, 1000, n, m m m n n 0 n, m m n 1-2. 0 m n m n 0 2 = 1.41421356 π = 3.141516 1-3. 1 0 1-4. 1-5. (1) + b = b +, (2) b = b, (3) + 0 =, (4) 1 =, (5) ( + b) + c = + (b + c),
, x R, f (x),, df dx : R R,, f : R R, f(x) ( ).,, f (a) d f dx (a), f (a) d3 f dx 3 (a),, f (n) (a) dn f dx n (a), f d f dx, f d3 f dx 3,, f (n) dn f
 ,,,,.,,,. R f : R R R a R, f(a + ) f(a) lim 0 (), df dx (a) f (a), f(x) x a, f (a), f(x) x a ( ). y f(a + ) y f(x) f(a+) f(a) f(a + ) f(a) f(a) x a 0 a a + x 0 a a + x y y f(x) 0 : 0, f(a+) f(a)., f(x)
,,,,.,,,. R f : R R R a R, f(a + ) f(a) lim 0 (), df dx (a) f (a), f(x) x a, f (a), f(x) x a ( ). y f(a + ) y f(x) f(a+) f(a) f(a + ) f(a) f(a) x a 0 a a + x 0 a a + x y y f(x) 0 : 0, f(a+) f(a)., f(x)
A S- hara/lectures/lectures-j.html r A = A 5 : 5 = max{ A, } A A A A B A, B A A A %
 A S- http://www.math.kyushu-u.ac.jp/ hara/lectures/lectures-j.html r A S- 3.4.5. 9 phone: 9-8-444, e-mail: hara@math.kyushu-u.ac.jp, http://www.math.kyushu-u.ac.jp/ hara/lectures/lectures-j.html Office
A S- http://www.math.kyushu-u.ac.jp/ hara/lectures/lectures-j.html r A S- 3.4.5. 9 phone: 9-8-444, e-mail: hara@math.kyushu-u.ac.jp, http://www.math.kyushu-u.ac.jp/ hara/lectures/lectures-j.html Office
I A A441 : April 15, 2013 Version : 1.1 I Kawahira, Tomoki TA (Shigehiro, Yoshida )
 I013 00-1 : April 15, 013 Version : 1.1 I Kawahira, Tomoki TA (Shigehiro, Yoshida) http://www.math.nagoya-u.ac.jp/~kawahira/courses/13s-tenbou.html pdf * 4 15 4 5 13 e πi = 1 5 0 5 7 3 4 6 3 6 10 6 17
I013 00-1 : April 15, 013 Version : 1.1 I Kawahira, Tomoki TA (Shigehiro, Yoshida) http://www.math.nagoya-u.ac.jp/~kawahira/courses/13s-tenbou.html pdf * 4 15 4 5 13 e πi = 1 5 0 5 7 3 4 6 3 6 10 6 17
combine: combine(exp1 ) collect: collect(exp1,x) convert: convert(exp1,opt ) > convert(sin(x),exp); > convert(sinh(x),exp); > convert(exp(i*x),trig);
 1 Maple exp Maple 1.1 1.1.1 solve( ), diff( ), int( ), 1: simplify: lhs, rhs: subs: expand: numer, denom: assume: factor: coeff: assuming: normal: nops, op assign: combine: about: collect: anames( user
1 Maple exp Maple 1.1 1.1.1 solve( ), diff( ), int( ), 1: simplify: lhs, rhs: subs: expand: numer, denom: assume: factor: coeff: assuming: normal: nops, op assign: combine: about: collect: anames( user
2.2 ( y = y(x ( (x 0, y 0 y (x 0 (y 0 = y(x 0 y = y(x ( y (x 0 = F (x 0, y(x 0 = F (x 0, y 0 (x 0, y 0 ( (x 0, y 0 F (x 0, y 0 xy (x, y (, F (x, y ( (
 (. x y y x f y = f(x y x y = y(x y x y dx = d dx y(x = y (x = f (x y = y(x x ( (differential equation ( + y 2 dx + xy = 0 dx = xy + y 2 2 2 x y 2 F (x, y = xy + y 2 y = y(x x x xy(x = F (x, y(x + y(x 2
(. x y y x f y = f(x y x y = y(x y x y dx = d dx y(x = y (x = f (x y = y(x x ( (differential equation ( + y 2 dx + xy = 0 dx = xy + y 2 2 2 x y 2 F (x, y = xy + y 2 y = y(x x x xy(x = F (x, y(x + y(x 2
II No.01 [n/2] [1]H n (x) H n (x) = ( 1) r n! r!(n 2r)! (2x)n 2r. r=0 [2]H n (x) n,, H n ( x) = ( 1) n H n (x). [3] H n (x) = ( 1) n dn x2 e dx n e x2
![II No.01 [n/2] [1]H n (x) H n (x) = ( 1) r n! r!(n 2r)! (2x)n 2r. r=0 [2]H n (x) n,, H n ( x) = ( 1) n H n (x). [3] H n (x) = ( 1) n dn x2 e dx n e x2 II No.01 [n/2] [1]H n (x) H n (x) = ( 1) r n! r!(n 2r)! (2x)n 2r. r=0 [2]H n (x) n,, H n ( x) = ( 1) n H n (x). [3] H n (x) = ( 1) n dn x2 e dx n e x2](/thumbs/101/149159646.jpg) II No.1 [n/] [1]H n x) H n x) = 1) r n! r!n r)! x)n r r= []H n x) n,, H n x) = 1) n H n x) [3] H n x) = 1) n dn x e dx n e x [4] H n+1 x) = xh n x) nh n 1 x) ) d dx x H n x) = H n+1 x) d dx H nx) = nh
II No.1 [n/] [1]H n x) H n x) = 1) r n! r!n r)! x)n r r= []H n x) n,, H n x) = 1) n H n x) [3] H n x) = 1) n dn x e dx n e x [4] H n+1 x) = xh n x) nh n 1 x) ) d dx x H n x) = H n+1 x) d dx H nx) = nh
untitled
 . x2.0 0.5 0 0.5.0 x 2 t= 0: : x α ij β j O x2 u I = α x j ij i i= 0 y j = + exp( u ) j v J = β y j= 0 j j o = + exp( v ) 0 0 e x p e x p J j I j ij i i o x β α = = = + +.. 2 3 8 x 75 58 28 36 x2 3 3 4
. x2.0 0.5 0 0.5.0 x 2 t= 0: : x α ij β j O x2 u I = α x j ij i i= 0 y j = + exp( u ) j v J = β y j= 0 j j o = + exp( v ) 0 0 e x p e x p J j I j ij i i o x β α = = = + +.. 2 3 8 x 75 58 28 36 x2 3 3 4
9. 05 L x P(x) P(0) P(x) u(x) u(x) (0 < = x < = L) P(x) E(x) A(x) P(L) f ( d EA du ) = 0 (9.) dx dx u(0) = 0 (9.2) E(L)A(L) du (L) = f (9.3) dx (9.) P
 9 (Finite Element Method; FEM) 9. 9. P(0) P(x) u(x) (a) P(L) f P(0) P(x) (b) 9. P(L) 9. 05 L x P(x) P(0) P(x) u(x) u(x) (0 < = x < = L) P(x) E(x) A(x) P(L) f ( d EA du ) = 0 (9.) dx dx u(0) = 0 (9.2) E(L)A(L)
9 (Finite Element Method; FEM) 9. 9. P(0) P(x) u(x) (a) P(L) f P(0) P(x) (b) 9. P(L) 9. 05 L x P(x) P(0) P(x) u(x) u(x) (0 < = x < = L) P(x) E(x) A(x) P(L) f ( d EA du ) = 0 (9.) dx dx u(0) = 0 (9.2) E(L)A(L)
 20 6 4 1 4 1.1 1.................................... 4 1.1.1.................................... 4 1.1.2 1................................ 5 1.2................................... 7 1.2.1....................................
20 6 4 1 4 1.1 1.................................... 4 1.1.1.................................... 4 1.1.2 1................................ 5 1.2................................... 7 1.2.1....................................
S I. dy fx x fx y fx + C 3 C vt dy fx 4 x, y dy yt gt + Ct + C dt v e kt xt v e kt + C k x v k + C C xt v k 3 r r + dr e kt S Sr πr dt d v } dt k e kt
 S I. x yx y y, y,. F x, y, y, y,, y n http://ayapin.film.s.dendai.ac.jp/~matuda n /TeX/lecture.html PDF PS yx.................................... 3.3.................... 9.4................5..............
S I. x yx y y, y,. F x, y, y, y,, y n http://ayapin.film.s.dendai.ac.jp/~matuda n /TeX/lecture.html PDF PS yx.................................... 3.3.................... 9.4................5..............
i
 009 I 1 8 5 i 0 1 0.1..................................... 1 0.................................................. 1 0.3................................. 0.4........................................... 3
009 I 1 8 5 i 0 1 0.1..................................... 1 0.................................................. 1 0.3................................. 0.4........................................... 3
, = = 7 6 = 42, =
 http://www.ss.u-tokai.ac.jp/~mahoro/2016autumn/alg_intro/ 1 1 2016.9.26, http://www.ss.u-tokai.ac.jp/~mahoro/2016autumn/alg_intro/ 1.1 1 214 132 = 28258 2 + 1 + 4 1 + 3 + 2 = 7 6 = 42, 4 + 2 = 6 2 + 8
http://www.ss.u-tokai.ac.jp/~mahoro/2016autumn/alg_intro/ 1 1 2016.9.26, http://www.ss.u-tokai.ac.jp/~mahoro/2016autumn/alg_intro/ 1.1 1 214 132 = 28258 2 + 1 + 4 1 + 3 + 2 = 7 6 = 42, 4 + 2 = 6 2 + 8
2012 IA 8 I p.3, 2 p.19, 3 p.19, 4 p.22, 5 p.27, 6 p.27, 7 p
 2012 IA 8 I 1 10 10 29 1. [0, 1] n x = 1 (n = 1, 2, 3,...) 2 f(x) = n 0 [0, 1] 2. 1 x = 1 (n = 1, 2, 3,...) 2 f(x) = n 0 [0, 1] 1 0 f(x)dx 3. < b < c [, c] b [, c] 4. [, b] f(x) 1 f(x) 1 f(x) [, b] 5.
2012 IA 8 I 1 10 10 29 1. [0, 1] n x = 1 (n = 1, 2, 3,...) 2 f(x) = n 0 [0, 1] 2. 1 x = 1 (n = 1, 2, 3,...) 2 f(x) = n 0 [0, 1] 1 0 f(x)dx 3. < b < c [, c] b [, c] 4. [, b] f(x) 1 f(x) 1 f(x) [, b] 5.
untitled
 1 ( 12 11 44 7 20 10 10 1 1 ( ( 2 10 46 11 10 10 5 8 3 2 6 9 47 2 3 48 4 2 2 ( 97 12 ) 97 12 -Spencer modulus moduli (modulus of elasticity) modulus (le) module modulus module 4 b θ a q φ p 1: 3 (le) module
1 ( 12 11 44 7 20 10 10 1 1 ( ( 2 10 46 11 10 10 5 8 3 2 6 9 47 2 3 48 4 2 2 ( 97 12 ) 97 12 -Spencer modulus moduli (modulus of elasticity) modulus (le) module modulus module 4 b θ a q φ p 1: 3 (le) module
 211 kotaro@math.titech.ac.jp 1 R *1 n n R n *2 R n = {(x 1,..., x n ) x 1,..., x n R}. R R 2 R 3 R n R n R n D D R n *3 ) (x 1,..., x n ) f(x 1,..., x n ) f D *4 n 2 n = 1 ( ) 1 f D R n f : D R 1.1. (x,
211 kotaro@math.titech.ac.jp 1 R *1 n n R n *2 R n = {(x 1,..., x n ) x 1,..., x n R}. R R 2 R 3 R n R n R n D D R n *3 ) (x 1,..., x n ) f(x 1,..., x n ) f D *4 n 2 n = 1 ( ) 1 f D R n f : D R 1.1. (x,
DVIOUT
 A. A. A-- [ ] f(x) x = f 00 (x) f 0 () =0 f 00 () > 0= f(x) x = f 00 () < 0= f(x) x = A--2 [ ] f(x) D f 00 (x) > 0= y = f(x) f 00 (x) < 0= y = f(x) P (, f()) f 00 () =0 A--3 [ ] y = f(x) [, b] x = f (y)
A. A. A-- [ ] f(x) x = f 00 (x) f 0 () =0 f 00 () > 0= f(x) x = f 00 () < 0= f(x) x = A--2 [ ] f(x) D f 00 (x) > 0= y = f(x) f 00 (x) < 0= y = f(x) P (, f()) f 00 () =0 A--3 [ ] y = f(x) [, b] x = f (y)
1 θ i (1) A B θ ( ) A = B = sin 3θ = sin θ (A B sin 2 θ) ( ) 1 2 π 3 < = θ < = 2 π 3 Ax Bx3 = 1 2 θ = π sin θ (2) a b c θ sin 5θ = sin θ f(sin 2 θ) 2
 θ i ) AB θ ) A = B = sin θ = sin θ A B sin θ) ) < = θ < = Ax Bx = θ = sin θ ) abc θ sin 5θ = sin θ fsin θ) fx) = ax bx c ) cos 5 i sin 5 ) 5 ) αβ α iβ) 5 α 4 β α β β 5 ) a = b = c = ) fx) = 0 x x = x =
θ i ) AB θ ) A = B = sin θ = sin θ A B sin θ) ) < = θ < = Ax Bx = θ = sin θ ) abc θ sin 5θ = sin θ fsin θ) fx) = ax bx c ) cos 5 i sin 5 ) 5 ) αβ α iβ) 5 α 4 β α β β 5 ) a = b = c = ) fx) = 0 x x = x =
I, II 1, A = A 4 : 6 = max{ A, } A A 10 10%
 1 2006.4.17. A 3-312 tel: 092-726-4774, e-mail: hara@math.kyushu-u.ac.jp, http://www.math.kyushu-u.ac.jp/ hara/lectures/lectures-j.html Office hours: B A I ɛ-δ ɛ-δ 1. 2. A 1. 1. 2. 3. 4. 5. 2. ɛ-δ 1. ɛ-n
1 2006.4.17. A 3-312 tel: 092-726-4774, e-mail: hara@math.kyushu-u.ac.jp, http://www.math.kyushu-u.ac.jp/ hara/lectures/lectures-j.html Office hours: B A I ɛ-δ ɛ-δ 1. 2. A 1. 1. 2. 3. 4. 5. 2. ɛ-δ 1. ɛ-n
A
 A 2563 15 4 21 1 3 1.1................................................ 3 1.2............................................. 3 2 3 2.1......................................... 3 2.2............................................
A 2563 15 4 21 1 3 1.1................................................ 3 1.2............................................. 3 2 3 2.1......................................... 3 2.2............................................
 i I II I II II IC IIC I II ii 5 8 5 3 7 8 iii I 3........................... 5......................... 7........................... 4........................ 8.3......................... 33.4...................
i I II I II II IC IIC I II ii 5 8 5 3 7 8 iii I 3........................... 5......................... 7........................... 4........................ 8.3......................... 33.4...................
S I. dy fx x fx y fx + C 3 C dy fx 4 x, y dy v C xt y C v e kt k > xt yt gt [ v dt dt v e kt xt v e kt + C k x v + C C k xt v k 3 r r + dr e kt S dt d
 S I.. http://ayapin.film.s.dendai.ac.jp/~matuda /TeX/lecture.html PDF PS.................................... 3.3.................... 9.4................5.............. 3 5. Laplace................. 5....
S I.. http://ayapin.film.s.dendai.ac.jp/~matuda /TeX/lecture.html PDF PS.................................... 3.3.................... 9.4................5.............. 3 5. Laplace................. 5....
*3 i 9 (1,) i (i,) (1,) 9 (i,) i i 2 1 ( 1, ) (1,) 18 2 i, 2 i i r 3r + 4i 1 i 1 i *4 1 i 9 i 1 1 i i 3 9 +
 1 2 IT 1 *1 1 2 3 π i 1i 2i 3i πi i 2 1 *2 2 + 3 + 4i π ei 3 4 4 2 2 *1 *2 x 2 + 1 = x 2 + x + 1 = 2 3 1 2 2 2 2 *3 i 9 (1,) i (i,) (1,) 9 (i,) i i 2 1 ( 1, ) (1,) 18 2 i, 2 i 1 2 1 2 2 1 i r 3r + 4i 1
1 2 IT 1 *1 1 2 3 π i 1i 2i 3i πi i 2 1 *2 2 + 3 + 4i π ei 3 4 4 2 2 *1 *2 x 2 + 1 = x 2 + x + 1 = 2 3 1 2 2 2 2 *3 i 9 (1,) i (i,) (1,) 9 (i,) i i 2 1 ( 1, ) (1,) 18 2 i, 2 i 1 2 1 2 2 1 i r 3r + 4i 1
SAMA- SUKU-RU Contents p-adic families of Eisenstein series (modular form) Hecke Eisenstein Eisenstein p T
 SAMA- SUKU-RU Contents 1. 1 2. 7.1. p-adic families of Eisenstein series 3 2.1. modular form Hecke 3 2.2. Eisenstein 5 2.3. Eisenstein p 7 3. 7.2. The projection to the ordinary part 9 3.1. The ordinary
SAMA- SUKU-RU Contents 1. 1 2. 7.1. p-adic families of Eisenstein series 3 2.1. modular form Hecke 3 2.2. Eisenstein 5 2.3. Eisenstein p 7 3. 7.2. The projection to the ordinary part 9 3.1. The ordinary
() x + y + y + x dy dx = 0 () dy + xy = x dx y + x y ( 5) ( s55906) 0.7. (). 5 (). ( 6) ( s6590) 0.8 m n. 0.9 n n A. ( 6) ( s6590) f A (λ) = det(a λi)
 0. A A = 4 IC () det A () A () x + y + z = x y z X Y Z = A x y z ( 5) ( s5590) 0. a + b + c b c () a a + b + c c a b a + b + c 0 a b c () a 0 c b b c 0 a c b a 0 0. A A = 7 5 4 5 0 ( 5) ( s5590) () A ()
0. A A = 4 IC () det A () A () x + y + z = x y z X Y Z = A x y z ( 5) ( s5590) 0. a + b + c b c () a a + b + c c a b a + b + c 0 a b c () a 0 c b b c 0 a c b a 0 0. A A = 7 5 4 5 0 ( 5) ( s5590) () A ()
 1 B () Ver 2014 0 2014/10 2015/1 http://www-cr.scphys.kyoto-u.ac.jp/member/tsuru/lecture/... 1. ( ) 2. 3. 3 1 7 1.1..................................................... 7 1.2.............................................
1 B () Ver 2014 0 2014/10 2015/1 http://www-cr.scphys.kyoto-u.ac.jp/member/tsuru/lecture/... 1. ( ) 2. 3. 3 1 7 1.1..................................................... 7 1.2.............................................
solutionJIS.dvi
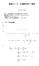 May 0, 006 6 morimune@econ.kyoto-u.ac.jp /9/005 (7 0/5/006 1 1.1 (a) (b) (c) c + c + + c = nc (x 1 x)+(x x)+ +(x n x) =(x 1 + x + + x n ) nx = nx nx =0 c(x 1 x)+c(x x)+ + c(x n x) =c (x i x) =0 y i (x
May 0, 006 6 morimune@econ.kyoto-u.ac.jp /9/005 (7 0/5/006 1 1.1 (a) (b) (c) c + c + + c = nc (x 1 x)+(x x)+ +(x n x) =(x 1 + x + + x n ) nx = nx nx =0 c(x 1 x)+c(x x)+ + c(x n x) =c (x i x) =0 y i (x
ad bc A A A = ad bc ( d ) b c a n A n A n A A det A A ( ) a b A = c d det A = ad bc σ {,,,, n} {,,, } {,,, } {,,, } ( ) σ = σ() = σ() = n sign σ sign(
 I n n A AX = I, YA = I () n XY A () X = IX = (YA)X = Y(AX) = YI = Y X Y () XY A A AB AB BA (AB)(B A ) = A(BB )A = AA = I (BA)(A B ) = B(AA )B = BB = I (AB) = B A (BA) = A B A B A = B = 5 5 A B AB BA A
I n n A AX = I, YA = I () n XY A () X = IX = (YA)X = Y(AX) = YI = Y X Y () XY A A AB AB BA (AB)(B A ) = A(BB )A = AA = I (BA)(A B ) = B(AA )B = BB = I (AB) = B A (BA) = A B A B A = B = 5 5 A B AB BA A
5.. z = f(x, y) y y = b f x x g(x) f(x, b) g x ( ) A = lim h 0 g(a + h) g(a) h g(x) a A = g (a) = f x (a, b)
 5 partial differentiation (total) differentiation 5. z = f(x, y) (a, b) A = lim h 0 f(a + h, b) f(a, b) h............................................................... ( ) f(x, y) (a, b) x A (a, b) x
5 partial differentiation (total) differentiation 5. z = f(x, y) (a, b) A = lim h 0 f(a + h, b) f(a, b) h............................................................... ( ) f(x, y) (a, b) x A (a, b) x
<4D F736F F D B B83578B6594BB2D834A836F815B82D082C88C60202E646F63>
 電気電子数学入門 サンプルページ この本の定価 判型などは, 以下の URL からご覧いただけます. http://www.morikita.co.jp/books/mid/073471 このサンプルページの内容は, 初版 1 刷発行当時のものです. i 14 (tool) [ ] IT ( ) PC (EXCEL) HP() 1 1 4 15 3 010 9 ii 1... 1 1.1 1 1.
電気電子数学入門 サンプルページ この本の定価 判型などは, 以下の URL からご覧いただけます. http://www.morikita.co.jp/books/mid/073471 このサンプルページの内容は, 初版 1 刷発行当時のものです. i 14 (tool) [ ] IT ( ) PC (EXCEL) HP() 1 1 4 15 3 010 9 ii 1... 1 1.1 1 1.
y π π O π x 9 s94.5 y dy dx. y = x + 3 y = x logx + 9 s9.6 z z x, z y. z = xy + y 3 z = sinx y 9 s x dx π x cos xdx 9 s93.8 a, fx = e x ax,. a =
 [ ] 9 IC. dx = 3x 4y dt dy dt = x y u xt = expλt u yt λ u u t = u u u + u = xt yt 6 3. u = x, y, z = x + y + z u u 9 s9 grad u ux, y, z = c c : grad u = u x i + u y j + u k i, j, k z x, y, z grad u v =
[ ] 9 IC. dx = 3x 4y dt dy dt = x y u xt = expλt u yt λ u u t = u u u + u = xt yt 6 3. u = x, y, z = x + y + z u u 9 s9 grad u ux, y, z = c c : grad u = u x i + u y j + u k i, j, k z x, y, z grad u v =
さくらの個別指導 ( さくら教育研究所 ) A a 1 a 2 a 3 a n {a n } a 1 a n n n 1 n n 0 a n = 1 n 1 n n O n {a n } n a n α {a n } α {a
 ... A a a a 3 a n {a n } a a n n 3 n n n 0 a n = n n n O 3 4 5 6 n {a n } n a n α {a n } α {a n } α α {a n } a n n a n α a n = α n n 0 n = 0 3 4. ()..0.00 + (0.) n () 0. 0.0 0.00 ( 0.) n 0 0 c c c c c
... A a a a 3 a n {a n } a a n n 3 n n n 0 a n = n n n O 3 4 5 6 n {a n } n a n α {a n } α {a n } α α {a n } a n n a n α a n = α n n 0 n = 0 3 4. ()..0.00 + (0.) n () 0. 0.0 0.00 ( 0.) n 0 0 c c c c c
 IA hara@math.kyushu-u.ac.jp Last updated: January,......................................................................................................................................................................................
IA hara@math.kyushu-u.ac.jp Last updated: January,......................................................................................................................................................................................
6 2 2 x y x y t P P = P t P = I P P P ( ) ( ) ,, ( ) ( ) cos θ sin θ cos θ sin θ, sin θ cos θ sin θ cos θ y x θ x θ P
 6 x x 6.1 t P P = P t P = I P P P 1 0 1 0,, 0 1 0 1 cos θ sin θ cos θ sin θ, sin θ cos θ sin θ cos θ x θ x θ P x P x, P ) = t P x)p ) = t x t P P ) = t x = x, ) 6.1) x = Figure 6.1 Px = x, P=, θ = θ P
6 x x 6.1 t P P = P t P = I P P P 1 0 1 0,, 0 1 0 1 cos θ sin θ cos θ sin θ, sin θ cos θ sin θ cos θ x θ x θ P x P x, P ) = t P x)p ) = t x t P P ) = t x = x, ) 6.1) x = Figure 6.1 Px = x, P=, θ = θ P
Part y mx + n mt + n m 1 mt n + n t m 2 t + mn 0 t m 0 n 18 y n n a 7 3 ; x α α 1 7α +t t 3 4α + 3t t x α x α y mx + n
 Part2 47 Example 161 93 1 T a a 2 M 1 a 1 T a 2 a Point 1 T L L L T T L L T L L L T T L L T detm a 1 aa 2 a 1 2 + 1 > 0 11 T T x x M λ 12 y y x y λ 2 a + 1λ + a 2 2a + 2 0 13 D D a + 1 2 4a 2 2a + 2 a
Part2 47 Example 161 93 1 T a a 2 M 1 a 1 T a 2 a Point 1 T L L L T T L L T L L L T T L L T detm a 1 aa 2 a 1 2 + 1 > 0 11 T T x x M λ 12 y y x y λ 2 a + 1λ + a 2 2a + 2 0 13 D D a + 1 2 4a 2 2a + 2 a
5.. z = f(x, y) y y = b f x x g(x) f(x, b) g x ( ) A = lim h g(a + h) g(a) h g(x) a A = g (a) = f x (a, b)............................................
 5 partial differentiation (total) differentiation 5. z = f(x, y) (a, b) A = lim h f(a + h, b) f(a, b) h........................................................... ( ) f(x, y) (a, b) x A (a, b) x (a, b)
5 partial differentiation (total) differentiation 5. z = f(x, y) (a, b) A = lim h f(a + h, b) f(a, b) h........................................................... ( ) f(x, y) (a, b) x A (a, b) x (a, b)
 (u(x)v(x)) = u (x)v(x) + u(x)v (x) ( ) u(x) = u (x)v(x) u(x)v (x) v(x) v(x) 2 y = g(t), t = f(x) y = g(f(x)) dy dx dy dx = dy dt dt dx., y, f, g y = f (g(x))g (x). ( (f(g(x)). ). [ ] y = e ax+b (a, b )
(u(x)v(x)) = u (x)v(x) + u(x)v (x) ( ) u(x) = u (x)v(x) u(x)v (x) v(x) v(x) 2 y = g(t), t = f(x) y = g(f(x)) dy dx dy dx = dy dt dt dx., y, f, g y = f (g(x))g (x). ( (f(g(x)). ). [ ] y = e ax+b (a, b )
3 3.1 R r r + R R r Rr [ ] ˆn(r) = ˆn(r + R) (3.1) R R = r ˆn(r) = ˆn(0) r 0 R = r C nn (r, r ) = C nn (r + R, r + R) = C nn (r r, 0) (3.2) ( 2.2 ) C
![3 3.1 R r r + R R r Rr [ ] ˆn(r) = ˆn(r + R) (3.1) R R = r ˆn(r) = ˆn(0) r 0 R = r C nn (r, r ) = C nn (r + R, r + R) = C nn (r r, 0) (3.2) ( 2.2 ) C 3 3.1 R r r + R R r Rr [ ] ˆn(r) = ˆn(r + R) (3.1) R R = r ˆn(r) = ˆn(0) r 0 R = r C nn (r, r ) = C nn (r + R, r + R) = C nn (r r, 0) (3.2) ( 2.2 ) C](/thumbs/94/119172213.jpg) 3 3.1 R r r + R R r Rr [ ] ˆn(r) = ˆn(r + R) (3.1) R R = r ˆn(r) = ˆn(0) r 0 R = r C nn (r, r ) = C nn (r + R, r + R) = C nn (r r, 0) (3.2) ( 2.2 ) C nn (r r ) = C nn (R(r r )) [2 ] 2 g(r, r ) ˆn(r) ˆn(r
3 3.1 R r r + R R r Rr [ ] ˆn(r) = ˆn(r + R) (3.1) R R = r ˆn(r) = ˆn(0) r 0 R = r C nn (r, r ) = C nn (r + R, r + R) = C nn (r r, 0) (3.2) ( 2.2 ) C nn (r r ) = C nn (R(r r )) [2 ] 2 g(r, r ) ˆn(r) ˆn(r
¥¢¥ë¥´¥ê¥º¥à¥¤¥ó¥È¥í¥À¥¯¥·¥ç¥ó ÎØ¹Ö #1
 #1 id:motemen August 27, 2008 id:motemen 1-3 1-5 6-9 10-14 1 2 : n < a 1, a 2,..., a n > a 1 a 2 a n < a 1, a 2,..., a n > : Google: insertion sort site:youtube.com 1 : procedure Insertion-Sort(A) for
#1 id:motemen August 27, 2008 id:motemen 1-3 1-5 6-9 10-14 1 2 : n < a 1, a 2,..., a n > a 1 a 2 a n < a 1, a 2,..., a n > : Google: insertion sort site:youtube.com 1 : procedure Insertion-Sort(A) for
ii 3.,. 4. F. (), ,,. 8.,. 1. (75%) (25%) =7 20, =7 21 (. ). 1.,, (). 3.,. 1. ().,.,.,.,.,. () (12 )., (), 0. 2., 1., 0,.
 24(2012) (1 C106) 4 11 (2 C206) 4 12 http://www.math.is.tohoku.ac.jp/~obata,.,,,.. 1. 2. 3. 4. 5. 6. 7.,,. 1., 2007 (). 2. P. G. Hoel, 1995. 3... 1... 2.,,. ii 3.,. 4. F. (),.. 5... 6.. 7.,,. 8.,. 1. (75%)
24(2012) (1 C106) 4 11 (2 C206) 4 12 http://www.math.is.tohoku.ac.jp/~obata,.,,,.. 1. 2. 3. 4. 5. 6. 7.,,. 1., 2007 (). 2. P. G. Hoel, 1995. 3... 1... 2.,,. ii 3.,. 4. F. (),.. 5... 6.. 7.,,. 8.,. 1. (75%)
f(x) = x (1) f (1) (2) f (2) f(x) x = a y y = f(x) f (a) y = f(x) A(a, f(a)) f(a + h) f(x) = A f(a) A x (3, 3) O a a + h x 1 f(x) x = a
 3 3.1 3.1.1 A f(a + h) f(a) f(x) lim f(x) x = a h 0 h f(x) x = a f 0 (a) f 0 (a) = lim h!0 f(a + h) f(a) h = lim x!a f(x) f(a) x a a + h = x h = x a h 0 x a 3.1 f(x) = x x = 3 f 0 (3) f (3) = lim h 0 (
3 3.1 3.1.1 A f(a + h) f(a) f(x) lim f(x) x = a h 0 h f(x) x = a f 0 (a) f 0 (a) = lim h!0 f(a + h) f(a) h = lim x!a f(x) f(a) x a a + h = x h = x a h 0 x a 3.1 f(x) = x x = 3 f 0 (3) f (3) = lim h 0 (
n Y 1 (x),..., Y n (x) 1 W (Y 1 (x),..., Y n (x)) 0 W (Y 1 (x),..., Y n (x)) = Y 1 (x)... Y n (x) Y 1(x)... Y n(x) (x)... Y n (n 1) (x) Y (n 1)
 D d dx 1 1.1 n d n y a 0 dx n + a d n 1 y 1 dx n 1 +... + a dy n 1 dx + a ny = f(x)...(1) dk y dx k = y (k) a 0 y (n) + a 1 y (n 1) +... + a n 1 y + a n y = f(x)...(2) (2) (2) f(x) 0 a 0 y (n) + a 1 y
D d dx 1 1.1 n d n y a 0 dx n + a d n 1 y 1 dx n 1 +... + a dy n 1 dx + a ny = f(x)...(1) dk y dx k = y (k) a 0 y (n) + a 1 y (n 1) +... + a n 1 y + a n y = f(x)...(2) (2) (2) f(x) 0 a 0 y (n) + a 1 y
( ) a, b c a 2 + b 2 = c 2. 2 1 2 2 : 2 2 = p q, p, q 2q 2 = p 2. p 2 p 2 2 2 q 2 p, q (QED)
 rational number p, p, (q ) q ratio 3.14 = 3 + 1 10 + 4 100 ( ) a, b c a 2 + b 2 = c 2. 2 1 2 2 : 2 2 = p q, p, q 2q 2 = p 2. p 2 p 2 2 2 q 2 p, q (QED) ( a) ( b) a > b > 0 a < nb n A A B B A A, B B A =
rational number p, p, (q ) q ratio 3.14 = 3 + 1 10 + 4 100 ( ) a, b c a 2 + b 2 = c 2. 2 1 2 2 : 2 2 = p q, p, q 2q 2 = p 2. p 2 p 2 2 2 q 2 p, q (QED) ( a) ( b) a > b > 0 a < nb n A A B B A A, B B A =
M3 x y f(x, y) (= x) (= y) x + y f(x, y) = x + y + *. f(x, y) π y f(x, y) x f(x + x, y) f(x, y) lim x x () f(x,y) x 3 -
 M3............................................................................................ 3.3................................................... 3 6........................................... 6..........................................
M3............................................................................................ 3.3................................................... 3 6........................................... 6..........................................






