(Stochastic Thermodynsmics) Langevin Langevin
|
|
|
- ひろみ きせんばる
- 5 years ago
- Views:
Transcription
1 6 8 5
2 (Stochastic Thermodynsmics) Langevin Langevin
3 Markov Master Liouville Poisson Gauss White (Lévy-Itô ) Gauss Multiplicative Poisson Taylor Gauss Gauss Stratonovich Fokker-Planck Onsager-Machlup Langevin Underdamped Langevin Overdamped Langevin Helmholtz Crooks A 3 A.1 Liouville (1.4) A. Multiplicative Gauss
4 B 5 B.1 Gauss B B..1 Langevin ( ) B
5 1 1 C. Gardiner 1], van Kampen ], H. Risken 3] H. Haken 4] Gauss Gauss Poisson Poisson Gauss Gauss Gauss 1.1 Markov 1 Markov Markov ˆx(t) P (x, t) P (ˆx(t) = x) L Master 3 P (x, t) = LP (x, t). (1.1) t 1. Master 1..1 Liouville P (ˆx(0) = x 0 ) P 0 (x 0 ) 4 : dt = a(ˆx), (1.3) Markov P (x, t) Liouville 5] A.1 P (x, t) = a(x)p (x, t). (1.4) t x 1 C. Gardiner1] 3 t P (x, t + t) 1Step (P (x, t)) L P (x, t + t) = L P (x, t) (1.) L = 1 + tl Master 4 ˆx(t) 3
6 (a) y * ^ δ( t t ) i (b) t^1 t^ t^3 1 t t^ t^ t^3 t 1.1: (a)poisson (b)poisson (a(x) = x ) 1.. Poisson Poisson Poisson 1 y λ t, t + dt] Poisson λdt + O(dt ) (1.5) {ˆt i } i ˆt i i y Poisson ( 1.1(a)) ˆξ P y,λ(t) = y δ(t ˆt i ). (1.6) i=1 Poisson dt = a(ˆx) + ˆξ P y,λ(t). (1.7) a(ˆx) = x 1.1(b) Jump Master P (x, t) = t x a(x)p (x, t) + λp (x y, t) P (x, t)] (1.8) Poisson Poisson Gauss Poisson a(x) = 0 dt ˆx(t + dt) x(t) 0 ( =1 λdt) = y ( =λdt) (1.9) () n 0 ( =1 λdt) = y n ( =λdt) (1.10) () n = λy n dt (1.11) 4
7 (a) (b) t^1 t^ t^3 1 t t^ t^ t^ 3 t 1.: (a) Poisson (b) Poisson (a(ˆx) = ˆx ) O(dt) 1 Poisson white. Poisson ( ) Poisson Poisson ˆξ y SP,λ(t) = ˆξ P y,λ/ (t) + ˆξ y P,λ/(t). (1.1) SP = a(ˆx) + ˆξ y dt,λ (1.13) Master P (x, t) = t x a(x)p (x, t) + λ P (x y, t) P (x, t)] + λ P (x + y, t) P (x, t)]. (1.14) Poisson : () n 0 (n ) =. (1.15) λy n dt (n ) 1..3 Gauss Poisson SP Poisson ˆξ y,λ Poisson = λy dt (1.16) (λy = σ ( )) y 0 (y +0) ˆξ G σ (t) lim ˆξ y SP,λ(t). (1.17) y λ=σ y +0 Gauss 1 Gauss ˆξ G λ +, y +0 5
8 (a) (b) t^1 t^ t^3 1 t t^ t^ t^ 3 t Gaussian limit (c) (d) t t 1.3: (a-b) Poisson a(ˆx) = ˆx (c-d) Poisson Gaussian (y +0, λy = 1) Gauss (c) Gauss (d) Gauss Master a(x) = 0 Poisson Master (1.14) Taylor t P (x, t) = λ P (x y, t) + P (x + y, t) P (x, t)] y n n = λ P (x, t). (1.18) (n)! xn n=1 λy = σ ( ) y +0 σ P (x, t) = P (x, t) (1.19) t x (1.19) Poisson Master Gauss 1 Gauss (ˆξ G (t) ˆξ σ G =1 (t)) : dŵ ˆξ G dt Ŵ (t) = t t i dsˆξ G (s). (1.0) Ŵ Wiener Gauss (Wiener ) dt (n = ) (dŵ )n =. (1.1) 0 ( ) 6
9 1..4 White (Lévy-Itô ) White White Lévy ˆξ(t 1 )ˆξ(t ) δ(t 1 t ). (1.) Poisson Gauss White White Lévy-Itô White Poisson Gauss White Markov 1..5 Gauss Gauss ( ) 1 Markov Gauss ( ) Gauss ( ) Gauss Gauss ( ) 5 Gauss Master (1.19) ( ) Gauss (1.1) (1.1) dt (n = ) (dŵ )n =. (1.3) 0 (n 3) Poisson Gauss 6 Ẑ (dŵ ) Ẑ 0 (dŵ ) (Ẑ Ẑ ) = Z Ẑ = (dŵ )4 dt = 0 + o(dt). (1.4) (dŵ (dŵ ) = dt lim ) = 1. (1.5) dt dt Gauss (dŵ ) 7 dtdŵ = 0. (1.6) 5 1] 6 Poisson (1.10) 7 Ẑ dtdŵ (Ẑ Ẑ ) = Z Ẑ = dt (dŵ ) = O(dt 3 ). 7
10 Multiplicative dt = a(ˆx) + ˆξ(t). (1.7) white additive Additive well-defined additive well-defined dt = a(ˆx) + b(ˆx)ˆξ. (1.8) multiplicative well-defined dx(t) = x(t)δ(t 1), (1.9) dt x(0) = 1 well-defined t = 1 t x(1+ t) = x i+1, x(1) = x i = 1 x i+1 x i t x i+1 x i t 1 = x i = x(1 + 0) =, (1.30) t = x i+1 + x i α (0 α 1 ) x i+1 x i t = αx i+1 + (1 α)x i ] 1 t 1 = x(1 + 0) = 3, (1.31) t = x(1 + 0) = α 1 α. (1.3) δ white δ 1.3. (1.30) t i, t f ] (t i = s 0 < s < < s N = t f ) t i t i+1 t i t max i t i N, t +0 tf t i t +0 N dsf(ˆx(s)) ˆξ(s) lim N 1 i=0 t i f(ˆx(s i ))ˆξ(s i ) (1.33) 8
11 multiplicative ˆx(t) = ˆx(t i ) + dt = a(ˆx) + b(ˆx) ˆξ (1.34) tf t i dsa(ˆx(s)) + tf t i dsb(ˆx(s)) ˆξ(s). (1.35) tf tf ds f(ˆx(s)) ˆξ(s) = ds f(ˆx(s)) ˆξ(s) = 0 t i t i f(ˆx(s)) ˆξ(s) = f(ˆx(s)) ˆξ(s) = 0. (1.36) (1.33) f(ˆx(s i ))ˆξ(s i ) ˆx(s i ) ˆξ G (s i ) decouple ˆξ G (s i ) ˆx(s i+1 ) 1.4 δ White (1.) White Poisson δ ŷ = f(ˆx), (1.37) f(x) Poisson Taylor Poisson (ˆξ P y,λ (t) = i=1 y δ(t ˆt i )) dt = a(ˆx) + b(ˆx) ˆξ P y,λ. (1.38) ˆt 0 = t i Poisson t = ˆt i ˆt i < t < ˆt i+1 y (t = ˆt i ) ˆx(ˆt i + dt) ˆx(ˆt i ) =. (1.39) a(ˆx)dt (ˆt i < t < ˆt i+1 ) ŷ = f(ˆx) f(ˆx(t)) f(ˆx(ˆt i ) + y ) f(ˆx(ˆt i )) (t = ˆt i ) f(ˆx(t + dt)) f(ˆx(t)) = (1.40) f (ˆx(t))a(ˆx)dt (ˆt i < t < ˆt i+1 ) 9
12 y t = ˆt i Taylor df(ˆx) = () n n=1 n! df n (ˆx) n df(ˆx) dt = n=1 1 () n df n (ˆx) n! dt n, (1.41) 1.4. Gauss Gauss dt = a(ˆx) + b(ˆx) ˆξ G. (1.4) Gauss 0 Gauss Gauss Wiener dŵ ˆξ G dt = a(ˆx)dt + b(ˆx) dŵ (1.43) Taylor df(ˆx) = n=1 = df(ˆx) 1 df n (ˆx) ( ) n n! n a(ˆx)dt + b(ˆx) dŵ (1.44) ( ) a(ˆx)dt + b(ˆx) dŵ + n= 1 df n (ˆx) ( ) n n! n bn (ˆx) dŵ, (1.45) dtdŵ = 0 Gauss (1.5) df(ˆx) df(ˆx) = a(ˆx) + 1 ] b (ˆx) d f(ˆx) dt + b(ˆx) dŵ df(ˆx) = df(ˆx) df(ˆx) dt = df(ˆx) + 1 b (ˆx) d f(ˆx) dt dt + 1 b (ˆx) d f(ˆx). (1.46) (1.46) (1.5) Remark: Additive Gauss 1 additive (b(ˆx) = c = const.) df(ˆx) = df(ˆx) a(ˆx) + c d ] f(ˆx) dt + cdŵ (1.47) Additive 10
13 1.4.3 Gauss Stratonovich (1.41) (1.46) df/dt = (df/dx)(dx/dt) Jump Taylor Gauss (1.46) Jump Taylor 1 Gauss Stratonovich Additive Gauss (b(ˆx) = c = const.) 8 Multiplicative Gauss Appendix A. Stratonovich (1.31) tf t i t +0 N dsˆξ G (s) f(ˆx(s)) lim N 1 i=0 t i ˆξ(si ) f(ˆx(s i+1)) + f(ˆx(s i )). (1.48) ˆx(t) (1.4) Stratonovich tf t i dsˆξ G (s) f(ˆx(s)) = dsˆξ G f(ˆx) = dŵ tf f(ˆx(s + dt)) + f(ˆx(s)) t i dsˆξ G (s) f(ˆx(s)) + c tf dŵ (s) = dŵ f(ˆx) + (f(ˆx(s + dt)) f(ˆx(s))) = dsˆξ G f(ˆx) + dŵ df(ˆx(s)) = dsˆξ G f(ˆx) + dŵ = dsˆξ G f(ˆx) + c (dŵ ) = dt dtdŵ = 0 df(ˆx) (a(ˆx)dt + cdŵ ) + c t i ds df(ˆx). (1.49) d ] f(ˆx) dt df(ˆx) dt (1.50) Stratonovich Stratonovich df(ˆx) = df(ˆx) df(ˆx) = df(ˆx) df(ˆx) dt (1.50) = df(ˆx) dx dŵ df(ˆx) dx 8 = df(ˆx) (a(ˆx)dt + cdŵ ) dt. (1.51) df(ˆx) = dsŵ + c d f(ˆx) dt (b(ˆx) dŵ ) = dsŵ b(ˆx)f(ˆx) + 1 b (ˆx) d f(ˆx) dt (1.5) 11
14 (1.46) df(ˆx) = df(ˆx) a(ˆx) + c d ] f(ˆx) dt + cdŵ = df(ˆx) a(ˆx)dt + cdf(ˆx) dx dŵ = df(ˆx). (1.53) 1.5 Fokker-Planck Fokker-Planck dt = a(ˆx) + b(ˆx) ˆξ G (t) (1.54) P (x, t) P (ˆx(t) = x) P (x, t) = t x a(x) + 1 ] x b (x) P (x, t) (1.55) f(x) df(ˆx) df(ˆx) = dt a(ˆx) + 1 ] b (ˆx) d f(ˆx) f G + b(ˆx) ˆξ x df(x) dxp (x, t)f(x) = dxp (x, t) t dx a(x) + 1 ] b (x) d f(x) dx dx t P (x, t)f(x) = dxf(x) x a(x) + 1 ] x b (x) P (x, t), (1.56) (1.56) f(x) (1.55) 1.6 Onsager-Machlup dt = a(ˆx) + b(x) ˆξ G (1.57) Fokker-Planck (1.57) Fokker-Planck P (x, t) t = LP (x, t), L = x a(x) + 1 x b (x). (1.58) P (x, t + t) = (1 + tl)p (x, t) + O( t ) (1.59) t y t + t x t 1
15 P (x, t y, t) = δ(x y) P (x, t + t y, t) ( P (x, t + t y, t) = 1 + t x a(y) + 1 ]) x b (y) δ(x y) ] = e t x a(y)+ 1 x b (y) ds = = ds π exp is(x y) + t 1 πb (y) t exp π eis(x y) ( isa(y) b (y)s )] ] (x y a(y) t) b. (1.60) (y) t x y = v t ] 1 P (x, t + t y, t) = πb (y) t exp (v a(y)) b t (y) (1.61) P x] = N 1 lim t +0 = lim t +0 i=0 ( N 1 i=0 1 πb (x i ) t exp (v i a(x i )) b (x i ) ) N 1 1 exp πb (x i ) t i=0 ] t t (v i a(x i )) b (x i ) ], (1.6) v i t = x i+1 x i Onsager-Machlup Onsager-Machlup N 1 i=0 t (v i a(x i )) b (x i ) = dt (v(t) a(x(t))) b. (1.63) (x(t)) Onsager-Machlup v i x i Stratonovich 13
16 6].1 (.1(a) ).1(a) (U U ) W W (.1(b) ) (U U ) (.1(b) ) W (a) U U (b) U U T T T T.1: 14
17 T bead Laser trap T U U.: Langevin 7]. Langevin. Langevin M dˆv dt = γˆv + ˆξ T U(ˆx; a), ˆx dt = ˆv, (.1) M γ ˆξ T U 1 a e.g., a a :a = a(t). ˆξ T Gauss ˆξ T (t 1 )ˆξ T (t ) = γk B T δ(t 1 t ). (.) ˆξ T (t) = γk B T ˆξ G (t) (.1) M(dˆv/dt) Underdamped Langevin τ i M/γ Overdamped Langevin γ dt = U(ˆx; a) ˆx + ˆξ T. (.3) Langevin (.1) (.3) ˆξ T (t 1 )ˆξ T (t ) = γk B T δ(t 1 t ) ˆξ T 9] 1 15
18 Langevin P C (x, v) = 1 Mv e β( +U(x;a)), (.4) Z β 1/k B T Z(a) dxdv exp β(mv / + U) Langevin (.1) Fokker-Planck (.4) f(ˆx, ˆv) = t df(ˆx, ˆv) = f f + ˆx ˆv dˆv + 1 γk B T f M ˆv dt f dxdvf(x, v)p (x, v) = dxdv x p + f ( γv v M 1 U M x P (x, v) dxdvf(x, v) = dxdvf(x, v) t x v + ( γv v M + 1 M ) + γk BT U x M ] f v P (x, v) ) + γk BT M v ] P (x, v) f Kramers P (x, v) t = (.4) (.5) x v + 1 ( γv + U ) + γk BT ] M v x M v P (x, v). (.6) Overdamped Langevin Fokker-Planck P (x) = 1 U t γ x x + k BT ] P (x). (.7) x Z (a) dxe βu. P C (x) = 1 Z e βu(x;a). (.8) Langevin.3(a) Johnson-Nyquist 1].3(a) R L q U(q) T Underdamped Langevin L dˆq dˆq = R dt dt + ˆξ T U(ˆq), (.9) ˆq 16
19 (a) R : Resistance Capacitor (b) + q - q +q A d - ε 0 εn( q ) q l L : Reactance l *.3: Langevin Langevin 8] Johnson-Nyquist ˆξ T ˆξ T (t 1 )ˆξ T (t ) = Rk B T δ(t 1 t ). (.10) L Overdamped Langevin U(q) = Sϵ 0q d (.11) d S ϵ 0 (.3(b)) d ϵ N (q) ( l ) (.3(b)).3 Langevin.3.1 Underdamped Langevin Underdamped Langevin M dˆv dt = γv + ˆξ U(x; a) T, x (.1) = ˆv. dt (.13) Ê M ˆv 17 + U(ˆx, a). (.14)
20 Langevin a dŵ dŵ Ê a U(ˆx; a) da = da. (.15) a d ˆQ d ˆQ dê dŵ. (.16) d ˆQ ( ) d ˆQ M ˆv = d + U(ˆx; a) U(ˆx; a) da a U(ˆx; a) = M ˆv dˆv + ˆx = ( γˆv + ˆξ ) T. (.17) Stratonovich U(ˆx; a) = 1 a ( ) ˆx. (.19) a (.3).3(b) U(ˆq; d, l) = Sq d (l l)ε 0 + lε N (q) l. (.0) d d ˆQ = ( γv + ˆξ T ) + γk BT dt. (.18) M 18
21 .3. Overdamped Langevin Overdamped Langevin dŵ U(ˆx; a) = da, a γ dt = U(ˆx; a) ˆx + ˆξ T (.1) du(ˆx; a) = dŵ +d ˆQ (.) d ˆQ = U(ˆx) ˆx = ( γˆv + ˆξ T ). (.3).3.3 Helmholtz Helmholtz dw qs = dxdv U a P C(x, v)da ( )] dxdv U a β exp Mv + U(x; a) = ( dxdv exp β Mv )] da + U(x; a) ( )] Mv = k B T d log dxdv exp β + U(x; a) (.4) W = F (a f ) F (a i ), F (a) = k B T log Z(a). (.5).4 Ŵ ˆQ W F (.6) ( ).4.1 Langevin log P x, v x 0, v 0 ] P x, v x 0, = βqx, v]. (.7) v 0 ] x(t), v(t) 0, T ] x (t) x(t t), v (t) v(t t) Langevin 19
22 3 Underdamped Langevin (.1) Kramers (.6) Kramers t (y, u) t + t (x, v) P (x, v, t + t y, u, t) U U/x P (x, v, t y, u, t) = δ(x y)δ(v u) P (x, v, t + t y, u, t) = 1 + t x v + 1 M = 1 + t = exp t = = dsdw (π) exp πm γk B T t exp x u + 1 M v (γv + U (x)) + γk BT M v v (γu + U (y)) + γk BT M v v (γu + U (y)) + γk BT M v x u + 1 M is(x y) + iw(v u) + t M 4γk B T t (v u + γu + U ]] δ(x y)δ(v u) ]] δ(x y)δ(v u) ]] dsdw (π) eis(x y)+iw(v u) ( isu + iw M (γu + U (y)) w γk B T M M )] ) ] t δ(x y u t). (.8) v i v i+1 v i x i x i+1 x i N 1 πm P x, v] = lim t 0 γk B T t exp M ( t vi 4γk B T t + γv i + U ) ] (x i ) δ( x i v i t), (.9) M i=0 x i = x(i t), v i = v(i t), t T/N v i = v (i t) = v(t i t) = v N i x i = x (i t) = x(t i t) = x N i log P x, v x 0, v 0 ] P x, v x 0, v 0 ] N 1 = lim M ( t vi+1 v i t 0 4γk i=0 B T t N 1 = lim M ( t vi+1 v i t 0 4γk i=0 B T t N 1 = lim M ( t vi+1 v i t 0 4γk B T t = lim t 0 = = i=0 N 1 i=0 T 0 T 0 t ( k B T v i M v i ds 1 k B T v ( M dv ds + U (x) + γv i + U ) ( (x i ) + M t v i+1 v i M 4γk B T t ) + γv i + U (x i ) M + γv i + U ) (x i ) + M ( t v N i 1 v N i + γv N i + U ) ] (x N i ) M 4γk B T t M + γv i + U ) (x i ) + M ( t vi+1 v i + γv i+1 + U ) ] (x i+1 ) M 4γk B T t M )] t + U (x i ) ) 1 ( γv + ξ) dx(s) = βqx, v] (.30) k B T 3 0
23 4 i N 1 i 5 O( t) 5 x i v i 4 Langevin Qx, v] Q Qx, v ] = T = = 0 T ( dsv (s) M dv (s) ds 0 T 0 dsv(t s) ) + U (x (s)) ( d ds v(t s) + U (x(t s)) ( dv(s ds v(s ) ) ) ds + U (x(s )) = Qx, v] (.31) ).4. Crooks P x, v x 0, v 0 ] = P x, v; x 0, v 0 ] P 0 (x 0, v 0 ) P x, v] P 0 (x 0, v 0 ) (.3) (.7) log P x, v] P x, v = σx, v], (.33) ] (Entropy production) σx, v] βqx, v] + log P 0 (x 0, v 0 ) log P 0 (x 0, v 0 ) (.34) Crooks 10] log P (+σ) P = σ, (.35) ( σ) P (σ) DxDvδ(σ σx, v])p x, v], P (σ) Dx Dv δ(σ σx, v ])P x, v ] (.36) (.33) P x, v]e σx,v] = P x, v ] DxDvP x, v]e σx,v] δ(σ σx, v]) = DxDvP x, v ]δ(σ σx, v]) P (+σ)e σ = Dx Dv P x, v ]δ(σ + σx, v ]) = P ( σ), (.37) DxDv = Dx Dv σx, v] = σx, v ] 4 v ξ t x 1
24 .4.3 log P SS(+σ) = σ. (.38) P SS ( σ) e ˆσ = 1 (.39) Jensen e x 1 + x ˆσ 0. (.40) Jarzynski E(x, v) = Mv / + U(x; a) P 0 (x 0, v 0 ) = e β(f E(x 0,v 0 )), F k B T log dx 0 dv 0 e βe(x 0,v 0 ). (.41) Jarzynski 11] σ = β ( Qx, v] F + E) = β(w x, v] F ) (.4) e βw = e β F. (.43) Jensen ( ) Ŵ F (.44)
25 A A.1 Liouville (1.4) Liouville (1.4) ˆx(0) = x 0. P (x, 0) = δ(x x 0 ) t P (x, t) = δ(x Xt; x 0 ]). Xt; x 0 ] ˆx(0) = x 0 (1.3) δ t δ(x Xt; x 0]) = dxt; x 0] dt X δ(x Xt; x 0]) = a(xt; x 0 ]) x δ(x Xt; x 0]) = x a(xt; x 0])δ(x Xt; x 0 ]) = x a(x)δ(x Xt; x 0]), (A.1) dxt; x 0 ] dt = a(xt; x 0 ]), δ(x X) = δ(x X). x X (A.) P 0 (x 0 ) (A.1) P (x(t) = x x(0) = x 0 ) P (x, t x 0 ) t P (x, t x 0) = x a(x)p (x, t x 0). (A.3) P (x, t) dx 0 P (x, t x 0 )P 0 (x 0 ) (A.3) (1.4) A. Multiplicative Gauss Stratonovich (1.31) tf t i t +0 N dsˆξ G (s) f(ˆx(s)) lim N 1 i=0 t i ˆξ(si ) f(ˆx(s i+1)) + f(ˆx(s i )). (A.4) ˆx(t) (1.4) Stratonovich tf t i dsˆξ G (s) f(ˆx(s)) = tf t i dsˆξ G (s) f(ˆx(s)) + 1 tf t i dsb(ˆx(s)) df(ˆx). (A.5) 3
26 dsˆξ G f(ˆx) = dŵ f(ˆx(s + dt)) + f(ˆx(s)) dŵ (s) = dŵ f(ˆx) + (f(ˆx(s + dt)) f(ˆx(s))) = dsˆξ G f(ˆx) + dŵ df(ˆx(s)) df(ˆx) = dsˆξ G f(ˆx) + dŵ = dsˆξ G f(ˆx) + 1 b(ˆx)df(ˆx) dt (a(ˆx)dt + b(ˆx) dŵ ) + b (ˆx) d ] f(ˆx) dt (A.6) (dŵ ) = dt dtdŵ = 0 Stratonovich Stratonovich df(ˆx) = df(ˆx) df(ˆx) = df(ˆx) df(ˆx) dt (A.6) = df(ˆx) (a(ˆx)dt + b(ˆx) dŵ ) dt. (A.7) = df(ˆx) dx (1.46) df(ˆx) = dŵ df(ˆx) dx df(ˆx) = dsŵ + 1 f(ˆx) b(ˆx)d dt (b(ˆx) dŵ ) = dsŵ b(ˆx)f(ˆx) + 1 b (ˆx) d f(ˆx) dt (A.8) df(ˆx) a(ˆx) + 1 ] b (ˆx) d f(ˆx) dt + b(ˆx) dŵ = df(ˆx) df(ˆx) a(ˆx)dt + (b(ˆx) dŵ ) dx = df(ˆx). (A.9) 4
27 B B.1 Gauss dt = a(ˆx) + b(ˆx) ˆξ G. (B.1) Gauss dŵ dtˆξ G (dŵ ) = dt, (dŵ )n = 0, dtdŵ = 0. (B.) ŷ = f(ˆx) df(ˆx) = f(ˆx) ˆx Stratonovich dx + 1 b (ˆx) f(ˆx) ˆx dt. (B.3) df(ˆx) = f(ˆx) ˆx dx. (B.4) Fokker-Planck P (x, t) = t x a(x) + 1 ] x b (x) P (x, t). (B.5) a, b Onsager-Machlup P x] = lim t +0 ( N 1 i=0 ) N 1 1 exp πb (x i ) t i=0 ] t (v i a(x i )) b. (B.6) (x i ) 5
28 B. B..1 Langevin ( ) Langevin M dˆv dt = γv + ˆξ U(x; a) T, x dt = ˆv, Ê M ˆv U(ˆx; a) + U(ˆx, a), dŵ da, d a ˆQ ( γˆv + ˆξ ) T. dê = dŵ + d ˆQ. (B.9) (B.7) (B.8) ˆσ β ˆQ log P 0 (ˆx 0, ˆv 0 ) + log P 0 (ˆx 0, ˆv 0 ). ˆσ 0. (B.10) (B.11) Ŵ F, (B.1) F (a) k B T log dxdve βe(x,v,a) F F (a f ) F (a i ) B.. log P x, v x 0, v 0 ] P x, v x 0, = βqx, v]. v 0 ] (B.13) Crooks log P (+σ) P ( σ) = σ, (B.14) e ˆσ = 1 (B.15) Jarzynski Jarzynski e βw = e β F. (B.16) 6
29 1] C. Gardiner, Stochastic Methods (Springer-Verlag, Berlin, 009), 4th ed. ] N. G. Van Kampen, Stochastic Processes in Physics and Chemistry (North-Holland, Amsterdam, 007), 3rd ed. 3] H. Risken, The Fokker-Planck Equation (Springer, Berlin, 1989), nd ed. 4] H. Haken ( ), (1976). 5] L. D. Landau and E. M. Lishitz, Course of theoretical physics, vol. 1, Mechanics (Oxford: Butterworth-Heinemann, 1976). 6] (004); K. Sekimoto, Stochastic Energetics, (Springer, Berlin, 010). 7] D. M. Carberry, J. C. Reid, G. M. Wang, E. M. Sevick, D. J. Searles, and D. J. Evans, Phys. Rev. Lett. 9, (004); E. H. Trepagnier, C. Jarzynski, F. Ritort, G. E. Crooks, C. Bustamante, and J. Liphardt, Proc. Natl. Acad. Sci. U.S.A. 101, (004); V. Blickle, T. Speck, L. Helden, U. Seifert, and C. Bechinger, Phys. Rev. Lett. 96, (006). 8] R. van Zon, S. Ciliberto, and E. G. D. Cohen, Phys. Rev. Lett. 9, (004); N. Garnier and S. Ciliberto, Phys. Rev. E 71, (R) (005); S. Ciliberto, A. Imparato, A. Naert, and M. Tanase, Phys. Rev. Lett. 110, (013); K. Kanazawa, T. Sagawa, and H. Hayakawa, Phys. Rev. E 90, (014). 9] et al., (011); M. Toda, R. Kubo et al., Statistical Physics II: Nonequilibrium Statistical Mechanics, (Springer, Berlin, 013), nd ed. 10] G. E. Crooks, Phys. Rev. E 60, 71 (1999). 11] C. Jarzynski, Phys. Rev. Lett. 78, 690 (1997). 7
2011 8 26 3 I 5 1 7 1.1 Markov................................ 7 2 Gau 13 2.1.................................. 13 2.2............................... 18 2.3............................ 23 3 Gau (Le vy
untitled
3 3. (stochastic differential equations) { dx(t) =f(t, X)dt + G(t, X)dW (t), t [,T], (3.) X( )=X X(t) : [,T] R d (d ) f(t, X) : [,T] R d R d (drift term) G(t, X) : [,T] R d R d m (diffusion term) W (t)
i
009 I 1 8 5 i 0 1 0.1..................................... 1 0.................................................. 1 0.3................................. 0.4........................................... 3
http://www.ns.kogakuin.ac.jp/~ft13389/lecture/physics1a2b/ pdf I 1 1 1.1 ( ) 1. 30 m µm 2. 20 cm km 3. 10 m 2 cm 2 4. 5 cm 3 km 3 5. 1 6. 1 7. 1 1.2 ( ) 1. 1 m + 10 cm 2. 1 hr + 6400 sec 3. 3.0 10 5 kg
201711grade1ouyou.pdf
2017 11 26 1 2 52 3 12 13 22 23 32 33 42 3 5 3 4 90 5 6 A 1 2 Web Web 3 4 1 2... 5 6 7 7 44 8 9 1 2 3 1 p p >2 2 A 1 2 0.6 0.4 0.52... (a) 0.6 0.4...... B 1 2 0.8-0.2 0.52..... (b) 0.6 0.52.... 1 A B 2
() Remrk I = [0, ] [x i, x i ]. (x : ) f(x) = 0 (x : ) ξ i, (f) = f(ξ i )(x i x i ) = (x i x i ) = ξ i, (f) = f(ξ i )(x i x i ) = 0 (f) 0.
![() Remrk I = [0, ] [x i, x i ]. (x : ) f(x) = 0 (x : ) ξ i, (f) = f(ξ i )(x i x i ) = (x i x i ) = ξ i, (f) = f(ξ i )(x i x i ) = 0 (f) 0.](/thumbs/89/98384840.jpg "() Remrk I = [0, ] [x i, x i ]. (x : ) f(x) = 0 (x : ) ξ i, (f) = f(ξ i )(x i x i ) = (x i x i ) = ξ i, (f) = f(ξ i )(x i x i ) = 0 (f) 0.")
() 6 f(x) [, b] 6. Riemnn [, b] f(x) S f(x) [, b] (Riemnn) = x 0 < x < x < < x n = b. I = [, b] = {x,, x n } mx(x i x i ) =. i [x i, x i ] ξ i n (f) = f(ξ i )(x i x i ) i=. (ξ i ) (f) 0( ), ξ i, S, ε >
2 1 x 2 x 2 = RT 3πηaN A t (1.2) R/N A N A N A = N A m n(z) = n exp ( ) m gz k B T (1.3) z n z = m = m ρgv k B = erg K 1 R =
1 1 1.1 1827 *1 195 *2 x 2 t x 2 = 2Dt D RT D = RT N A 1 6πaη (1.1) D N A a η 198 *3 ( a =.212µ) *1 Robert Brown (1773-1858. *2 Albert Einstein (1879-1955 *3 Jean Baptiste Perrin (187-1942 2 1 x 2 x 2
(1.2) T D = 0 T = D = 30 kn 1.2 (1.4) 2F W = 0 F = W/2 = 300 kn/2 = 150 kn 1.3 (1.9) R = W 1 + W 2 = = 1100 N. (1.9) W 2 b W 1 a = 0
1 1 1.1 1.) T D = T = D = kn 1. 1.4) F W = F = W/ = kn/ = 15 kn 1. 1.9) R = W 1 + W = 6 + 5 = 11 N. 1.9) W b W 1 a = a = W /W 1 )b = 5/6) = 5 cm 1.4 AB AC P 1, P x, y x, y y x 1.4.) P sin 6 + P 1 sin 45
() x + y + y + x dy dx = 0 () dy + xy = x dx y + x y ( 5) ( s55906) 0.7. (). 5 (). ( 6) ( s6590) 0.8 m n. 0.9 n n A. ( 6) ( s6590) f A (λ) = det(a λi)
0. A A = 4 IC () det A () A () x + y + z = x y z X Y Z = A x y z ( 5) ( s5590) 0. a + b + c b c () a a + b + c c a b a + b + c 0 a b c () a 0 c b b c 0 a c b a 0 0. A A = 7 5 4 5 0 ( 5) ( s5590) () A ()
IA hara@math.kyushu-u.ac.jp Last updated: January,......................................................................................................................................................................................
(3) (2),,. ( 20) ( s200103) 0.7 x C,, x 2 + y 2 + ax = 0 a.. D,. D, y C, C (x, y) (y 0) C m. (2) D y = y(x) (x ± y 0), (x, y) D, m, m = 1., D. (x 2 y
[ ] 7 0.1 2 2 + y = t sin t IC ( 9) ( s090101) 0.2 y = d2 y 2, y = x 3 y + y 2 = 0 (2) y + 2y 3y = e 2x 0.3 1 ( y ) = f x C u = y x ( 15) ( s150102) [ ] y/x du x = Cexp f(u) u (2) x y = xey/x ( 16) ( s160101)
Grushin 2MA16039T
Grushin 2MA1639T 3 2 2 R d Borel α i k (x, bi (x, 1 i d, 1 k N d N α R d b α = α(x := (αk(x i 1 i d, 1 k N b = b(x := (b i (x 1 i d X = (X t t x R d dx t = α(x t db t + b(x t dt ( 3 u t = Au + V u, u(,
ii 3.,. 4. F. (), ,,. 8.,. 1. (75%) (25%) =7 20, =7 21 (. ). 1.,, (). 3.,. 1. ().,.,.,.,.,. () (12 )., (), 0. 2., 1., 0,.
24(2012) (1 C106) 4 11 (2 C206) 4 12 http://www.math.is.tohoku.ac.jp/~obata,.,,,.. 1. 2. 3. 4. 5. 6. 7.,,. 1., 2007 (). 2. P. G. Hoel, 1995. 3... 1... 2.,,. ii 3.,. 4. F. (),.. 5... 6.. 7.,,. 8.,. 1. (75%)
1 1.1 ( ). z = a + bi, a, b R 0 a, b 0 a 2 + b 2 0 z = a + bi = ( ) a 2 + b 2 a a 2 + b + b 2 a 2 + b i 2 r = a 2 + b 2 θ cos θ = a a 2 + b 2, sin θ =
1 1.1 ( ). z = + bi,, b R 0, b 0 2 + b 2 0 z = + bi = ( ) 2 + b 2 2 + b + b 2 2 + b i 2 r = 2 + b 2 θ cos θ = 2 + b 2, sin θ = b 2 + b 2 2π z = r(cos θ + i sin θ) 1.2 (, ). 1. < 2. > 3. ±,, 1.3 ( ). A
K E N Z OU
K E N Z OU 11 1 1 1.1..................................... 1.1.1............................ 1.1..................................................................................... 4 1.........................................
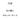23 7 28 i i 1 1 1.1................................... 2 1.2............................... 3 1.2.1.................................... 3 1.2.2............................... 4 1.2.3 SI..............................
Black-Scholes [1] Nelson [2] Schrödinger 1 Black Scholes [1] Black-Scholes Nelson [2][3][4] Schrödinger Nelson Parisi Wu [5] Nelson Parisi-W
![Black-Scholes [1] Nelson [2] Schrödinger 1 Black Scholes [1] Black-Scholes Nelson [2][3][4] Schrödinger Nelson Parisi Wu [5] Nelson Parisi-W](/thumbs/97/132191371.jpg "Black-Scholes [1] Nelson [2] Schrödinger 1 Black Scholes [1] Black-Scholes Nelson [2][3][4] Schrödinger Nelson Parisi Wu [5] Nelson Parisi-W")
003 7 14 Black-Scholes [1] Nelson [] Schrödinger 1 Black Scholes [1] Black-Scholes Nelson [][3][4] Schrödinger Nelson Parisi Wu [5] Nelson Parisi-Wu Nelson e-mail: takatoshi-tasaki@nifty.com kabutaro@mocha.freemail.ne.jp
43433 8 3 . Stochastic exponentials...................................... 3. Girsanov s theorem......................................... 4 On the martingale property of stochastic exponentials 5. Gronwall
( ) Loewner SLE 13 February
( ) Loewner SLE 3 February 00 G. F. Lawler, Conformally Invariant Processes in the Plane, (American Mathematical Society, 005)., Summer School 009 (009 8 7-9 ) . d- (BES d ) d B t = (Bt, B t,, Bd t ) (d
I ( ) 1 de Broglie 1 (de Broglie) p λ k h Planck ( Js) p = h λ = k (1) h 2π : Dirac k B Boltzmann ( J/K) T U = 3 2 k BT
I (008 4 0 de Broglie (de Broglie p λ k h Planck ( 6.63 0 34 Js p = h λ = k ( h π : Dirac k B Boltzmann (.38 0 3 J/K T U = 3 k BT ( = λ m k B T h m = 0.067m 0 m 0 = 9. 0 3 kg GaAs( a T = 300 K 3 fg 07345
Feynman Encounter with Mathematics 52, [1] N. Kumano-go, Feynman path integrals as analysis on path space by time slicing approximation. Bull
![Feynman Encounter with Mathematics 52, [1] N. Kumano-go, Feynman path integrals as analysis on path space by time slicing approximation. Bull](/thumbs/94/118365979.jpg "Feynman Encounter with Mathematics 52, [1] N. Kumano-go, Feynman path integrals as analysis on path space by time slicing approximation. Bull")
Feynman Encounter with Mathematics 52, 200 9 [] N. Kumano-go, Feynman path integrals as analysis on path space by time slicing approximation. Bull. Sci. Math. vol. 28 (2004) 97 25. [2] D. Fujiwara and
4. ϵ(ν, T ) = c 4 u(ν, T ) ϵ(ν, T ) T ν π4 Planck dx = 0 e x 1 15 U(T ) x 3 U(T ) = σt 4 Stefan-Boltzmann σ 2π5 k 4 15c 2 h 3 = W m 2 K 4 5.
A 1. Boltzmann Planck u(ν, T )dν = 8πh ν 3 c 3 kt 1 dν h 6.63 10 34 J s Planck k 1.38 10 23 J K 1 Boltzmann u(ν, T ) T ν e hν c = 3 10 8 m s 1 2. Planck λ = c/ν Rayleigh-Jeans u(ν, T )dν = 8πν2 kt dν c
untitled
2 : n =1, 2,, 10000 0.5125 0.51 0.5075 0.505 0.5025 0.5 0.4975 0.495 0 2000 4000 6000 8000 10000 2 weak law of large numbers 1. X 1,X 2,,X n 2. µ = E(X i ),i=1, 2,,n 3. σi 2 = V (X i ) σ 2,i=1, 2,,n ɛ>0
( ) ) ) ) 5) 1 J = σe 2 6) ) 9) 1955 Statistical-Mechanical Theory of Irreversible Processes )
( 3 7 4 ) 2 2 ) 8 2 954 2) 955 3) 5) J = σe 2 6) 955 7) 9) 955 Statistical-Mechanical Theory of Irreversible Processes 957 ) 3 4 2 A B H (t) = Ae iωt B(t) = B(ω)e iωt B(ω) = [ Φ R (ω) Φ R () ] iω Φ R (t)
30
3 ............................................2 2...........................................2....................................2.2...................................2.3..............................
A A = a 41 a 42 a 43 a 44 A (7) 1 (3) A = M 12 = = a 41 (8) a 41 a 43 a 44 (3) n n A, B a i AB = A B ii aa
1 2 21 2 2 [ ] a 11 a 12 A = a 21 a 22 (1) A = a 11 a 22 a 12 a 21 (2) 3 3 n n A A = n ( 1) i+j a ij M ij i =1 n (3) j=1 M ij A i j (n 1) (n 1) 2-1 3 3 A A = a 11 a 12 a 13 a 21 a 22 a 23 a 31 a 32 a 33
S I. dy fx x fx y fx + C 3 C dy fx 4 x, y dy v C xt y C v e kt k > xt yt gt [ v dt dt v e kt xt v e kt + C k x v + C C k xt v k 3 r r + dr e kt S dt d
S I.. http://ayapin.film.s.dendai.ac.jp/~matuda /TeX/lecture.html PDF PS.................................... 3.3.................... 9.4................5.............. 3 5. Laplace................. 5....
1 No.1 5 C 1 I III F 1 F 2 F 1 F 2 2 Φ 2 (t) = Φ 1 (t) Φ 1 (t t). = Φ 1(t) t = ( 1.5e 0.5t 2.4e 4t 2e 10t ) τ < 0 t > τ Φ 2 (t) < 0 lim t Φ 2 (t) = 0
1 No.1 5 C 1 I III F 1 F 2 F 1 F 2 2 Φ 2 (t) = Φ 1 (t) Φ 1 (t t). = Φ 1(t) t = ( 1.5e 0.5t 2.4e 4t 2e 10t ) τ < 0 t > τ Φ 2 (t) < 0 lim t Φ 2 (t) = 0 0 < t < τ I II 0 No.2 2 C x y x y > 0 x 0 x > b a dx
20 4 20 i 1 1 1.1............................ 1 1.2............................ 4 2 11 2.1................... 11 2.2......................... 11 2.3....................... 19 3 25 3.1.............................
第5章 偏微分方程式の境界値問題
October 5, 2018 1 / 113 4 ( ) 2 / 113 Poisson 5.1 Poisson ( A.7.1) Poisson Poisson 1 (A.6 ) Γ p p N u D Γ D b 5.1.1: = Γ D Γ N 3 / 113 Poisson 5.1.1 d {2, 3} Lipschitz (A.5 ) Γ D Γ N = \ Γ D Γ p Γ N Γ
S I. dy fx x fx y fx + C 3 C vt dy fx 4 x, y dy yt gt + Ct + C dt v e kt xt v e kt + C k x v k + C C xt v k 3 r r + dr e kt S Sr πr dt d v } dt k e kt
S I. x yx y y, y,. F x, y, y, y,, y n http://ayapin.film.s.dendai.ac.jp/~matuda n /TeX/lecture.html PDF PS yx.................................... 3.3.................... 9.4................5..............
b3e2003.dvi
15 II 5 5.1 (1) p, q p = (x + 2y, xy, 1), q = (x 2 + 3y 2, xyz, ) (i) p rotq (ii) p gradq D (2) a, b rot(a b) div [11, p.75] (3) (i) f f grad f = 1 2 grad( f 2) (ii) f f gradf 1 2 grad ( f 2) rotf 5.2
f(x) = f(x ) + α(x)(x x ) α(x) x = x. x = f (y), x = f (y ) y = f f (y) = f f (y ) + α(f (y))(f (y) f (y )) f (y) = f (y ) + α(f (y)) (y y ) ( (2) ) f
22 A 3,4 No.3 () (2) (3) (4), (5) (6) (7) (8) () n x = (x,, x n ), = (,, n ), x = ( (x i i ) 2 ) /2 f(x) R n f(x) = f() + i α i (x ) i + o( x ) α,, α n g(x) = o( x )) lim x g(x) x = y = f() + i α i(x )
24 I ( ) 1. R 3 (i) C : x 2 + y 2 1 = 0 (ii) C : y = ± 1 x 2 ( 1 x 1) (iii) C : x = cos t, y = sin t (0 t 2π) 1.1. γ : [a, b] R n ; t γ(t) = (x
![24 I ( ) 1. R 3 (i) C : x 2 + y 2 1 = 0 (ii) C : y = ± 1 x 2 ( 1 x 1) (iii) C : x = cos t, y = sin t (0 t 2π) 1.1. γ : [a, b] R n ; t γ(t) = (x](/thumbs/94/118744578.jpg "24 I ( ) 1. R 3 (i) C : x 2 + y 2 1 = 0 (ii) C : y = ± 1 x 2 ( 1 x 1) (iii) C : x = cos t, y = sin t (0 t 2π) 1.1. γ : [a, b] R n ; t γ(t) = (x")
24 I 1.1.. ( ) 1. R 3 (i) C : x 2 + y 2 1 = 0 (ii) C : y = ± 1 x 2 ( 1 x 1) (iii) C : x = cos t, y = sin t (0 t 2π) 1.1. γ : [a, b] R n ; t γ(t) = (x 1 (t), x 2 (t),, x n (t)) ( ) ( ), γ : (i) x 1 (t),
2019 1 5 0 3 1 4 1.1.................... 4 1.1.1......................... 4 1.1.2........................ 5 1.1.3................... 5 1.1.4........................ 6 1.1.5......................... 6 1.2..........................
L. S. Abstract. Date: last revised on 9 Feb translated to Japanese by Kazumoto Iguchi. Original papers: Received May 13, L. Onsager and S.
L. S. Abstract. Date: last revised on 9 Feb 01. translated to Japanese by Kazumoto Iguchi. Original papers: Received May 13, 1953. L. Onsager and S. Machlup, Fluctuations and Irreversibel Processes, Physical
2 G(k) e ikx = (ik) n x n n! n=0 (k ) ( ) X n = ( i) n n k n G(k) k=0 F (k) ln G(k) = ln e ikx n κ n F (k) = F (k) (ik) n n= n! κ n κ n = ( i) n n k n
. X {x, x 2, x 3,... x n } X X {, 2, 3, 4, 5, 6} X x i P i. 0 P i 2. n P i = 3. P (i ω) = i ω P i P 3 {x, x 2, x 3,... x n } ω P i = 6 X f(x) f(x) X n n f(x i )P i n x n i P i X n 2 G(k) e ikx = (ik) n
構造と連続体の力学基礎
II 37 Wabash Avenue Bridge, Illinois 州 Winnipeg にある歩道橋 Esplanade Riel 橋6 6 斜張橋である必要は多分無いと思われる すぐ横に道路用桁橋有り しかも塔基部のレストランは 8 年には営業していなかった 9 9. 9.. () 97 [3] [5] k 9. m w(t) f (t) = f (t) + mg k w(t) Newton
1 (Berry,1975) 2-6 p (S πr 2 )p πr 2 p 2πRγ p p = 2γ R (2.5).1-1 : : : : ( ).2 α, β α, β () X S = X X α X β (.1) 1 2
2005 9/8-11 2 2.2 ( 2-5) γ ( ) γ cos θ 2πr πρhr 2 g h = 2γ cos θ ρgr (2.1) γ = ρgrh (2.2) 2 cos θ θ cos θ = 1 (2.2) γ = 1 ρgrh (2.) 2 2. p p ρgh p ( ) p p = p ρgh (2.) h p p = 2γ r 1 1 (Berry,1975) 2-6
‚åŁÎ“·„´Šš‡ðŠp‡¢‡½‹âfi`fiI…A…‰…S…−…Y…•‡ÌMarkovŸA“½fiI›ð’Í
Markov 2009 10 2 Markov 2009 10 2 1 / 25 1 (GA) 2 GA 3 4 Markov 2009 10 2 2 / 25 (GA) (GA) L ( 1) I := {0, 1} L f : I (0, ) M( 2) S := I M GA (GA) f (i) i I Markov 2009 10 2 3 / 25 (GA) ρ(i, j), i, j I
u = u(t, x 1,..., x d ) : R R d C λ i = 1 := x 2 1 x 2 d d Euclid Laplace Schrödinger N := {1, 2, 3,... } Z := {..., 3, 2, 1,, 1, 2, 3
2 2 1 5 5 Schrödinger i u t + u = λ u 2 u. u = u(t, x 1,..., x d ) : R R d C λ i = 1 := 2 + + 2 x 2 1 x 2 d d Euclid Laplace Schrödinger 3 1 1.1 N := {1, 2, 3,... } Z := {..., 3, 2, 1,, 1, 2, 3,... } Q
³ÎΨÏÀ
2017 12 12 Makoto Nakashima 2017 12 12 1 / 22 2.1. C, D π- C, D. A 1, A 2 C A 1 A 2 C A 3, A 4 D A 1 A 2 D Makoto Nakashima 2017 12 12 2 / 22 . (,, L p - ). Makoto Nakashima 2017 12 12 3 / 22 . (,, L p
n ξ n,i, i = 1,, n S n ξ n,i n 0 R 1,.. σ 1 σ i .10.14.15 0 1 0 1 1 3.14 3.18 3.19 3.14 3.14,. ii 1 1 1.1..................................... 1 1............................... 3 1.3.........................
通信容量制約を考慮したフィードバック制御 - 電子情報通信学会 情報理論研究会（IT） 若手研究者のための講演会
IT 1 2 1 2 27 11 24 15:20 16:05 ( ) 27 11 24 1 / 49 1 1940 Witsenhausen 2 3 ( ) 27 11 24 2 / 49 1940 2 gun director Warren Weaver, NDRC (National Defence Research Committee) Final report D-2 project #2,
H.Haken Synergetics 2nd (1978)
27 3 27 ) Ising Landau Synergetics Fokker-Planck F-P Landau F-P Gizburg-Landau G-L G-L Bénard/ Hopfield H.Haken Synergetics 2nd (1978) (1) Ising m T T C 1: m h Hamiltonian H = J ij S i S j h i S
phs.dvi
483F 3 6.........3... 6.4... 7 7.... 7.... 9.5 N (... 3.6 N (... 5.7... 5 3 6 3.... 6 3.... 7 3.3... 9 3.4... 3 4 7 4.... 7 4.... 9 4.3... 3 4.4... 34 4.4.... 34 4.4.... 35 4.5... 38 4.6... 39 5 4 5....
5 H Boltzmann Einstein Brown 5.1 Onsager [ ] Tr Tr Tr = dγ (5.1) A(p, q) Â 0 = Tr Âe βĥ0 Tr e βĥ0 = dγ e βh 0(p,q) A(p, q) dγ e βh 0(p,q) (5.2) e βĥ0
![5 H Boltzmann Einstein Brown 5.1 Onsager [ ] Tr Tr Tr = dγ (5.1) A(p, q) Â 0 = Tr Âe βĥ0 Tr e βĥ0 = dγ e βh 0(p,q) A(p, q) dγ e βh 0(p,q) (5.2) e βĥ0](/thumbs/99/141438380.jpg "5 H Boltzmann Einstein Brown 5.1 Onsager [ ] Tr Tr Tr = dγ (5.1) A(p, q) Â 0 = Tr Âe βĥ0 Tr e βĥ0 = dγ e βh 0(p,q) A(p, q) dγ e βh 0(p,q) (5.2) e βĥ0")
5 H Boltzmann Einstein Brown 5.1 Onsager [ ] Tr Tr Tr = dγ (5.1) A(p, q) Â = Tr Âe βĥ Tr e βĥ = dγ e βh (p,q) A(p, q) dγ e βh (p,q) (5.2) e βĥ A(p, q) p q Â(t) = Tr Â(t)e βĥ Tr e βĥ = dγ() e βĥ(p(),q())
,,,17,,, ( ),, E Q [S T F t ] < S t, t [, T ],,,,,,,,
![,,,17,,, ( ),, E Q [S T F t ] < S t, t [, T ],,,,,,,,](/thumbs/91/105754403.jpg ",,,17,,, ( ),, E Q [S T F t ] < S t, t [, T ],,,,,,,,")
14 5 1 ,,,17,,,194 1 4 ( ),, E Q [S T F t ] < S t, t [, T ],,,,,,,, 1 4 1.1........................................ 4 5.1........................................ 5.........................................
(2 X Poisso P (λ ϕ X (t = E[e itx ] = k= itk λk e k! e λ = (e it λ k e λ = e eitλ e λ = e λ(eit 1. k! k= 6.7 X N(, 1 ϕ X (t = e 1 2 t2 : Cauchy ϕ X (t
![(2 X Poisso P (λ ϕ X (t = E[e itx ] = k= itk λk e k! e λ = (e it λ k e λ = e eitλ e λ = e λ(eit 1. k! k= 6.7 X N(, 1 ϕ X (t = e 1 2 t2 : Cauchy ϕ X (t](/thumbs/67/56612994.jpg "(2 X Poisso P (λ ϕ X (t = E[e itx ] = k= itk λk e k! e λ = (e it λ k e λ = e eitλ e λ = e λ(eit 1. k! k= 6.7 X N(, 1 ϕ X (t = e 1 2 t2 : Cauchy ϕ X (t")
6 6.1 6.1 (1 Z ( X = e Z, Y = Im Z ( Z = X + iy, i = 1 (2 Z E[ e Z ] < E[ Im Z ] < Z E[Z] = E[e Z] + ie[im Z] 6.2 Z E[Z] E[ Z ] : E[ Z ] < e Z Z, Im Z Z E[Z] α = E[Z], Z = Z Z 1 {Z } E[Z] = α = α [ α ]
,. Black-Scholes u t t, x c u 0 t, x x u t t, x c u t, x x u t t, x + σ x u t, x + rx ut, x rux, t 0 x x,,.,. Step 3, 7,,, Step 6., Step 4,. Step 5,,.
9 α ν β Ξ ξ Γ γ o δ Π π ε ρ ζ Σ σ η τ Θ θ Υ υ ι Φ φ κ χ Λ λ Ψ ψ µ Ω ω Def, Prop, Th, Lem, Note, Remark, Ex,, Proof, R, N, Q, C [a, b {x R : a x b} : a, b {x R : a < x < b} : [a, b {x R : a x < b} : a,
* 1 2014 7 8 *1 iii 1. Newton 1 1.1 Newton........................... 1 1.2............................. 4 1.3................................. 5 2. 9 2.1......................... 9 2.2........................
Morse ( ) 2014
Morse ( ) 2014 1 1 Morse 1 1.1 Morse................................ 1 1.2 Morse.............................. 7 2 12 2.1....................... 12 2.2.................. 13 2.3 Smale..............................
Part () () Γ Part ,
Contents a 6 6 6 6 6 6 6 7 7. 8.. 8.. 8.3. 8 Part. 9. 9.. 9.. 3. 3.. 3.. 3 4. 5 4.. 5 4.. 9 4.3. 3 Part. 6 5. () 6 5.. () 7 5.. 9 5.3. Γ 3 6. 3 6.. 3 6.. 3 6.3. 33 Part 3. 34 7. 34 7.. 34 7.. 34 8. 35
I-2 (100 ) (1) y(x) y dy dx y d2 y dx 2 (a) y + 2y 3y = 9e 2x (b) x 2 y 6y = 5x 4 (2) Bernoulli B n (n = 0, 1, 2,...) x e x 1 = n=0 B 0 B 1 B 2 (3) co
16 I ( ) (1) I-1 I-2 I-3 (2) I-1 ( ) (100 ) 2l x x = 0 y t y(x, t) y(±l, t) = 0 m T g y(x, t) l y(x, t) c = 2 y(x, t) c 2 2 y(x, t) = g (A) t 2 x 2 T/m (1) y 0 (x) y 0 (x) = g c 2 (l2 x 2 ) (B) (2) (1)
I 1
I 1 1 1.1 1. 3 m = 3 1 7 µm. cm = 1 4 km 3. 1 m = 1 1 5 cm 4. 5 cm 3 = 5 1 15 km 3 5. 1 = 36 6. 1 = 8.64 1 4 7. 1 = 3.15 1 7 1 =3 1 7 1 3 π 1. 1. 1 m + 1 cm = 1.1 m. 1 hr + 64 sec = 1 4 sec 3. 3. 1 5 kg
( 12 ( ( ( ( Levi-Civita grad div rot ( ( = 4 : 6 3 1 1.1 f(x n f (n (x, d n f(x (1.1 dxn f (2 (x f (x 1.1 f(x = e x f (n (x = e x d dx (fg = f g + fg (1.2 d dx d 2 dx (fg = f g + 2f g + fg 2... d n n
ohpmain.dvi
fujisawa@ism.ac.jp 1 Contents 1. 2. 3. 4. γ- 2 1. 3 10 5.6, 5.7, 5.4, 5.5, 5.8, 5.5, 5.3, 5.6, 5.4, 5.2. 5.5 5.6 +5.7 +5.4 +5.5 +5.8 +5.5 +5.3 +5.6 +5.4 +5.2 =5.5. 10 outlier 5 5.6, 5.7, 5.4, 5.5, 5.8,
ii 3.,. 4. F. ( ), ,,. 8.,. 1. (75% ) (25% ) =7 24, =7 25, =7 26 (. ). 1.,, ( ). 3.,...,.,.,.,.,. ( ) (1 2 )., ( ), 0., 1., 0,.
(1 C205) 4 10 (2 C206) 4 11 (2 B202) 4 12 25(2013) http://www.math.is.tohoku.ac.jp/~obata,.,,,..,,. 1. 2. 3. 4. 5. 6. 7. 8. 1., 2007 ( ).,. 2. P. G., 1995. 3. J. C., 1988. 1... 2.,,. ii 3.,. 4. F. ( ),..
25 7 18 1 1 1.1 v.s............................. 1 1.1.1.................................. 1 1.1.2................................. 1 1.1.3.................................. 3 1.2................... 3
18 I ( ) (1) I-1,I-2,I-3 (2) (3) I-1 ( ) (100 ) θ ϕ θ ϕ m m l l θ ϕ θ ϕ 2 g (1) (2) 0 (3) θ ϕ (4) (3) θ(t) = A 1 cos(ω 1 t + α 1 ) + A 2 cos(ω 2 t + α
18 I ( ) (1) I-1,I-2,I-3 (2) (3) I-1 ( ) (100 ) θ ϕ θ ϕ m m l l θ ϕ θ ϕ 2 g (1) (2) 0 (3) θ ϕ (4) (3) θ(t) = A 1 cos(ω 1 t + α 1 ) + A 2 cos(ω 2 t + α 2 ), ϕ(t) = B 1 cos(ω 1 t + α 1 ) + B 2 cos(ω 2 t
2011 ( ) ( ) ( ),,.,,.,, ,.. (. ), 1. ( ). ( ) ( ). : obata/,.,. ( )
2011 () () (),,.,,.,,. 1. 2. 3. 4. 5. 6. 7. 8. 9. 10.,.. (. ), 1. ( ). ()(). : www.math.is.tohoku.ac.jp/ obata/,.,. () obata@math.is.tohoku.ac.jp http://www.dais.is.tohoku.ac.jp/ amf/, (! 22 10.6; 23 10.20;
meiji_resume_1.PDF
β β β (q 1,q,..., q n ; p 1, p,..., p n ) H(q 1,q,..., q n ; p 1, p,..., p n ) Hψ = εψ ε k = k +1/ ε k = k(k 1) (x, y, z; p x, p y, p z ) (r; p r ), (θ; p θ ), (ϕ; p ϕ ) ε k = 1/ k p i dq i E total = E
2011de.dvi
211 ( 4 2 1. 3 1.1............................... 3 1.2 1- -......................... 13 1.3 2-1 -................... 19 1.4 3- -......................... 29 2. 37 2.1................................ 37
I A A441 : April 21, 2014 Version : Kawahira, Tomoki TA (Kondo, Hirotaka ) Google
I4 - : April, 4 Version :. Kwhir, Tomoki TA (Kondo, Hirotk) Google http://www.mth.ngoy-u.c.jp/~kwhir/courses/4s-biseki.html pdf 4 4 4 4 8 e 5 5 9 etc. 5 6 6 6 9 n etc. 6 6 6 3 6 3 7 7 etc 7 4 7 7 8 5 59
Note.tex 2008/09/19( )
1 20 9 19 2 1 5 1.1........................ 5 1.2............................. 8 2 9 2.1............................. 9 2.2.............................. 10 3 13 3.1.............................. 13 3.2..................................
r 1 m A r/m i) t ii) m i) t B(t; m) ( B(t; m) = A 1 + r ) mt m ii) B(t; m) ( B(t; m) = A 1 + r ) mt m { ( = A 1 + r ) m } rt r m n = m r m n B
1 1.1 1 r 1 m A r/m i) t ii) m i) t Bt; m) Bt; m) = A 1 + r ) mt m ii) Bt; m) Bt; m) = A 1 + r ) mt m { = A 1 + r ) m } rt r m n = m r m n Bt; m) Aert e lim 1 + 1 n 1.1) n!1 n) e a 1, a 2, a 3,... {a n
untitled
- k k k = y. k = ky. y du dx = ε ux ( ) ux ( ) = ax+ b x u() = ; u( ) = AE u() = b= u () = a= ; a= d x du ε x = = = dx dx N = σ da = E ε da = EA ε A x A x x - σ x σ x = Eε x N = EAε x = EA = N = EA k =
/ Christopher Essex Radiation and the Violation of Bilinearity in the Thermodynamics of Irreversible Processes, Planet.Space Sci.32 (1984) 1035 Radiat
/ Christopher Essex Radiation and the Violation of Bilinearity in the Thermodynamics of Irreversible Processes, Planet.Space Sci.32 (1984) 1035 Radiation and the Continuing Failure of the Bilinear Formalism,
II 2 II
II 2 II 2005 yugami@cc.utsunomiya-u.ac.jp 2005 4 1 1 2 5 2.1.................................... 5 2.2................................. 6 2.3............................. 6 2.4.................................
1 filename=mathformula tex 1 ax 2 + bx + c = 0, x = b ± b 2 4ac, (1.1) 2a x 1 + x 2 = b a, x 1x 2 = c a, (1.2) ax 2 + 2b x + c = 0, x = b ± b 2
filename=mathformula58.tex ax + bx + c =, x = b ± b 4ac, (.) a x + x = b a, x x = c a, (.) ax + b x + c =, x = b ± b ac. a (.3). sin(a ± B) = sin A cos B ± cos A sin B, (.) cos(a ± B) = cos A cos B sin
08-Note2-web
r(t) t r(t) O v(t) = dr(t) dt a(t) = dv(t) dt = d2 r(t) dt 2 r(t), v(t), a(t) t dr(t) dt r(t) =(x(t),y(t),z(t)) = d 2 r(t) dt 2 = ( dx(t) dt ( d 2 x(t) dt 2, dy(t), dz(t) dt dt ), d2 y(t) dt 2, d2 z(t)
II No.01 [n/2] [1]H n (x) H n (x) = ( 1) r n! r!(n 2r)! (2x)n 2r. r=0 [2]H n (x) n,, H n ( x) = ( 1) n H n (x). [3] H n (x) = ( 1) n dn x2 e dx n e x2
![II No.01 [n/2] [1]H n (x) H n (x) = ( 1) r n! r!(n 2r)! (2x)n 2r. r=0 [2]H n (x) n,, H n ( x) = ( 1) n H n (x). [3] H n (x) = ( 1) n dn x2 e dx n e x2](/thumbs/101/149159646.jpg "II No.01 [n/2] [1]H n (x) H n (x) = ( 1) r n! r!(n 2r)! (2x)n 2r. r=0 [2]H n (x) n,, H n ( x) = ( 1) n H n (x). [3] H n (x) = ( 1) n dn x2 e dx n e x2")
II No.1 [n/] [1]H n x) H n x) = 1) r n! r!n r)! x)n r r= []H n x) n,, H n x) = 1) n H n x) [3] H n x) = 1) n dn x e dx n e x [4] H n+1 x) = xh n x) nh n 1 x) ) d dx x H n x) = H n+1 x) d dx H nx) = nh
http://www.ike-dyn.ritsumei.ac.jp/ hyoo/wave.html 1 1, 5 3 1.1 1..................................... 3 1.2 5.1................................... 4 1.3.......................... 5 1.4 5.2, 5.3....................
( )
7..-8..8.......................................................................... 4.................................... 3...................................... 3..3.................................. 4.3....................................
1 4 1 ( ) ( ) ( ) ( ) () 1 4 2
7 1995, 2017 7 21 1 2 2 3 3 4 4 6 (1).................................... 6 (2)..................................... 6 (3) t................. 9 5 11 (1)......................................... 11 (2)
1 8, : 8.1 1, 2 z = ax + by + c ax by + z c = a b +1 x y z c = 0, (0, 0, c), n = ( a, b, 1). f = n i=1 a ii x 2 i + i<j 2a ij x i x j = ( x, A x), f =
1 8, : 8.1 1, z = ax + by + c ax by + z c = a b +1 x y z c = 0, (0, 0, c), n = ( a, b, 1). f = a ii x i + i
68 A mm 1/10 A. (a) (b) A.: (a) A.3 A.4 1 1
67 A Section A.1 0 1 0 1 Balmer 7 9 1 0.1 0.01 1 9 3 10:09 6 A.1: A.1 1 10 9 68 A 10 9 10 9 1 10 9 10 1 mm 1/10 A. (a) (b) A.: (a) A.3 A.4 1 1 A.1. 69 5 1 10 15 3 40 0 0 ¾ ¾ É f Á ½ j 30 A.3: A.4: 1/10
Aharonov-Bohm(AB) S 0 1/ 2 1/ 2 S t = 1/ 2 1/2 1/2 1/, (12.1) 2 1/2 1/2 *1 AB ( ) 0 e iθ AB S AB = e iθ, AB 0 θ 2π ϕ = e ϕ (ϕ ) ϕ
1 13 6 8 3.6.3 - Aharonov-BohmAB) S 1/ 1/ S t = 1/ 1/ 1/ 1/, 1.1) 1/ 1/ *1 AB ) e iθ AB S AB = e iθ, AB θ π ϕ = e ϕ ϕ ) ϕ 1.) S S ) e iθ S w = e iθ 1.3) θ θ AB??) S t = 4 sin θ 1 + e iθ AB e iθ AB + e
ohp_06nov_tohoku.dvi
2006 11 28 1. (1) ẋ = ax = x(t) =Ce at C C>0 a0 x(t) 0(t )!! 1 0.8 0.6 0.4 0.2 2 4 6 8 10-0.2 (1) a =2 C =1 1. (1) τ>0 (2) ẋ(t) = ax(t τ) 4 2 2 4 6 8 10-2 -4 (2) a =2 τ =1!! 1. (2) A. (2)
.1 z = e x +xy y z y 1 1 x 0 1 z x y α β γ z = αx + βy + γ (.1) ax + by + cz = d (.1') a, b, c, d x-y-z (a, b, c). x-y-z 3 (0,
.1.1 Y K L Y = K 1 3 L 3 L K K (K + ) 1 1 3 L 3 K 3 L 3 K 0 (K + K) 1 3 L 3 K 1 3 L 3 lim K 0 K = L (K + K) 1 3 K 1 3 3 lim K 0 K = 1 3 K 3 L 3 z = f(x, y) x y z x-y-z.1 z = e x +xy y 3 x-y ( ) z 0 f(x,
newmain.dvi
数論 サンプルページ この本の定価 判型などは, 以下の URL からご覧いただけます. http://www.morikita.co.jp/books/mid/008142 このサンプルページの内容は, 第 2 版 1 刷発行当時のものです. Daniel DUVERNEY: THÉORIE DES NOMBRES c Dunod, Paris, 1998, This book is published
( ) ( )
20 21 2 8 1 2 2 3 21 3 22 3 23 4 24 5 25 5 26 6 27 8 28 ( ) 9 3 10 31 10 32 ( ) 12 4 13 41 0 13 42 14 43 0 15 44 17 5 18 6 18 1 1 2 2 1 2 1 0 2 0 3 0 4 0 2 2 21 t (x(t) y(t)) 2 x(t) y(t) γ(t) (x(t) y(t))
III 1 (X, d) d U d X (X, d). 1. (X, d).. (i) d(x, y) d(z, y) d(x, z) (ii) d(x, y) d(z, w) d(x, z) + d(y, w) 2. (X, d). F X.. (1), X F, (2) F 1, F 2 F
III 1 (X, d) d U d X (X, d). 1. (X, d).. (i) d(x, y) d(z, y) d(x, z) (ii) d(x, y) d(z, w) d(x, z) + d(y, w) 2. (X, d). F X.. (1), X F, (2) F 1, F 2 F F 1 F 2 F, (3) F λ F λ F λ F. 3., A λ λ A λ. B λ λ
21 2 26 i 1 1 1.1............................ 1 1.2............................ 3 2 9 2.1................... 9 2.2.......... 9 2.3................... 11 2.4....................... 12 3 15 3.1..........
II ( ) (7/31) II ( [ (3.4)] Navier Stokes [ (6/29)] Navier Stokes 3 [ (6/19)] Re
![II ( ) (7/31) II ( [ (3.4)] Navier Stokes [ (6/29)] Navier Stokes 3 [ (6/19)] Re](/thumbs/94/118770263.jpg "II ( ) (7/31) II ( [ (3.4)] Navier Stokes [ (6/29)] Navier Stokes 3 [ (6/19)] Re")
II 29 7 29-7-27 ( ) (7/31) II (http://www.damp.tottori-u.ac.jp/~ooshida/edu/fluid/) [ (3.4)] Navier Stokes [ (6/29)] Navier Stokes 3 [ (6/19)] Reynolds [ (4.6), (45.8)] [ p.186] Navier Stokes I Euler Navier
st.dvi
9 3 5................................... 5............................. 5....................................... 5.................................. 7.........................................................................
,, Andrej Gendiar (Density Matrix Renormalization Group, DMRG) 1 10 S.R. White [1, 2] 2 DMRG ( ) [3, 2] DMRG Baxter [4, 5] 2 Ising 2 1 Ising 1 1 Ising
![,, Andrej Gendiar (Density Matrix Renormalization Group, DMRG) 1 10 S.R. White [1, 2] 2 DMRG ( ) [3, 2] DMRG Baxter [4, 5] 2 Ising 2 1 Ising 1 1 Ising](/thumbs/94/118770363.jpg ",, Andrej Gendiar (Density Matrix Renormalization Group, DMRG) 1 10 S.R. White [1, 2] 2 DMRG ( ) [3, 2] DMRG Baxter [4, 5] 2 Ising 2 1 Ising 1 1 Ising")
,, Andrej Gendiar (Density Matrix Renormalization Group, DMRG) 1 10 S.R. White [1, 2] 2 DMRG ( ) [3, 2] DMRG Baxter [4, 5] 2 Ising 2 1 Ising 1 1 Ising Model 1 Ising 1 Ising Model N Ising (σ i = ±1) (Free
1 (1) () (3) I 0 3 I I d θ = L () dt θ L L θ I d θ = L = κθ (3) dt κ T I T = π κ (4) T I κ κ κ L l a θ L r δr δl L θ ϕ ϕ = rθ (5) l
1 1 ϕ ϕ ϕ S F F = ϕ (1) S 1: F 1 1 (1) () (3) I 0 3 I I d θ = L () dt θ L L θ I d θ = L = κθ (3) dt κ T I T = π κ (4) T I κ κ κ L l a θ L r δr δl L θ ϕ ϕ = rθ (5) l : l r δr θ πrδr δf (1) (5) δf = ϕ πrδr
Maxwell
I 2018 12 13 0 4 1 6 1.1............................ 6 1.2 Maxwell......................... 8 1.3.......................... 9 1.4..................... 11 1.5..................... 12 2 13 2.1...................
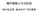4 5.............................................. 5............................................ 6.............................................. 7......................................... 8.3.................................................4.........................................4..............................................4................................................4.3...............................................
B [ 0.1 ] x > 0 x 6= 1 f(x) µ 1 1 xn 1 + sin sin x 1 x 1 f(x) := lim. n x n (1) lim inf f(x) (2) lim sup f(x) x 1 0 x 1 0 (
![B [ 0.1 ] x > 0 x 6= 1 f(x) µ 1 1 xn 1 + sin sin x 1 x 1 f(x) := lim. n x n (1) lim inf f(x) (2) lim sup f(x) x 1 0 x 1 0 (](/thumbs/97/133036028.jpg "B [ 0.1 ] x > 0 x 6= 1 f(x) µ 1 1 xn 1 + sin sin x 1 x 1 f(x) := lim. n x n (1) lim inf f(x) (2) lim sup f(x) x 1 0 x 1 0 (")
. 28 4 14 [.1 ] x > x 6= 1 f(x) µ 1 1 xn 1 + sin + 2 + sin x 1 x 1 f(x) := lim. 1 + x n (1) lim inf f(x) (2) lim sup f(x) x 1 x 1 (3) lim inf x 1+ f(x) (4) lim sup f(x) x 1+ [.2 ] [, 1] Ω æ x (1) (2) nx(1
6kg 1.1m 1.m.1m.1 l λ ϵ λ l + λ l l l dl dl + dλ ϵ dλ dl dl + dλ dl dl 3 1. JIS 1 6kg 1% 66kg 1 13 σ a1 σ m σ a1 σ m σ m σ a1 f f σ a1 σ a1 σ m f 4
35-8585 7 8 1 I I 1 1.1 6kg 1m P σ σ P 1 l l λ λ l 1.m 1 6kg 1.1m 1.m.1m.1 l λ ϵ λ l + λ l l l dl dl + dλ ϵ dλ dl dl + dλ dl dl 3 1. JIS 1 6kg 1% 66kg 1 13 σ a1 σ m σ a1 σ m σ m σ a1 f f σ a1 σ a1 σ m
Untitled
II 14 14-7-8 8/4 II (http://www.damp.tottori-u.ac.jp/~ooshida/edu/fluid/) [ (3.4)] Navier Stokes [ 6/ ] Navier Stokes 3 [ ] Reynolds [ (4.6), (45.8)] [ p.186] Navier Stokes I 1 balance law t (ρv i )+ j
: , 2.0, 3.0, 2.0, (%) ( 2.
2017 1 2 1.1...................................... 2 1.2......................................... 4 1.3........................................... 10 1.4................................. 14 1.5..........................................
2.2 ( y = y(x ( (x 0, y 0 y (x 0 (y 0 = y(x 0 y = y(x ( y (x 0 = F (x 0, y(x 0 = F (x 0, y 0 (x 0, y 0 ( (x 0, y 0 F (x 0, y 0 xy (x, y (, F (x, y ( (
(. x y y x f y = f(x y x y = y(x y x y dx = d dx y(x = y (x = f (x y = y(x x ( (differential equation ( + y 2 dx + xy = 0 dx = xy + y 2 2 2 x y 2 F (x, y = xy + y 2 y = y(x x x xy(x = F (x, y(x + y(x 2
5 Armitage x 1,, x n y i = 10x i + 3 y i = log x i {x i } {y i } 1.2 n i i x ij i j y ij, z ij i j 2 1 y = a x + b ( cm) x ij (i j )
5 Armitage. x,, x n y i = 0x i + 3 y i = log x i x i y i.2 n i i x ij i j y ij, z ij i j 2 y = a x + b 2 2. ( cm) x ij (i j ) (i) x, x 2 σ 2 x,, σ 2 x,2 σ x,, σ x,2 t t x * (ii) (i) m y ij = x ij /00 y
II Brown Brown
II 16 12 5 1 Brown 3 1.1..................................... 3 1.2 Brown............................... 5 1.3................................... 8 1.4 Markov.................................... 1 1.5
chap10.dvi
. q {y j } I( ( L y j =Δy j = u j = C l ε j l = C(L ε j, {ε j } i.i.d.(,i q ( l= y O p ( {u j } q {C l } A l C l
9 2 1 f(x, y) = xy sin x cos y x y cos y y x sin x d (x, y) = y cos y (x sin x) = y cos y(sin x + x cos x) x dx d (x, y) = x sin x (y cos y) = x sin x
2009 9 6 16 7 1 7.1 1 1 1 9 2 1 f(x, y) = xy sin x cos y x y cos y y x sin x d (x, y) = y cos y (x sin x) = y cos y(sin x + x cos x) x dx d (x, y) = x sin x (y cos y) = x sin x(cos y y sin y) y dy 1 sin
1 2 LDA Local Density Approximation 2 LDA 1 LDA LDA N N N H = N [ 2 j + V ion (r j ) ] + 1 e 2 2 r j r k j j k (3) V ion V ion (r) = I Z I e 2 r
![1 2 LDA Local Density Approximation 2 LDA 1 LDA LDA N N N H = N [ 2 j + V ion (r j ) ] + 1 e 2 2 r j r k j j k (3) V ion V ion (r) = I Z I e 2 r](/thumbs/91/104998910.jpg "1 2 LDA Local Density Approximation 2 LDA 1 LDA LDA N N N H = N [ 2 j + V ion (r j ) ] + 1 e 2 2 r j r k j j k (3) V ion V ion (r) = I Z I e 2 r")
11 March 2005 1 [ { } ] 3 1/3 2 + V ion (r) + V H (r) 3α 4π ρ σ(r) ϕ iσ (r) = ε iσ ϕ iσ (r) (1) KS Kohn-Sham [ 2 + V ion (r) + V H (r) + V σ xc(r) ] ϕ iσ (r) = ε iσ ϕ iσ (r) (2) 1 2 1 2 2 1 1 2 LDA Local