( ) 1
|
|
|
- ゆずさ かりこめ
- 5 years ago
- Views:
Transcription
1 ( ) 1
2 1 ( ),,. R R R R : (a, b) a + b, R R R : (a, b) a b, R. (R1) R +. (R2) R a (b c) = (a b) c. (R3) R a (b + c) = a b + a c, (a + b) c = a c + b c. (R4) R 0, R e 0, a R e a = a e = a. e R, 1 1 R. 0 0 R. R a b = b a, R.. R, R Z R Z R = {a R b R a b = b a }. Z R R. Z R R. R. R a, a b = b a = 1 R b R, a. b a 1, a. R R. R. R. K K. (K1) K. (K2) K = K {0}, K. 2
3 K K. K, K. K. A K, λ K, a, b A (λ a) b = a (λ b) = λ (a b), A K K. A K, K A : λ λ1 A. K 1 A K, K A. R, M +. ( ) M R M : (u, a) ua (1), a, b R, u, v M, M R. (M1) (u + v)a = ua + va (M2) u(a + b) = ua + ub (M3) u(a b) = (ua)b (M4) u1 R = u R. R 1 R 2, M R 1 R 2., a 1 R 1, a 2 R 2, u M (a 1 u)a 2 = a 1 (ua 2 ), M (R 1, R 2 ). R. R M, {0} M R, R ( )R, R R ( ).. R, {0} R,. 3
4 2 2.1 R. M R. End R (M) End R (M) = {f : M M f R } (f + g)(u) = f(u) + g(u), (f g)(u) = f(g(u)) (u M)., End R (M). M. End R (M) M M : (f, u) f(u), M End R (M). R, M (End R (M), R). End R (M) Aut R (M), M. Aut R (M) = {f End R (M) f }. 1 (Schur) M R, End R (M). f End R (M), f 0. f 0, Kerf M {0} Imf. M, Kerf = {0} Imf = M, f, f Aut R (M). 2.2 R, m, n, M m,n (R) R m n. m = n, M n,n (R) M n (R). M n (R), R n. i = 1, 2,, n, j = 1, 2,, n, e ij (i, j) 1, 0 M n (R). e ij M n (R). e ij. e ij e kl = { eil (j = k) 0 Mn (R) (j k) (1 i, j, k, l n). 4
5 e ij M n (R)e ij e ij M n (R) 0 0 a 1i 0 0 M n (R)e ij =..... j a 1i,, a ni R, 0 0 a ni e ij M n (R) = a j1 a jn i a j1,, a jn R M n (R) R. R a 1 R n a 2 =. a 1, a 2,, a n R a n. R n 1 0 e 1 = 0, e 2 = ,, e n = e 1,, e n R n. End R (R n ) f, a 1j a 2j f(e j ) =., (a 1j, a 2j,, a nj R, j = 1, 2,, n) a nj. M n (R) a f a f = (a ij ) 1 i,j n, f(u) = a f u (u R n ). f a f, End R (R n ) = M n (R)., End R (R n ) M n (R). 2.3 D. 1 M n (D). 5
6 e ij, (1 i, j n) M n (D). I M n (D) {0 Mn (D)}, 0 Mn (D) a I. a (k, l) a kl 0. (a 1 kl e ik) a e li = e ii i = 1, 2,, n. I 1 Mn (D) = e 11 + e e nn = n (a 1 kl e ik) a e li I i=1, I. I = M n (D). 2 D n M n (D). D n e 1, e 2,, e n, M n (D) {e ij } 1 i,j n. D n 0 u = e 1 a e n a n, (a 1,, a n D). j a j 0, i = 1, 2,, n, a 1 j e ij u a 1 j e ij u = e i., e 1, e 2,, e n u M n (D) M n (D)u. M n (D)u = D n u D, u 0. 3 M n (D) D n. M n (D) D n. M n (D) {e ij } 1 i,j n, 1 Mn (D) = e 11 + e e nn. M M n (D), 0 u M. u = 1 Mn (D)u = e 11 u + e 22 u + + e nn u, i e ii u 0. e ii u M n (D) M n (D)e ii u. M M = M n (D)e ii u. M n (D)e ii M n (D) D n. M n (D)e ii M n (D)e ii u = M : a au M n (D), M n (D)e ii = D n., M n (D). M M n (D). M n (D) {a1 Mn (D) a D} D, M D. M n (D) = 1 i,j n De ij 6
7 , M D. M D. M M n (D),., D, M, M M n (D). N. u M u N. N, M/N, M = N + M n (D)u. M n (D)u = M n (D)e 11 u + M n (D)e 22 u + + M n (D)e nn u, i, {0} M n (D)e ii u N., D n = M n (D)e ii u, M n (D)e ii u. M n (D)e ii u N M n (D)e ii u M n (D), M n (D)e ii u N M n (D)e ii u N = {0}. M/N M = N + M n (D)e ii u = N M n (D)e ii u = N D n. D. 7
8 3 R, I {0} R. A R, B R AB = { a i b i a i A, b i B}. 3.1 R J, {0} J I, I. I R. End R (I): R I 1 I. (1) x I, x 0, I = xr. (2) x I, xi {0}, I e, e 2 = e xe = x. (1) x 0, {0} xr I. I I = xr. (2) R φ : I I φ(a) = xa. φ End R (I), φ Aut R (I). x I, I e φ(e) = x. xe = x. x(e 2 e) = 0, e 2 = e., R 0 e e 2 = e. e, ere = {eae a R}, R., eae, ebe ere eae + ebe = e(a + b)e ere (eae)(ebe) = e(aeb)e ere. e(eae) = eae = (eae)e, e ere, 0 R ere. ere. 2 e R, e I = er. R I End R (I). f End R (I), ψ(f) = f(e)e, ψ(f) ere, ψ : End R (I) ere. I, ψ. f End R (I), f(e) I = er f(e)e Ie = ere. ψ. f, g End R (I). ψ(f + g) = (f + g)(e)e = (f(e) + g(e))e = f(e)e + g(e)e = ψ(f) + ψ(g) 8
9 . f(e) = ea, g(e) = eb, f R e 2 = e ψ(f g) = (f g)(e)e = f(g(e))e = f(eb)e = f(e)be = f(e 2 )be = f(e)ebe = eaebe = (eae)(ebe) = ψ(f)ψ(g). id I End R (I) I, ψ(id I ) = e 2 = e. ψ.. c R, f c End R (I) f c (x) = ecx (x I). ψ(f c ) = ece ψ. Kerψ End R (I). I End R (I), Kerψ = {0}. ψ. 3.2 Wedderburn I, R I S = End R (I), S I T = End S (I). I (S, R) (S, T ). R a, ι a T u ι a = ua (u I). a ι a ι : R T. 3 a I, t T ι a t = ι a t. ι I ι(i) T. I b, s b S s b u = bu (u I)., t T a I (ba) t = (s b a) t = s b (a t) = b(a t), b (ι a t) = (b ι a ) t = b ι a t b I. ι a t = ι a t. 4 R, ι : R T. R, ι. ι. ι ι(r). ι 1R = 1 T ι(r), ι(r)t ι(r). I R RI. I, RI. R R = RI., ι(r) = ι(r)ι(i), ι(i)t = ι(i) ι(r)t ι(r). 2 R. (1) (2). 9
10 (1) R. (2) R D n M n (D). (1). I. R I, End R (I). D = End R (I). I D. J = {a R Ia = {0}}, J R. J R, R J = {0}. II {0}, I e. I I = er, D Ie = ere. Ie D. S = {a R dim D Ia < }, S R, e S, {0} S. R S = R, 1 R S, dim D I = n <. R = End D (I) = M n (D). 5 D 1 D 2 M n1 (D 1 ) = M n2 (D 2 ), n 1 = n 2 D 1 = D2. R = M n1 (D 1 ) = M n2 (D 2 ), I. D = End R (I). e 11 M n1 (D 1 ), I = e 11 M n1 (D 1 ). e 11 I,, D = e 11 Re 11 = D1. D = D 2 D 1 = D2., dim D I = n 1 = n 2. 6 R Z R. R Z R a Z R, a 0, Ra = ar, Ra. Ra = R, a a 1 R. b R, a b = b a, b a 1 = a 1 b. a 1 Z R. 7 D. Z Mn (D) = Z D. 10
11 4 K. 4.1 K R,. (CS1) R K. (CS2) Z R = K.,. A(K) = K A s (K) = K A cs (K) = K D(K) = K D(K) A cs (K) A s (K) A(K).. 3 R A s (K), K D R = M n (D). R A cs (K) D D(K). 4 K. A s (K) = A cs (K), D(K) = {K}, A A s (K) M n (K). A A s (K), K Z A. Z A /K K = Z A, A A cs (K). A s (K) = A cs (K)., A = M n (D), D D(K). a D, K K(a) D, K(a)/K, K = K(a), a K. D = K, A = M n (K). 4.2 A, B A(K), A B K A B. A B A(K). 8 A, B A(K) = Z A B = Z A Z B. {b i } B K, A B x x = i x i b i, (x i A) 11
12 . x Z A B, a A (a 1)x = x(a 1), (ax i ) b i = (x i a) b i i i. i ax i = x i a, x i Z A. Z A B Z A B. {a i } Z A K, Z A B y y = a i y i, (y i B) i. y Z A B y i Z B, Z A B Z A Z B. Z A Z B Z A B. 5 A A cs (K), B A(K). 0 I A B, I 1 A b 0. A, 0 a A, x 1, x 1, x 2, x 2,, x m, x m A. x I m x i ax i = 1 A (2) i=1 p(x) = min{j x = a 1 b a j b j, a i A, b i B} p = min{p(x) 0 x I}. p = 1. p > 1. x I, p(x) = p, x = a 1 b a p b p. a p = 1 A., a p 1 a p. ( p(x) < p ). a p 1 / Z A, c A, ca p 1 a p 1 c 0 x = (c 1)x x(c 1) = (ca 1 a 1 c) b (ca p 1 a p 1 c) b p 1 0 p(x ) < p. p = 1. 0 a b I. (x i 1)(a b)(x i 1) = 1 A b I. i 12
13 1 A A cs (K), B A s (K) = A B A s (K). 0 I A B, 0 1 A b I. B, y 1, y 1,, y n, y n B n y i by i = 1 B. n (1 A y i )(1 A b)(1 A y i) = 1 A 1 B I. i=1 i=1 2 A, B A cs (K) = A B A cs (K) L/K. A A cs (K) A L A cs (L). 3 A A cs (K), dim K A. K/K K. A K = M n (K), dim K A = n 2. A A cs (K), D D(K), A = M m (D). deg A = dim K A A, inda = dim K D A. 4.4 A A(K). A : A A A a b = ba (a, b A). A K. A, A. A A cs (K) A A cs (K). 10 A A cs (K), deg A = n. A A = End K (A) = M n 2(K). 13
14 . a A b A, ψ a,b End K (A) ψ a,b (x) = axb (x A). ψ : A A End K (A) : m a i b i i=1 m i=1 ψ ai,b i, ψ. A A ψ. ψ. 4.5 Brauer A, B A cs (K), 1 p, q Z A M p (K) = B M q (K), A B Brauer, A B.. A [A].. Br(K) = A cs (K)/ = 11 D 1, D 2 D(K). M n (D 1 ) M m (D 2 ) D 1 D 2 D 1 = D A, B A cs (K) A = B A B deg A = deg B 13 D(K)/ = K.. D(K)/ = Br(K) : (D ) [D]. 14
15 14 Br(K) [A] [B] = [A B], (A, B A cs (K)). (1) well-definied. (2) [K]. (3) [A] [A] 1 = [A ]... Br(K). K Brauer. 15 L/K.. Br(K) Br(L) : [A] [A L] 15
16 5 5.1 A A(K), X A, {a A x X ax = xa} X A Z A (X). Z A (X) A. 6 A A cs (K), B A. Z A (B) dim A = dim B dim Z A (B). ψ : A A End K (A), A A A. B A A A, A B A. f End K (A), f End B A (A) f ψ b,a = ψ b,a f (b B, a A )., ψ. Z A A (B A ) = End B A (A) Z A A (B A ) = Z A (B) Z A = ZA (B). B A, I B A, D = End B A (I), I D s B A = End D (I) = M s (D)., B A A = I k. Z A (B) = End B A (I k ) = M k (D), Z A (B). dim Z A (B) = k 2 dim D, dim B A = s 2 dim D, dim A = k dim I = ks dim D. dim B dim C = s k k2 dim D = dim A. 4 A A cs (K), B A K, K A = B Z A (B). Z A (B) K. B Z A (B) K. B Z A (B) A : x y xy B Z A (B) = A. 16
17 5.2 A A cs (K). L/K L A A. A. 16 L A. (1) C = Z A (L), C Z C = L. C A cs (L). (2) deg A = [L : K] deg C. [L : K] deg A. (1) C. B = L, D C = Z A (L) = M k (D), L A = Ms (D). L A A cs (L) D D(L). C A cs (L). (2) dim L C = m 2, dim K A = dim K L dim K C = [L : K]m 2 [L : K] = [L : K] 2 m 2. deg A = [L : K]m. L A, [L : K] = deg(a).. M n (C). 17 D D(K). L D, L. D. C = Z D (L)., L = Z C C D. L C, c C, c L. C, L(c) L. L = C, deg D = [L : K] 5.3 A A cs (K), deg A = n. L/K, L A. A L = M n (L) 17
18 18 A A cs (K), D D(K), A D. L/K L A L D 19 D D(K). L/K D deg D [L : K]. deg D = d, D L = M d (L). V D L. V = L d, D M d (L) = V d. [L : K] = dim D D L = dim D M d (L) = dim D V d = d dim D V, d [L : K]. 20 D D(K), L D.. L L L D deg D = d. ( =) [L : K] d, d [L : K]. [L : K] = d. (= ) [L : K] = d dim L D = d. D (D, L). φ : D L = D L End L (D) = M d (L). D L.. 4 D D(K), D K D = K. d = deg D, L D. [L : K] = d. (ch(k) = 0 ) 1 < d L/K. d = 1, D = K. (ch(k) = p > 0 ) L/K d = [L : K] = p m. K K, D K = M d (K)., K D D K : a a 1 φ : D M d (K). a D, K(a)/K a pk = λ K. p k = [K(a) : K] d = p m., M d (K) (φ(a) λ 1/pk 1 d ) pk = 0 18
19 . φ(a) λ 1/pk 1 d, 1 < d, p d dλ 1/pk = 0, Tr(φ(a)) dλ 1/pk = Tr(φ(a) λ 1/pk 1 d ) = 0. Tr(φ(a)) = 0 (a D) M d (L) = D K = φ(d)k, x M d (K) Tr(x) = 0. d = 1, D = K. 7 D D(K), L D, L/K. L = K D. C = Z D (L), C D(L). L C, L L C, L /K, L. L = C, L = Z D (L), L. 5 A A cs (K), K. 19
20 6 Skolem-Noether 6.1 Skolem-Noether 5 R A s (K), M 1 M 2 R. dim K M 1 = dim K M 2 M 1 = M2., M 1, M 2 R N. M 1 = N k, M 2 = N l dim K M 1 = dim K M 2 k = l M 1 = M2. A A cs (K). a A, i a : A A : i a (x) = axa 1 (x A). i a K.. Inn(A) = {i a a A }.. a i a A /Z A = Inn(A). 8 (Skolem-Noether) A A cs (K), R A s (K), f, g : R A K. i a Inn(A), g = i a f. D D(K), A = M n (D) = End D (D n ). D D. V = D n ( D ) (A D ) V V : (a d, v) avd A D. f : R A V R D V f. V f = V, (R D ) V f V f : (α d, v) f(α)vd. g : R A V R D V g. R D A s (K). dim K V f = dim K V = dim K V g, R D V f = Vg. φ : V f V g, φ(f(α)vd) = g(α)φ(v)d (α R, d D, v V ). D φ Aut D (V ) = A. A a a f(α)v = φ(f(α)v) = g(α)φ(v) = g(α)a v v V. g(α) = af(α)a 1 = i a f(α) α R, g = i a f. 20
21 6 A A cs (K), R A. f : R A, i a Inn(A) f = i a R. 6.2 Brauer 9 (Frobenius) D D(R) = D = H. 1 < deg D. L D. 1 < d = [L : R] = 2. L = C. τ : C C : α + β 1 α β 1. Skolem-Noether, i a Inn(D), i a C = τ. a(α + β 1)a 1 = α β 1. i a 2 C, a 2 Z D (C) = C. τ(a 2 ) = aa 2 a 1 = a 2 a 2 R. a 2 > 0 a 2 = t 2 (t R), a = ±t, i a C τ. a 2 < 0, a 2 = t 2., i = 1, j = at 1, k = ij i 2 = j 2 = k 2 = ijk = 1, dim R D = 4 D = R1 + Ri + Rj + Rk, D = H. 7 Br(R) = {±1}. 6.3 Brauer 6 G, H G. ghg 1 G. g G [G : H] = n > 1, H = h G = nh. G/H g 1,, g n ghg 1 = g G n i=1 g i Hg 1 i ghg 1 g i Hgi 1 (n 1) = nh (n 1) < nh = G g G i=1 21
22 10 (Wedderburn). D. K = Z D. q = K, deg D = d > 1. D L 1, L 2, [L 1 : K] = [L 2 : K] = d L 1 = L 2 = q d. L 1 = L2. Skolem-Noether, D. L 1 L 2. D. D,, D = al 1 a 1 a D,,. 8 K Br(K) = 0. 22
23 7 7.1 K. L/K n, Γ L/K =< σ >. a K, x. x 1 = x 0, x, x 2,, x n 1 L n V = L1 + Lx + + Lx n 1. (αx i )(βx j ) = { (ασ i (β))x i+j (i + j < n) (aασ i (β))x i+j n (i + j n) (0 i, j n 1. α, β L).. (CY1) x n = a. (CY2) xα = σ(α)x (α L). V K., V = (L/K, σ, a). 21 (L/K, σ, a) A cs (K).. 22 L A A cs (K), u A. (CY0) 1, u,, u n 1 L A. (CY1) u n K. (CY2) uαu 1 = σ(α) (α L). A = (L/K, σ, u n ). Skolem-Noether, σ : L L i u Inn(A). uαu 1 = σ(α) u n αu n = σ n (α) = α u n Z A (L) = L, (α L). (α L). σ(u n ) = uu n u 1 = u n u n K. 1, u,, u n 1 L. dim L A = n, A. 23
24 9 A A cs (K), A, A K. 11 (1) n k (L/K, σ, a) = (L/K, σ k, a k ). (2) (L/K, σ, a) = (L/K, σ, b) ab 1 Nr L/K (L ). (3) (L/K, σ, a) = M n (K) a Nr L/K (L ). (1) (L/K, σ k, a k ) = L1 + Ly + + Ly n 1. φ : (L/K, σ k, a k ) (L/K, σ, a) : { φ(α) = α (α L) φ(y) = x k. (2) A = (L/K, σ, a), B = (L/K, σ, b). ( =) ab 1 = δ δ σ δ σn 1 B = L1 + Ly + + Ly n 1. φ : A B : { φ(α) = α (α L) φ(x) = δy. (= ) φ : A B. Inn(B), φ L = id. i x Inn(A), i y Inn(B), i φ(x) L = i y L = σ. φ(x)y 1 Z B (L) = L, δ L φ(x) = δy. a = φ(a) = φ(x n ) = (δy) n = Nr L/K (δ)y n = Nr L/K (δ)b. (3) (2) (L/K, σ, a) = (L/K, σ, 1) a Nr L/K (L ), (L/K, σ, 1) = M n (K). M n (K) = End K (L). φ : (L/K, σ, 1) End K (L) : { φ(α) = α (α L) φ(x) = σ. 23 a, b K. (L/K, σ, a) (L/K, σ, b) (L/K, σ, ab). 24
25 7.2 n ch(k), µ n K 1 n. µ n K. 1 n ζ K. L/K n, Kummer, a K, L = K( n a), Γ L/K µ n : σ σ( n a) n a. Γ L/K σ σ( n a) n a = ζ. b K, (L/K, σ, b) (L/K, σ, b) = L1 + Ly + + Ly n 1 { (CY1) y n = b (CY2) yα = σ(α)y (α L). x = n a L = K1 + Kx + + Kx n 1, x n = a., (CY2) α = x. yα = σ(α)y (α L) yx = σ(x)y = ζxy. (L/K, σ, b) (L/K, σ, b) = Kx i y j 05i,j5n 1 x n = a y n = b yx = ζxy. a K. µ n K, n ζ µ n. a, b K, x n = a Kx i y j y n = b yx = ζxy 05i,j5n 1 K (a, b) n, ( ) a, b. K, ζ 24 (a, b) n A cs (K). A = (a, b) n, K(x) = K1 + Kx + + Kx n 1. K(x) A,. A u = f 0 (x)1 + f 1 (x)y + + f n 1 (x)y n 1, f j (x) = c 0j 1 + c 1j x + + c n 1j x n 1 K(x) 25
26 . ( ) u Z A. yu = uy c ij = c ij ζ i (0 i, j n 1). i 0 c ij = 0. u = c c 01 y + + c 0n 1 y n 1. xu = ux c 01 = = c 0n 1 = 0, u = c 00 K. Z A = K. ( ) 0 I A. l = min{deg y u u I}, I l = {u I deg y u = l} I, k = min{deg f(x) f(x) M}, M = { u I l y l } K(x). u I l u = f(x)y l + v + f 0 (x)1, deg f(x) = k, 1 deg y v l 1, f 0 (x) K(x). f(x) = c 0 + c 1 x + + c k x k. 1 k. I l u yuy 1 = (f(x) f(ζx))y l +, f(x) f(ζx) M 0 I x 1 (u yuy 1 ) = x 1 (f(x) f(ζx))y l +, deg x 1 (f(x) f(ζx)) = k 1, k. k = 0, f(x) = c 0 K u = c 0 y l + v + f 0 (x) I l. 1 l. f 0 (x) = 0, uy 1 I, deg y uy 1 = l 1 l, f 0 (x) 0. I u ζ l xux 1 = v ζ l xvx 1 + (1 ζ l )f 0 (x) 0 deg y (u ζ l xux 1 ) l 1, l. l = 0, I u = c 0 K I = A. 7 A A cs (K), deg A = n, A = M n (K) A K n = K K. 26
27 (= ). ( =). K n e 1,, e n e e n = 1, e i e j = δ ij e j. A A A = e 1 A e n A. A = M m (D), D D(K), D m A, m n. m deg A = n, m = n D = K. 12 d n. a, b K ( a d ) ( ), b a, b = K, ζ K, ζ d M d (K). (a n, b) n = Mn (K). n = dm. ζ d 1 m. A = (a d, b) n = Kx i y j. K(x) = K1 + Kx + + Kx n 1, K. K(x) = K[X]/(X n a d ) d 1 = K[X]/(X m aζ im ) i=0 (ζ i X mod (X m aζ im )) i=0,1,,d 1 K(x) z. z m = a, y d zy d = ζz. z w = y d ( ) B = Kz i w j a, b = K, ζ d 05i,j5m 1. B A cs (K), A = B Z A (B). x m Z A (B), t = x m Z A (B) K(t) = K1 + Kt + + Kt d 1 = K[X]/(X d a d ) = K d. deg Z A (B) = d, Z A (B) = M d (K). 27
28 10 a, b K, L X n a = 0 m = [L : K]. σ Γ L/K σ( n a) = ζ n/m n a, (a, b) n = (L/K, σ, b) Mn/q (K). (a, b) n = Mn (K) b Nr L/K (L ). c = ( n a) m K, L = K( m c). a = c n/m ( ) ( ) (a, b) n c, b c, b = K, ζ n/m M n/m (K), = K, ζ n/m (L/K, σ, b).,. (a, b) n = Mn (K) (L/K, σ, b) = M m (K) b Nr L/K (L ) 13 a, b, c K. (1) (a, b) n = (b 1, a) n (2) (a, b) n (a, c) n (a, bc) n, (a, b) n (c, b) n (ac, b) n. (3) (a, 1 a) n = Mn (K). (1) (a, b) n = Kx i y j, (b 1, a) n ) = Kz i w j. φ : (a, b) n (b 1, a) n : φ(x) = w, φ(y) = w 1. (2) L X n a = 0, (a, b) n (a, c) n (L/K, σ, b) (L/K, σ, c) (L/K, σ, bc) (a, bc) n. (3) (a, 1 a) n = Kx i y j. z = x + y z n = (x + y) n = x n + y n = a + 1 a = 1. K(z) = K1 + Kz + + Kz n 1 = K n, (a, 1 a) n = Mn (K). 28
29 7.3 K. A A cs (K) deg A = 2,. A. 8 A A cs (K). (1) A, A = M 2 (K). (2) A A. (1) A, inda < deg A = 2 A = M 2 (K). (2) A, A K L. [L : K] = deg A = 2, L/K. A. A = (L/K, σ, b). ch(k) 2, L = K( a) A = (a, b) 2 = K1 + Kx + Ky + Kxy, x 2 = a, y 2 = b, yx = xy. ch(k) = 2, L X 2 + X + a = 0,. A = K1 + Kx + Ky + Kxy, x 2 + x = a, y 2 = b, yxy 1 = x
30 8 8.1 Noether A A cs (K),. (CP) A K L. L/K Γ = Γ L/K. σ Γ Skolem-Noether, u σ A σ = i uσ L. ξ σ,τ = u σ u τ u 1 στ Z A (L) = L (σ, τ Γ). u σ u τ = ξ σ,τ u στ (u σ u τ )u ρ = u σ (u τ u ρ ) ξ σ,τ ξ στ,ρ = ξ σ,τρ σ(ξ τ,ρ ) (σ, τ, ρ Γ). A B = σ Γ Lu σ, {u σ } σ Γ L, dim K B = [L : K] 2 = dim K A, A A = σ Γ Lu σ, { uσ αu 1 σ = σ(α) (α L) u σ u τ = ξ σ,τ u στ. L/K, Γ = Γ L/K. ξ : Γ Γ L : (σ, τ) ξ σ,τ, ξ Noether 2. (NF) ξ σ,τ ξ στ,ρ = ξ σ,τρ σ(ξ τ,ρ ) (σ, τ, ρ Γ). Noether Z 2 (Γ, L ). 9 ξ Z 2 (Γ, L ) ξ e,σ = ξ e,e, ξ σ,e = σ(ξ e,e ), (σ Γ). (NF), σ = τ = e, ξ e,e ξ e,ρ = ξe,ρ 2, ξ e,e = ξ e,ρ. τ = ρ = e, ξσ,e 2 = ξ σ,e σ(ξ e,e ), ξ σ,e = σ(ξ e,e ). 30
31 10 ξ, η Z 2 (Γ, L ), ξ η : Γ Γ L (ξ η) σ,τ = ξ σ,τ η σ,τ, (σ, τ Γ), ξ η Z 2 (Γ, L ), Z 2 (Γ, L ). 1 1 σ,τ = 1 (σ, τ Γ). t : Γ L, t : Γ Γ L t σ,τ = t σ σ(t τ )t 1 στ, (σ, τ Γ), t.. B 2 (Γ, L ). 11 B 2 (Γ, L ) Z 2 (Γ, L ). Z 2 (Γ, L )/B 2 (Γ, L ), Γ L, H 2 (Γ, L ). ξ Z 2 (Γ, L ) [ξ], [1]. 8.2 L/K, Γ = Γ L/K. V = σ Γ Lx σ {x σ } σ Γ L. ξ Z 2 (Γ, L ), V (CP1) x σ α = σ(α)x σ, (α L, σ Γ). (CP2) x σ x τ = ξ σ,τ x στ, (σ, τ Γ).. V ξ 1 e,e x e K. L Γ ξ, (L, Γ, ξ).. 25 (L, Γ, ξ) A cs (K), L (L, Γ, ξ). 31
32 A = (L, Γ, ξ). ( ) Z A z = σ Γ a σ x σ. α L, αz = zα αa σ = σ(α)a σ, (σ Γ). σ e σ(α) α α L, a σ = 0. z = a e x e, zx σ = x σ z σ(a e )ξ σ,e = a e ξ e,σ. σ(a e ξ e,e ) = a e ξ e,e, a e ξ e,e K. z = a e x e = a e ξ e,e 1 A K. ( ) I A. π : A A/I. π L : L A/I, L A/I. x σ = π(x σ ). x σ α = σ(α)x σ (α L, σ Γ). {x σ } σ Γ L., J Γ {x σ } σ J. J Γ, τ J x τ = σ J a σ x σ. α L τ(α)x τ = x τ α = σ J a σ x σ α = σ J a σ σ(α)x σ 0 = σ J a σ (τ(α) σ(α))x σ. {x σ } σ J σ J a σ 0, a σ (τ(α) σ(α)) = 0. τ(α) = σ(α), (α L), τ = σ J. J = Γ. dim L A/I = dim L A = [L : K], I = A A cs (K), A K. 32
33 14 ξ, η Z 2 (Γ, L ) (L, Γ, ξ) = (L, Γ, η) [ξ] = [η]. A = (L, Γ, ξ) = Lx σ, B = (L, Γ, η) = Ly σ. (= ) φ : A B. Skolem-Noether φ L = id. φ(x σ )α = σ(α)φ(x σ ), (α L). φ(x σ ) = c τ y τ τ Γ,. φ(x σ ) = c σ y σ,. φ(x σ x τ ) = c σ y σ c τ y τ = c σ σ(c τ )y σ y τ = c σ σ(c τ )η σ,τ y στ φ(x σ x τ ) = φ(ξ σ,τ x στ ) = ξ σ,τ c στ y στ ξ σ,τ = ĉ σ,τ η σ,τ, [ξ] = [η]. ( =) [ξ] = [η], c : Γ L ξ = ĉ η. φ : A B φ( a σ x σ ) = a σ c σ y σ,. 15 (L, Γ, 1) = M n (K). (L, Γ, 1) = Lx σ. φ : (L, Γ, 1) End K (L) : { φ(α) = α (α L) φ(x σ ) = σ (σ Γ). 12 (L, Γ, ξ) = M n (K) [ξ] = [1]. 16 ξ, η Z 2 (Γ, L ) (L, Γ, ξ) (L, Γ, η) (L, Γ, ξ η). 33
34 A = (L, Γ, ξ) = Lx σ, B = (L, Γ, η) = Ly σ, C = (L, Γ, ξ η) = Lz σ. A, B L. A L, L V = A L B. V (A, B), A B. C V. (a L b )(a b) = a a L b b = a a L b b, (a, a A, b, b B) C V V : αz σ (a L b) = a (αx σ ) L y σ b = αx σ a L y σ b αz σ βz τ (a L b) = αz σ (βx τ a L y τ b) = (αx σ βx τ a) L (y σ y τ b) = (ασ(β)ξ σ,τ x στ a) L (η σ,τ y στ b) = (ασ(β)ξ σ,τ η σ,τ z στ )(a L b) = (αz σ βz τ )(a L b), V C. V (C, A B), K (A B) End C (V ). (A B),. dim K V = [L : K] dim L A dim L B = n 3 = n dim K C, C V = C n. End C (V ) = End C (C n ) = M n (End C (C)) = M n (C ) = C M n (K). dim K End C (V ) = n 2 dim K C = n 4 = dim K (A B) (A B) = EndC (V ) = C M n (K). A B C. 34
35 8.3 L/K n, Γ = Γ L/K =< σ >. 26 a K, (L/K, σ, a). ξ σ i,σ j = { 1 (i + j < n) a (i + j n) ξ, (L/K, σ, a) = (L, Γ, ξ). (L/K, σ, a) L,. (L/K, σ, a) = Lxσ, Noether ξ. 27 ξ Z 2 (Γ, L ), (L, Γ, ξ).,,. 35
36 9 Brauer 9.1 L/K,, Br(K) Br(L) : [A] [A L]. Br(L/K). Br(L/K) = {[A] Br(A) [A L] = [1]}. 28 Br(K) = Br(L/K). L/K :, A A cs (K) K. 17 L/K,. H 2 (Γ, L ) Br(L/K) : [ξ] [(L, Γ, ξ)] Z 2 (Γ, L ) Br(K) : ξ [(L, Γ, ξ)], B 2 (Γ, L ). H 2 (Γ, L ) Br(K). Br(L/K). D D(K) [D] Br(L/K). deg D = d, [D ] = [D] 1 Br(L/K) L D = Md (L). V = L d L D. V (L, D). D V n. End D (V ) = M n (D) dim K V = d[l : K] = n dim K D = nd 2 dim K End D (V ) = dim K M n (D) = d 2 n 2 = (dn) 2 = [L : K] 2. L End D (V ) : α α, L End D (V ), L End D (V )., End D (V ) = (L, Γ, ξ) ξ Z 2 (Γ, L ).. [D] = [M n (D)] = [End D (V )] = [(L, Γ, ξ)] 36
37 9.2 A A cs (K), [A] Br(K) A, exp(a). 29 m = ind(a) [A] m = [1]. exp(a) ind(a). L/K [A] Br(L/K). [A] = [(L, Γ, ξ)]. [A] m = [(L, Γ, ξ m )], ξ m B 2 (Γ, L ). B = (L, Γ, ξ) = M r (D), D D(K), V = D r B. L B, V L. [L : K] = deg(l, Γ, ξ) = deg M r (D) = rm dim K V = r dim K D = rm 2 = dim L V [L : K] = dim L V rm dim L V = m. V L v 1,, v m, b B bv i = b i1 v b in v n m m (b ij ) M m (L)., x σ X σ. ξ m B 2 (Γ, L ). B = Lx σ ξ σ,τ X στ = σ(x τ )X σ ξ m σ,τ det(x στ ) = σ(det(x τ )) det(x σ ) 13 Br(K). 30 p ind(a), p exp(a).. [A] = [(L, Γ, ξ)], (L, Γ, ξ) = M r (D) d = ind(a) = deg(d) Γ = [L : K] = (deg M r (D)) = dr p Γ. Γ p-sylow Γ p. L p = L Γp [L : L p ] = Γ p. p [L p : K], L p D. A. exp(a L p ) 1. [A L p ] Br(L/L p ), ind(a L p ) [L : L p ] = Γ p. exp(a L p ) ind(a L p ) p exp(a L p ). exp(a L p ) exp(a) p exp(a). 37
38 D 1, D 2 D(K), deg(d 1 ) deg(d 2 ) D 1 D 2. d 1 = deg(d 1 ), d 2 = deg(d 2 ). D 1 D 2 = Mn (D), D D(K). n = 1. D 3 D(K) D 1 D = M r (D 3 ), M d 2 1 (D 2 ) = Md 2 1 (K) D 2 = D 1 D 1 D 2 = D 1 M n (D) = M nr (D 3 ), D 2 = D3 d 2 1 = nr. n d2 1. n d2 2 n = D D(K), d = deg(d) d = p e 1 1 pe 2 2 pe l l D 1,, D l D(K). D = D 1 D 2 D l, deg(d i ) = p e i i, i = 1, 2, l. D 1,, D l. d = d 1 d 2, d 1 d 2,, D 1, D 2 D(K) D = D 1 D 2, deg(d 1 ) = d 1, deg(d 2 ) = d 2,. q 1, q 2 Z d 1 q 1 + d 2 q 2 = 1. Br(K), [D] d 2q 2 = [D 1 ], [D] d 1q 1 = [D 2 ] D 1, D 2 D(K). D 1, D 2. [D 1 D 2 ] = [D] d 2q 2 +d 1 q 1 = [D]. deg(d) = ind(d) = d, [D 1 ] d 1 = [D] d 1d 2 q 2 = [D] dq 2 = [1]. exp(d 1 ) d 1. exp(d 2 ) d 2. d 1 d 2 exp(d 1 ) exp(d 2 ). ind(d 1 ) = deg(d 1 ) ind(d 2 ) = deg(d 2 ). D 1 D 2, [D] = [D 1 D 2 ] D = D 1 D , Brauer.. 1,. 38
39 19 (Wedderburn, Albert, Dickson) D D(K), deg D {2, 3, 4, 6, 12} D. 20 (Frobenius, Hasse, Tsen, Wedderburn, Witt) K. C, C(t), C((t)). R, R(t), R((t)).. ().. D D(K).,. 21 (Brussel) K Q Q(t) Q Q((t)). D(K),. 2 D D(K) deg D = p, D. p = 2, 3. p = 5. D D(K), D = D 1 D 2 K D 1, K D 2 D(K) decomposable, indecomposable., D indecomposable, deg(d)., p p e p d, exp(d) = p e, deg(d) = p d indecomposable. 22 (Albert, Jacob) (p e, p d ) (2, 4), K indecomposable D D(K) exp(d) = p e, deg(d) = p d.,, exp(d) = 2, deg(d) = 4,. 39
40 10 Merkurjev-Suslin 10.1 Milnor K K K K 1 (K). l : K K 1 (K) : a l(a). l(1) = 0, l(a n ) = nl(a), l(ab) = l(a) + l(b) (n Z, a, b K ). K 1 (K) Z, TK 1 (K). TK 1 (K) = T n K 1 (K), n=0 T 0 K 1 (K) = Z, T n K 1 (K) = K 1 (K) Z Z K 1 (K). TK 1 (K) J = {l(a) l(1 a) a K, a 1}, TK 1 (K)/J. π : TK 1 (K) TK 1 (K)/J, K n (K) = π(t n K 1 (K)), {a 1,, a n } = π(l(a 1 ) l(a n )).. TK 1 (K)/J = K n (K) n=0 K n (K) K Milnor K. K 2 (K), (M1) {ab, c} = {a, c} + {b, c}, {a, bc} = {a, b} + {a, c}. (M2) {a, 1 a} = 0, (a 1). (M3) {a, 1} = {1, a} = 0, {a, b 1 } = {a, b}.. 31 a, b K. (M4) {a, a} = 0. (M5) {a, b} = {b, a}. 40
41 10.2 Merkurjev-Suslin n. Br(K) n = {[A] Br(K) [A] n = [1]} 23 (Merkurjev-Suslin) (n, ch(k)) = 1, µ n K. 1 n ζ µ n. ( ) a, b K 2 (K) Br(K) : {a, b} (a, b) n = K, ζ well-definied, nk 2 (K), Br(K) n.. K 2 (K)/nK 2 (K) = Br(K) n 14 (n, ch(k)) = 1 µ n K. A A cs (K) exp(a) n, a 1, b 1,, a r, b r K. A (a 1, b 1 ) n (a r, b r ) n 15 ch(k) 2. A A cs (K), exp(a) = 2, a 1, b 1,, a r, b r K. A (a 1, b 1 ) 2 (a r, b r ) 2 41
42 11 Brauer 11.1 D. D. v : D R v. (VA1) v(x) 0, (x D), v(x) = 0 x = 0. (VA2) v(xy) = v(x)v(y), (x, y D). (VA3) v(x + y) max(v(x), v(y)), (x, y D)., v(x) = 1, (x D ). v D, O v = {x D v(x) 1}. v. O v., O v, O v = {x D v(x) = 1} P v = {x O v v(x) < 1}., O v /P v v. v, D δ v δ v (x, y) = v(x y), (x, y D). D. δ v D D v. D v, v D v. D = D v v. v w D. r > 0, w = v r, v w, v w.., v w O v O w P v P w (D, δ v ) (D, δ w ) v(d ) R v. ϖ O v, v(d ) = {v(ϖ) n n Z}. ϖ O v. v. D O v v, O v /P v 42
43 v D (D ). O D, P D, f D = O D /P D. K v, K D K. v D w, D. e(d/k) = [w(d ) : v(k )], f(d/k) = [f D : f K ]. [D : K] = e(d/k)f(d/k). K v, L/K. L. e(l/k) = 1, L/K. n, K n /K, [K n : K] = n. q = [O v : P v ], K n = K(µ q n 1). K n /K. Frobenius K, K n /K Γ Kn/K, f Kn /f K Γ fkn /f K. Γ Kn/K Γ fkn /f K : σ σ OKn. f K = Fq, f Kn = Fq n, Γ fk n /f K σ : f Kn f Kn : x x q. σ Γ Kn /K σ n, K n /K Frobenius Brauer 24 K, D D(K), deg D = d. D d K d /K e(d/k) = f(d/k) = [K d, K]. K d D, D. 25 K, ϖ K. θ K : Q/Z Br(K) : m n mod Z [(K n /K, σ n, ϖ m )]. 43
44 12 Brauer 12.1 K/Q. K V K,f. V K,f. V K,f v. v V K,f, K v K δ v. K v., θ v : Br(K v ) Q/Z. K C Hom(K, C). Hom(K, C) φ ψ φ(x) = ψ(x) (x K), V K,. V K, K. w V K,, w ψ w Hom(K, C). K w = ψ w (K) C. ψ w, K w = R K w = C. K w = R w, K w = C w. V K,1, V K,2. [K : Q] = (V K,1 ) + 2 (V K,2 ). w V K,1, θ w : Br(K w ) = Br(R) 1 2 Z/Z : θ w([a]) =. V K = V K, V K,f. V K K. { 1/2 + Z ([A] = [H]) 0 + Z ([A] = [1]) 12.2 K/Q. A A cs (K) v V K A v = A K K v. ([A v ]) v VK v V K Br(K v ) 44
45 ,. Br(K) v V K Br(K v ) : [A] ([A v ]) v VK 26 (Albert-Hasse-Brauer-Noether) A A cs (K), deg A = n. (1) v V K, A v = Mn (K v ). ([A v ]) v VK v V K Br(K v ). (2) v V K A v = Mn (K v ) A = M n (K). Br(K) v V K Br(K v ). (3). 1 Br(K) v V K Br(K v ) Br(K) = (α v) v V K Q/Z P θv Q/Z 1 α v = 0, α w 1 2 Z/Z (w V K,1), α w = 0 (w V K,2 ). v V K 27 A A cs (K) ind(a) = exp(a). 45
46 13 Hausdorff-Banach-Tarski Paradox Brauer-Severi 46
47 , Bourbaki ( ) van der Waerden ( III).., (associative ring), [1] Rowen, Ring Theory I & II, Academic Press, 1988 [2] Pierce, Associative Algebras, Springer Verlag, [1],. [2], [1]. Wedderburn,,, [1],, [2]. [3] Herstein, Noncommutative Rings, The Mathematical Association of America, 1968., Brauer, [4] Draxl, Skew Fields, Cambridge University Press, 1983 [5] Farb & Dennis, Noncommutative Algebra, Springer Verlag, 1993 [6] Jacobson, Finite-Dimensional Division Algebras over Fields, Springer Verlag, 1996 [7] Kersten, Brauergruppen von Körpern, Vieweg, Brauer, [1],[2],[4],[5], [8] Deuring, Algebren, Springer Verlag, 1968 [9] Albert, Structure of Algebras, American Mathematical Society, 1961 [10] Weil, Basic Number Theory, Springer Verlag, [11] Saltman, Lectures on Division Algebras, American Mathematical Society, [12] Platonov & Yanchevskii, Finite-Dimensional Division Algebras, in Algebra IX, Encyclopaedia of Mathematical Sciences, Springer Verlag, [12]., (order). [13] Reiner, Maximal Orders (2nd ed.), Clarendon Press, [14] Vignéra, Arithmétique des Algèbres de Quaternions, Springer Verlag, Eichler [15],,,, 21, [16] Kleinert, Units in Skew Fields, Birkhäuser, 2000., (involution), [17] Knus, Merkurjev, Rost & Tignol, The Book of Involutions, American Mathematical Society,
 X G P G (X) G BG [X, BG] S 2 2 2 S 2 2 S 2 = { (x 1, x 2, x 3 ) R 3 x 2 1 + x 2 2 + x 2 3 = 1 } R 3 S 2 S 2 v x S 2 x x v(x) T x S 2 T x S 2 S 2 x T x S 2 = { ξ R 3 x ξ } R 3 T x S 2 S 2 x x T x S 2
X G P G (X) G BG [X, BG] S 2 2 2 S 2 2 S 2 = { (x 1, x 2, x 3 ) R 3 x 2 1 + x 2 2 + x 2 3 = 1 } R 3 S 2 S 2 v x S 2 x x v(x) T x S 2 T x S 2 S 2 x T x S 2 = { ξ R 3 x ξ } R 3 T x S 2 S 2 x x T x S 2
16 B
 16 B (1) 3 (2) (3) 5 ( ) 3 : 2 3 : 3 : () 3 19 ( ) 2 ax 2 + bx + c = 0 (a 0) x = b ± b 2 4ac 2a 3, 4 5 1824 5 Contents 1. 1 2. 7 3. 13 4. 18 5. 22 6. 25 7. 27 8. 31 9. 37 10. 46 11. 50 12. 56 i 1 1. 1.1..
16 B (1) 3 (2) (3) 5 ( ) 3 : 2 3 : 3 : () 3 19 ( ) 2 ax 2 + bx + c = 0 (a 0) x = b ± b 2 4ac 2a 3, 4 5 1824 5 Contents 1. 1 2. 7 3. 13 4. 18 5. 22 6. 25 7. 27 8. 31 9. 37 10. 46 11. 50 12. 56 i 1 1. 1.1..
2 (2016 3Q N) c = o (11) Ax = b A x = c A n I n n n 2n (A I n ) (I n X) A A X A n A A A (1) (2) c 0 c (3) c A A i j n 1 ( 1) i+j A (i, j) A (i, j) ã i
 [ ] (2016 3Q N) a 11 a 1n m n A A = a m1 a mn A a 1 A A = a n (1) A (a i a j, i j ) (2) A (a i ca i, c 0, i ) (3) A (a i a i + ca j, j i, i ) A 1 A 11 0 A 12 0 0 A 1k 0 1 A 22 0 0 A 2k 0 1 0 A 3k 1 A rk
[ ] (2016 3Q N) a 11 a 1n m n A A = a m1 a mn A a 1 A A = a n (1) A (a i a j, i j ) (2) A (a i ca i, c 0, i ) (3) A (a i a i + ca j, j i, i ) A 1 A 11 0 A 12 0 0 A 1k 0 1 A 22 0 0 A 2k 0 1 0 A 3k 1 A rk
II A A441 : October 02, 2014 Version : Kawahira, Tomoki TA (Kondo, Hirotaka )
 II 214-1 : October 2, 214 Version : 1.1 Kawahira, Tomoki TA (Kondo, Hirotaka ) http://www.math.nagoya-u.ac.jp/~kawahira/courses/14w-biseki.html pdf 1 2 1 9 1 16 1 23 1 3 11 6 11 13 11 2 11 27 12 4 12 11
II 214-1 : October 2, 214 Version : 1.1 Kawahira, Tomoki TA (Kondo, Hirotaka ) http://www.math.nagoya-u.ac.jp/~kawahira/courses/14w-biseki.html pdf 1 2 1 9 1 16 1 23 1 3 11 6 11 13 11 2 11 27 12 4 12 11
newmain.dvi
 数論 サンプルページ この本の定価 判型などは, 以下の URL からご覧いただけます. http://www.morikita.co.jp/books/mid/008142 このサンプルページの内容は, 第 2 版 1 刷発行当時のものです. Daniel DUVERNEY: THÉORIE DES NOMBRES c Dunod, Paris, 1998, This book is published
数論 サンプルページ この本の定価 判型などは, 以下の URL からご覧いただけます. http://www.morikita.co.jp/books/mid/008142 このサンプルページの内容は, 第 2 版 1 刷発行当時のものです. Daniel DUVERNEY: THÉORIE DES NOMBRES c Dunod, Paris, 1998, This book is published
数学Ⅱ演習(足助・09夏)
 II I 9/4/4 9/4/2 z C z z z z, z 2 z, w C zw z w 3 z, w C z + w z + w 4 t R t C t t t t t z z z 2 z C re z z + z z z, im z 2 2 3 z C e z + z + 2 z2 + 3! z3 + z!, I 4 x R e x cos x + sin x 2 z, w C e z+w
II I 9/4/4 9/4/2 z C z z z z, z 2 z, w C zw z w 3 z, w C z + w z + w 4 t R t C t t t t t z z z 2 z C re z z + z z z, im z 2 2 3 z C e z + z + 2 z2 + 3! z3 + z!, I 4 x R e x cos x + sin x 2 z, w C e z+w
ver Web
 ver201723 Web 1 4 11 4 12 5 13 7 2 9 21 9 22 10 23 10 24 11 3 13 31 n 13 32 15 33 21 34 25 35 (1) 27 4 30 41 30 42 32 43 36 44 (2) 38 45 45 46 45 5 46 51 46 52 48 53 49 54 51 55 54 56 58 57 (3) 61 2 3
ver201723 Web 1 4 11 4 12 5 13 7 2 9 21 9 22 10 23 10 24 11 3 13 31 n 13 32 15 33 21 34 25 35 (1) 27 4 30 41 30 42 32 43 36 44 (2) 38 45 45 46 45 5 46 51 46 52 48 53 49 54 51 55 54 56 58 57 (3) 61 2 3
(2018 2Q C) [ ] R 2 2 P = (a, b), Q = (c, d) Q P QP = ( ) a c b d (a c, b d) P = (a, b) O P ( ) a p = b P = (a, b) p = ( ) a b R 2 {( ) } R 2 x = x, y
![(2018 2Q C) [ ] R 2 2 P = (a, b), Q = (c, d) Q P QP = ( ) a c b d (a c, b d) P = (a, b) O P ( ) a p = b P = (a, b) p = ( ) a b R 2 {( ) } R 2 x = x, y (2018 2Q C) [ ] R 2 2 P = (a, b), Q = (c, d) Q P QP = ( ) a c b d (a c, b d) P = (a, b) O P ( ) a p = b P = (a, b) p = ( ) a b R 2 {( ) } R 2 x = x, y](/thumbs/101/151465237.jpg) (2018 2Q C) [ ] R 2 2 P = (a, b), Q = (c, d) Q P QP = a c b d (a c, b d) P = (a, b) O P a p = b P = (a, b) p = a b R 2 { } R 2 x = x, y R y 2 a p =, c q = b d p + a + c q = b + d q p P q a p = c R c b
(2018 2Q C) [ ] R 2 2 P = (a, b), Q = (c, d) Q P QP = a c b d (a c, b d) P = (a, b) O P a p = b P = (a, b) p = a b R 2 { } R 2 x = x, y R y 2 a p =, c q = b d p + a + c q = b + d q p P q a p = c R c b
v v = v 1 v 2 v 3 (1) R = (R ij ) (2) R (R 1 ) ij = R ji (3) 3 R ij R ik = δ jk (4) i=1 δ ij Kronecker δ ij = { 1 (i = j) 0 (i
 1. 1 1.1 1.1.1 1.1.1.1 v v = v 1 v 2 v 3 (1) R = (R ij ) (2) R (R 1 ) ij = R ji (3) R ij R ik = δ jk (4) δ ij Kronecker δ ij = { 1 (i = j) 0 (i j) (5) 1 1.1. v1.1 2011/04/10 1. 1 2 v i = R ij v j (6) [
1. 1 1.1 1.1.1 1.1.1.1 v v = v 1 v 2 v 3 (1) R = (R ij ) (2) R (R 1 ) ij = R ji (3) R ij R ik = δ jk (4) δ ij Kronecker δ ij = { 1 (i = j) 0 (i j) (5) 1 1.1. v1.1 2011/04/10 1. 1 2 v i = R ij v j (6) [
(2016 2Q H) [ ] R 2 2 P = (a, b), Q = (c, d) Q P QP = ( ) a c b d (a c, b d) P = (a, b) O P ( ) a p = b P = (a, b) p = ( ) a b R 2 {( ) } R 2 x = x, y
![(2016 2Q H) [ ] R 2 2 P = (a, b), Q = (c, d) Q P QP = ( ) a c b d (a c, b d) P = (a, b) O P ( ) a p = b P = (a, b) p = ( ) a b R 2 {( ) } R 2 x = x, y (2016 2Q H) [ ] R 2 2 P = (a, b), Q = (c, d) Q P QP = ( ) a c b d (a c, b d) P = (a, b) O P ( ) a p = b P = (a, b) p = ( ) a b R 2 {( ) } R 2 x = x, y](/thumbs/98/136512264.jpg) (2016 2Q H) [ ] R 2 2 P = (a, b), Q = (c, d) Q P QP = a c b d (a c, b d) P = (a, b) O P a p = b P = (a, b) p = a b R 2 { } R 2 x = x, y R y 2 a p =, c q = b d p + a + c q = b + d q p P q a p = c R c b
(2016 2Q H) [ ] R 2 2 P = (a, b), Q = (c, d) Q P QP = a c b d (a c, b d) P = (a, b) O P a p = b P = (a, b) p = a b R 2 { } R 2 x = x, y R y 2 a p =, c q = b d p + a + c q = b + d q p P q a p = c R c b
 i I II I II II IC IIC I II ii 5 8 5 3 7 8 iii I 3........................... 5......................... 7........................... 4........................ 8.3......................... 33.4...................
i I II I II II IC IIC I II ii 5 8 5 3 7 8 iii I 3........................... 5......................... 7........................... 4........................ 8.3......................... 33.4...................
30
 3 ............................................2 2...........................................2....................................2.2...................................2.3..............................
3 ............................................2 2...........................................2....................................2.2...................................2.3..............................
D 24 D D D
 5 Paper I.R. 2001 5 Paper HP Paper 5 3 5.1................................................... 3 5.2.................................................... 4 5.3.......................................... 6
5 Paper I.R. 2001 5 Paper HP Paper 5 3 5.1................................................... 3 5.2.................................................... 4 5.3.......................................... 6
,. Black-Scholes u t t, x c u 0 t, x x u t t, x c u t, x x u t t, x + σ x u t, x + rx ut, x rux, t 0 x x,,.,. Step 3, 7,,, Step 6., Step 4,. Step 5,,.
 9 α ν β Ξ ξ Γ γ o δ Π π ε ρ ζ Σ σ η τ Θ θ Υ υ ι Φ φ κ χ Λ λ Ψ ψ µ Ω ω Def, Prop, Th, Lem, Note, Remark, Ex,, Proof, R, N, Q, C [a, b {x R : a x b} : a, b {x R : a < x < b} : [a, b {x R : a x < b} : a,
9 α ν β Ξ ξ Γ γ o δ Π π ε ρ ζ Σ σ η τ Θ θ Υ υ ι Φ φ κ χ Λ λ Ψ ψ µ Ω ω Def, Prop, Th, Lem, Note, Remark, Ex,, Proof, R, N, Q, C [a, b {x R : a x b} : a, b {x R : a < x < b} : [a, b {x R : a x < b} : a,
untitled
 0. =. =. (999). 3(983). (980). (985). (966). 3. := :=. A A. A A. := := 4 5 A B A B A B. A = B A B A B B A. A B A B, A B, B. AP { A, P } = { : A, P } = { A P }. A = {0, }, A, {0, }, {0}, {}, A {0}, {}.
0. =. =. (999). 3(983). (980). (985). (966). 3. := :=. A A. A A. := := 4 5 A B A B A B. A = B A B A B B A. A B A B, A B, B. AP { A, P } = { : A, P } = { A P }. A = {0, }, A, {0, }, {0}, {}, A {0}, {}.
 13 0 1 1 4 11 4 12 5 13 6 2 10 21 10 22 14 3 20 31 20 32 25 33 28 4 31 41 32 42 34 43 38 5 41 51 41 52 43 53 54 6 57 61 57 62 60 70 0 Gauss a, b, c x, y f(x, y) = ax 2 + bxy + cy 2 = x y a b/2 b/2 c x
13 0 1 1 4 11 4 12 5 13 6 2 10 21 10 22 14 3 20 31 20 32 25 33 28 4 31 41 32 42 34 43 38 5 41 51 41 52 43 53 54 6 57 61 57 62 60 70 0 Gauss a, b, c x, y f(x, y) = ax 2 + bxy + cy 2 = x y a b/2 b/2 c x
医系の統計入門第 2 版 サンプルページ この本の定価 判型などは, 以下の URL からご覧いただけます. このサンプルページの内容は, 第 2 版 1 刷発行時のものです.
 医系の統計入門第 2 版 サンプルページ この本の定価 判型などは, 以下の URL からご覧いただけます. http://www.morikita.co.jp/books/mid/009192 このサンプルページの内容は, 第 2 版 1 刷発行時のものです. i 2 t 1. 2. 3 2 3. 6 4. 7 5. n 2 ν 6. 2 7. 2003 ii 2 2013 10 iii 1987
医系の統計入門第 2 版 サンプルページ この本の定価 判型などは, 以下の URL からご覧いただけます. http://www.morikita.co.jp/books/mid/009192 このサンプルページの内容は, 第 2 版 1 刷発行時のものです. i 2 t 1. 2. 3 2 3. 6 4. 7 5. n 2 ν 6. 2 7. 2003 ii 2 2013 10 iii 1987
II No.01 [n/2] [1]H n (x) H n (x) = ( 1) r n! r!(n 2r)! (2x)n 2r. r=0 [2]H n (x) n,, H n ( x) = ( 1) n H n (x). [3] H n (x) = ( 1) n dn x2 e dx n e x2
![II No.01 [n/2] [1]H n (x) H n (x) = ( 1) r n! r!(n 2r)! (2x)n 2r. r=0 [2]H n (x) n,, H n ( x) = ( 1) n H n (x). [3] H n (x) = ( 1) n dn x2 e dx n e x2 II No.01 [n/2] [1]H n (x) H n (x) = ( 1) r n! r!(n 2r)! (2x)n 2r. r=0 [2]H n (x) n,, H n ( x) = ( 1) n H n (x). [3] H n (x) = ( 1) n dn x2 e dx n e x2](/thumbs/101/149159646.jpg) II No.1 [n/] [1]H n x) H n x) = 1) r n! r!n r)! x)n r r= []H n x) n,, H n x) = 1) n H n x) [3] H n x) = 1) n dn x e dx n e x [4] H n+1 x) = xh n x) nh n 1 x) ) d dx x H n x) = H n+1 x) d dx H nx) = nh
II No.1 [n/] [1]H n x) H n x) = 1) r n! r!n r)! x)n r r= []H n x) n,, H n x) = 1) n H n x) [3] H n x) = 1) n dn x e dx n e x [4] H n+1 x) = xh n x) nh n 1 x) ) d dx x H n x) = H n+1 x) d dx H nx) = nh
 n ξ n,i, i = 1,, n S n ξ n,i n 0 R 1,.. σ 1 σ i .10.14.15 0 1 0 1 1 3.14 3.18 3.19 3.14 3.14,. ii 1 1 1.1..................................... 1 1............................... 3 1.3.........................
n ξ n,i, i = 1,, n S n ξ n,i n 0 R 1,.. σ 1 σ i .10.14.15 0 1 0 1 1 3.14 3.18 3.19 3.14 3.14,. ii 1 1 1.1..................................... 1 1............................... 3 1.3.........................
linearal1.dvi
 19 4 30 I 1 1 11 1 12 2 13 3 131 3 132 4 133 5 134 6 14 7 2 9 21 9 211 9 212 10 213 13 214 14 22 15 221 15 222 16 223 17 224 20 3 21 31 21 32 21 33 22 34 23 341 23 342 24 343 27 344 29 35 31 351 31 352
19 4 30 I 1 1 11 1 12 2 13 3 131 3 132 4 133 5 134 6 14 7 2 9 21 9 211 9 212 10 213 13 214 14 22 15 221 15 222 16 223 17 224 20 3 21 31 21 32 21 33 22 34 23 341 23 342 24 343 27 344 29 35 31 351 31 352
Part () () Γ Part ,
 Contents a 6 6 6 6 6 6 6 7 7. 8.. 8.. 8.3. 8 Part. 9. 9.. 9.. 3. 3.. 3.. 3 4. 5 4.. 5 4.. 9 4.3. 3 Part. 6 5. () 6 5.. () 7 5.. 9 5.3. Γ 3 6. 3 6.. 3 6.. 3 6.3. 33 Part 3. 34 7. 34 7.. 34 7.. 34 8. 35
Contents a 6 6 6 6 6 6 6 7 7. 8.. 8.. 8.3. 8 Part. 9. 9.. 9.. 3. 3.. 3.. 3 4. 5 4.. 5 4.. 9 4.3. 3 Part. 6 5. () 6 5.. () 7 5.. 9 5.3. Γ 3 6. 3 6.. 3 6.. 3 6.3. 33 Part 3. 34 7. 34 7.. 34 7.. 34 8. 35
Jacobson Prime Avoidance
 2016 2017 2 22 1 1 3 2 4 2.1 Jacobson................. 4 2.2.................... 5 3 6 3.1 Prime Avoidance....................... 7 3.2............................. 8 3.3..............................
2016 2017 2 22 1 1 3 2 4 2.1 Jacobson................. 4 2.2.................... 5 3 6 3.1 Prime Avoidance....................... 7 3.2............................. 8 3.3..............................
I ( ) 1 de Broglie 1 (de Broglie) p λ k h Planck ( Js) p = h λ = k (1) h 2π : Dirac k B Boltzmann ( J/K) T U = 3 2 k BT
 I (008 4 0 de Broglie (de Broglie p λ k h Planck ( 6.63 0 34 Js p = h λ = k ( h π : Dirac k B Boltzmann (.38 0 3 J/K T U = 3 k BT ( = λ m k B T h m = 0.067m 0 m 0 = 9. 0 3 kg GaAs( a T = 300 K 3 fg 07345
I (008 4 0 de Broglie (de Broglie p λ k h Planck ( 6.63 0 34 Js p = h λ = k ( h π : Dirac k B Boltzmann (.38 0 3 J/K T U = 3 k BT ( = λ m k B T h m = 0.067m 0 m 0 = 9. 0 3 kg GaAs( a T = 300 K 3 fg 07345
 (u(x)v(x)) = u (x)v(x) + u(x)v (x) ( ) u(x) = u (x)v(x) u(x)v (x) v(x) v(x) 2 y = g(t), t = f(x) y = g(f(x)) dy dx dy dx = dy dt dt dx., y, f, g y = f (g(x))g (x). ( (f(g(x)). ). [ ] y = e ax+b (a, b )
(u(x)v(x)) = u (x)v(x) + u(x)v (x) ( ) u(x) = u (x)v(x) u(x)v (x) v(x) v(x) 2 y = g(t), t = f(x) y = g(f(x)) dy dx dy dx = dy dt dt dx., y, f, g y = f (g(x))g (x). ( (f(g(x)). ). [ ] y = e ax+b (a, b )
A11 (1993,1994) 29 A12 (1994) 29 A13 Trefethen and Bau Numerical Linear Algebra (1997) 29 A14 (1999) 30 A15 (2003) 30 A16 (2004) 30 A17 (2007) 30 A18
 2013 8 29y, 2016 10 29 1 2 2 Jordan 3 21 3 3 Jordan (1) 3 31 Jordan 4 32 Jordan 4 33 Jordan 6 34 Jordan 8 35 9 4 Jordan (2) 10 41 x 11 42 x 12 43 16 44 19 441 19 442 20 443 25 45 25 5 Jordan 26 A 26 A1
2013 8 29y, 2016 10 29 1 2 2 Jordan 3 21 3 3 Jordan (1) 3 31 Jordan 4 32 Jordan 4 33 Jordan 6 34 Jordan 8 35 9 4 Jordan (2) 10 41 x 11 42 x 12 43 16 44 19 441 19 442 20 443 25 45 25 5 Jordan 26 A 26 A1
No δs δs = r + δr r = δr (3) δs δs = r r = δr + u(r + δr, t) u(r, t) (4) δr = (δx, δy, δz) u i (r + δr, t) u i (r, t) = u i x j δx j (5) δs 2
 No.2 1 2 2 δs δs = r + δr r = δr (3) δs δs = r r = δr + u(r + δr, t) u(r, t) (4) δr = (δx, δy, δz) u i (r + δr, t) u i (r, t) = u i δx j (5) δs 2 = δx i δx i + 2 u i δx i δx j = δs 2 + 2s ij δx i δx j
No.2 1 2 2 δs δs = r + δr r = δr (3) δs δs = r r = δr + u(r + δr, t) u(r, t) (4) δr = (δx, δy, δz) u i (r + δr, t) u i (r, t) = u i δx j (5) δs 2 = δx i δx i + 2 u i δx i δx j = δs 2 + 2s ij δx i δx j
II 2 3.,, A(B + C) = AB + AC, (A + B)C = AC + BC. 4. m m A, m m B,, m m B, AB = BA, A,, I. 5. m m A, m n B, AB = B, A I E, 4 4 I, J, K
 II. () 7 F 7 = { 0,, 2, 3, 4, 5, 6 }., F 7 a, b F 7, a b, F 7,. (a) a, b,,. (b) 7., 4 5 = 20 = 2 7 + 6, 4 5 = 6 F 7., F 7,., 0 a F 7, ab = F 7 b F 7. (2) 7, 6 F 6 = { 0,, 2, 3, 4, 5 },,., F 6., 0 0 a F
II. () 7 F 7 = { 0,, 2, 3, 4, 5, 6 }., F 7 a, b F 7, a b, F 7,. (a) a, b,,. (b) 7., 4 5 = 20 = 2 7 + 6, 4 5 = 6 F 7., F 7,., 0 a F 7, ab = F 7 b F 7. (2) 7, 6 F 6 = { 0,, 2, 3, 4, 5 },,., F 6., 0 0 a F
S I. dy fx x fx y fx + C 3 C dy fx 4 x, y dy v C xt y C v e kt k > xt yt gt [ v dt dt v e kt xt v e kt + C k x v + C C k xt v k 3 r r + dr e kt S dt d
 S I.. http://ayapin.film.s.dendai.ac.jp/~matuda /TeX/lecture.html PDF PS.................................... 3.3.................... 9.4................5.............. 3 5. Laplace................. 5....
S I.. http://ayapin.film.s.dendai.ac.jp/~matuda /TeX/lecture.html PDF PS.................................... 3.3.................... 9.4................5.............. 3 5. Laplace................. 5....
 20 9 19 1 3 11 1 3 111 3 112 1 4 12 6 121 6 122 7 13 7 131 8 132 10 133 10 134 12 14 13 141 13 142 13 143 15 144 16 145 17 15 19 151 1 19 152 20 2 21 21 21 211 21 212 1 23 213 1 23 214 25 215 31 22 33
20 9 19 1 3 11 1 3 111 3 112 1 4 12 6 121 6 122 7 13 7 131 8 132 10 133 10 134 12 14 13 141 13 142 13 143 15 144 16 145 17 15 19 151 1 19 152 20 2 21 21 21 211 21 212 1 23 213 1 23 214 25 215 31 22 33
AI n Z f n : Z Z f n (k) = nk ( k Z) f n n 1.9 R R f : R R f 1 1 {a R f(a) = 0 R = {0 R 1.10 R R f : R R f 1 : R R 1.11 Z Z id Z 1.12 Q Q id
 1 1.1 1.1 R R (1) R = 1 2 Z = 2 n Z (2) R 1.2 R C Z R 1.3 Z 2 = {(a, b) a Z, b Z Z 2 a, b, c, d Z (a, b) + (c, d) = (a + c, b + d), (a, b)(c, d) = (ac, bd) (1) Z 2 (2) Z 2? (3) Z 2 1.4 C Q[ 1] = {a + bi
1 1.1 1.1 R R (1) R = 1 2 Z = 2 n Z (2) R 1.2 R C Z R 1.3 Z 2 = {(a, b) a Z, b Z Z 2 a, b, c, d Z (a, b) + (c, d) = (a + c, b + d), (a, b)(c, d) = (ac, bd) (1) Z 2 (2) Z 2? (3) Z 2 1.4 C Q[ 1] = {a + bi
S K(S) = T K(T ) T S K n (1.1) n {}}{ n K n (1.1) 0 K 0 0 K Q p K Z/pZ L K (1) L K L K (2) K L L K [L : K] 1.1.
![S K(S) = T K(T ) T S K n (1.1) n {}}{ n K n (1.1) 0 K 0 0 K Q p K Z/pZ L K (1) L K L K (2) K L L K [L : K] 1.1. S K(S) = T K(T ) T S K n (1.1) n {}}{ n K n (1.1) 0 K 0 0 K Q p K Z/pZ L K (1) L K L K (2) K L L K [L : K] 1.1.](/thumbs/91/105754396.jpg) () 1.1.. 1. 1.1. (1) L K (i) 0 K 1 K (ii) x, y K x + y K, x y K (iii) x, y K xy K (iv) x K \ {0} x 1 K K L L K ( 0 L 1 L ) L K L/K (2) K M L M K L 1.1. C C 1.2. R K = {a + b 3 i a, b Q} Q( 2, 3) = Q( 2
() 1.1.. 1. 1.1. (1) L K (i) 0 K 1 K (ii) x, y K x + y K, x y K (iii) x, y K xy K (iv) x K \ {0} x 1 K K L L K ( 0 L 1 L ) L K L/K (2) K M L M K L 1.1. C C 1.2. R K = {a + b 3 i a, b Q} Q( 2, 3) = Q( 2
1. 2 P 2 (x, y) 2 x y (0, 0) R 2 = {(x, y) x, y R} x, y R P = (x, y) O = (0, 0) OP ( ) OP x x, y y ( ) x v = y ( ) x 2 1 v = P = (x, y) y ( x y ) 2 (x
 . P (, (0, 0 R {(,, R}, R P (, O (0, 0 OP OP, v v P (, ( (, (, { R, R} v (, (, (,, z 3 w z R 3,, z R z n R n.,..., n R n n w, t w ( z z Ke Words:. A P 3 0 B P 0 a. A P b B P 3. A π/90 B a + b c π/ 3. +
. P (, (0, 0 R {(,, R}, R P (, O (0, 0 OP OP, v v P (, ( (, (, { R, R} v (, (, (,, z 3 w z R 3,, z R z n R n.,..., n R n n w, t w ( z z Ke Words:. A P 3 0 B P 0 a. A P b B P 3. A π/90 B a + b c π/ 3. +
 20 6 4 1 4 1.1 1.................................... 4 1.1.1.................................... 4 1.1.2 1................................ 5 1.2................................... 7 1.2.1....................................
20 6 4 1 4 1.1 1.................................... 4 1.1.1.................................... 4 1.1.2 1................................ 5 1.2................................... 7 1.2.1....................................
B ver B
 B ver. 2017.02.24 B Contents 1 11 1.1....................... 11 1.1.1............. 11 1.1.2.......................... 12 1.2............................. 14 1.2.1................ 14 1.2.2.......................
B ver. 2017.02.24 B Contents 1 11 1.1....................... 11 1.1.1............. 11 1.1.2.......................... 12 1.2............................. 14 1.2.1................ 14 1.2.2.......................
W u = u(x, t) u tt = a 2 u xx, a > 0 (1) D := {(x, t) : 0 x l, t 0} u (0, t) = 0, u (l, t) = 0, t 0 (2)
 3 215 4 27 1 1 u u(x, t) u tt a 2 u xx, a > (1) D : {(x, t) : x, t } u (, t), u (, t), t (2) u(x, ) f(x), u(x, ) t 2, x (3) u(x, t) X(x)T (t) u (1) 1 T (t) a 2 T (t) X (x) X(x) α (2) T (t) αa 2 T (t) (4)
3 215 4 27 1 1 u u(x, t) u tt a 2 u xx, a > (1) D : {(x, t) : x, t } u (, t), u (, t), t (2) u(x, ) f(x), u(x, ) t 2, x (3) u(x, t) X(x)T (t) u (1) 1 T (t) a 2 T (t) X (x) X(x) α (2) T (t) αa 2 T (t) (4)
(3) (2),,. ( 20) ( s200103) 0.7 x C,, x 2 + y 2 + ax = 0 a.. D,. D, y C, C (x, y) (y 0) C m. (2) D y = y(x) (x ± y 0), (x, y) D, m, m = 1., D. (x 2 y
 [ ] 7 0.1 2 2 + y = t sin t IC ( 9) ( s090101) 0.2 y = d2 y 2, y = x 3 y + y 2 = 0 (2) y + 2y 3y = e 2x 0.3 1 ( y ) = f x C u = y x ( 15) ( s150102) [ ] y/x du x = Cexp f(u) u (2) x y = xey/x ( 16) ( s160101)
[ ] 7 0.1 2 2 + y = t sin t IC ( 9) ( s090101) 0.2 y = d2 y 2, y = x 3 y + y 2 = 0 (2) y + 2y 3y = e 2x 0.3 1 ( y ) = f x C u = y x ( 15) ( s150102) [ ] y/x du x = Cexp f(u) u (2) x y = xey/x ( 16) ( s160101)
S I. dy fx x fx y fx + C 3 C vt dy fx 4 x, y dy yt gt + Ct + C dt v e kt xt v e kt + C k x v k + C C xt v k 3 r r + dr e kt S Sr πr dt d v } dt k e kt
 S I. x yx y y, y,. F x, y, y, y,, y n http://ayapin.film.s.dendai.ac.jp/~matuda n /TeX/lecture.html PDF PS yx.................................... 3.3.................... 9.4................5..............
S I. x yx y y, y,. F x, y, y, y,, y n http://ayapin.film.s.dendai.ac.jp/~matuda n /TeX/lecture.html PDF PS yx.................................... 3.3.................... 9.4................5..............
2012 A, N, Z, Q, R, C
 2012 A, N, Z, Q, R, C 1 2009 9 2 2011 2 3 2012 9 1 2 2 5 3 11 4 16 5 22 6 25 7 29 8 32 1 1 1.1 3 1 1 1 1 1 1? 3 3 3 3 3 3 3 1 1, 1 1 + 1 1 1+1 2 2 1 2+1 3 2 N 1.2 N (i) 2 a b a 1 b a < b a b b a a b (ii)
2012 A, N, Z, Q, R, C 1 2009 9 2 2011 2 3 2012 9 1 2 2 5 3 11 4 16 5 22 6 25 7 29 8 32 1 1 1.1 3 1 1 1 1 1 1? 3 3 3 3 3 3 3 1 1, 1 1 + 1 1 1+1 2 2 1 2+1 3 2 N 1.2 N (i) 2 a b a 1 b a < b a b b a a b (ii)
 2019 1 5 0 3 1 4 1.1.................... 4 1.1.1......................... 4 1.1.2........................ 5 1.1.3................... 5 1.1.4........................ 6 1.1.5......................... 6 1.2..........................
2019 1 5 0 3 1 4 1.1.................... 4 1.1.1......................... 4 1.1.2........................ 5 1.1.3................... 5 1.1.4........................ 6 1.1.5......................... 6 1.2..........................
量子力学 問題
 3 : 203 : 0. H = 0 0 2 6 0 () = 6, 2 = 2, 3 = 3 3 H 6 2 3 ϵ,2,3 (2) ψ = (, 2, 3 ) ψ Hψ H (3) P i = i i P P 2 = P 2 P 3 = P 3 P = O, P 2 i = P i (4) P + P 2 + P 3 = E 3 (5) i ϵ ip i H 0 0 (6) R = 0 0 [H,
3 : 203 : 0. H = 0 0 2 6 0 () = 6, 2 = 2, 3 = 3 3 H 6 2 3 ϵ,2,3 (2) ψ = (, 2, 3 ) ψ Hψ H (3) P i = i i P P 2 = P 2 P 3 = P 3 P = O, P 2 i = P i (4) P + P 2 + P 3 = E 3 (5) i ϵ ip i H 0 0 (6) R = 0 0 [H,
(1.2) T D = 0 T = D = 30 kn 1.2 (1.4) 2F W = 0 F = W/2 = 300 kn/2 = 150 kn 1.3 (1.9) R = W 1 + W 2 = = 1100 N. (1.9) W 2 b W 1 a = 0
 1 1 1.1 1.) T D = T = D = kn 1. 1.4) F W = F = W/ = kn/ = 15 kn 1. 1.9) R = W 1 + W = 6 + 5 = 11 N. 1.9) W b W 1 a = a = W /W 1 )b = 5/6) = 5 cm 1.4 AB AC P 1, P x, y x, y y x 1.4.) P sin 6 + P 1 sin 45
1 1 1.1 1.) T D = T = D = kn 1. 1.4) F W = F = W/ = kn/ = 15 kn 1. 1.9) R = W 1 + W = 6 + 5 = 11 N. 1.9) W b W 1 a = a = W /W 1 )b = 5/6) = 5 cm 1.4 AB AC P 1, P x, y x, y y x 1.4.) P sin 6 + P 1 sin 45
1 Ricci V, V i, W f : V W f f(v ) = Imf W ( ) f : V 1 V k W 1
 1 Ricci V, V i, W f : V W f f(v = Imf W ( f : V 1 V k W 1 {f(v 1,, v k v i V i } W < Imf > < > f W V, V i, W f : U V L(U; V f : V 1 V r W L(V 1,, V r ; W L(V 1,, V r ; W (f + g(v 1,, v r = f(v 1,, v r
1 Ricci V, V i, W f : V W f f(v = Imf W ( f : V 1 V k W 1 {f(v 1,, v k v i V i } W < Imf > < > f W V, V i, W f : U V L(U; V f : V 1 V r W L(V 1,, V r ; W L(V 1,, V r ; W (f + g(v 1,, v r = f(v 1,, v r
6kg 1.1m 1.m.1m.1 l λ ϵ λ l + λ l l l dl dl + dλ ϵ dλ dl dl + dλ dl dl 3 1. JIS 1 6kg 1% 66kg 1 13 σ a1 σ m σ a1 σ m σ m σ a1 f f σ a1 σ a1 σ m f 4
 35-8585 7 8 1 I I 1 1.1 6kg 1m P σ σ P 1 l l λ λ l 1.m 1 6kg 1.1m 1.m.1m.1 l λ ϵ λ l + λ l l l dl dl + dλ ϵ dλ dl dl + dλ dl dl 3 1. JIS 1 6kg 1% 66kg 1 13 σ a1 σ m σ a1 σ m σ m σ a1 f f σ a1 σ a1 σ m
35-8585 7 8 1 I I 1 1.1 6kg 1m P σ σ P 1 l l λ λ l 1.m 1 6kg 1.1m 1.m.1m.1 l λ ϵ λ l + λ l l l dl dl + dλ ϵ dλ dl dl + dλ dl dl 3 1. JIS 1 6kg 1% 66kg 1 13 σ a1 σ m σ a1 σ m σ m σ a1 f f σ a1 σ a1 σ m
n (1.6) i j=1 1 n a ij x j = b i (1.7) (1.7) (1.4) (1.5) (1.4) (1.7) u, v, w ε x, ε y, ε x, γ yz, γ zx, γ xy (1.8) ε x = u x ε y = v y ε z = w z γ yz
 1 2 (a 1, a 2, a n ) (b 1, b 2, b n ) A (1.1) A = a 1 b 1 + a 2 b 2 + + a n b n (1.1) n A = a i b i (1.2) i=1 n i 1 n i=1 a i b i n i=1 A = a i b i (1.3) (1.3) (1.3) (1.1) (ummation convention) a 11 x
1 2 (a 1, a 2, a n ) (b 1, b 2, b n ) A (1.1) A = a 1 b 1 + a 2 b 2 + + a n b n (1.1) n A = a i b i (1.2) i=1 n i 1 n i=1 a i b i n i=1 A = a i b i (1.3) (1.3) (1.3) (1.1) (ummation convention) a 11 x
, = = 7 6 = 42, =
 http://www.ss.u-tokai.ac.jp/~mahoro/2016autumn/alg_intro/ 1 1 2016.9.26, http://www.ss.u-tokai.ac.jp/~mahoro/2016autumn/alg_intro/ 1.1 1 214 132 = 28258 2 + 1 + 4 1 + 3 + 2 = 7 6 = 42, 4 + 2 = 6 2 + 8
http://www.ss.u-tokai.ac.jp/~mahoro/2016autumn/alg_intro/ 1 1 2016.9.26, http://www.ss.u-tokai.ac.jp/~mahoro/2016autumn/alg_intro/ 1.1 1 214 132 = 28258 2 + 1 + 4 1 + 3 + 2 = 7 6 = 42, 4 + 2 = 6 2 + 8
2.2 ( y = y(x ( (x 0, y 0 y (x 0 (y 0 = y(x 0 y = y(x ( y (x 0 = F (x 0, y(x 0 = F (x 0, y 0 (x 0, y 0 ( (x 0, y 0 F (x 0, y 0 xy (x, y (, F (x, y ( (
 (. x y y x f y = f(x y x y = y(x y x y dx = d dx y(x = y (x = f (x y = y(x x ( (differential equation ( + y 2 dx + xy = 0 dx = xy + y 2 2 2 x y 2 F (x, y = xy + y 2 y = y(x x x xy(x = F (x, y(x + y(x 2
(. x y y x f y = f(x y x y = y(x y x y dx = d dx y(x = y (x = f (x y = y(x x ( (differential equation ( + y 2 dx + xy = 0 dx = xy + y 2 2 2 x y 2 F (x, y = xy + y 2 y = y(x x x xy(x = F (x, y(x + y(x 2
 2 2 MATHEMATICS.PDF 200-2-0 3 2 (p n ), ( ) 7 3 4 6 5 20 6 GL 2 (Z) SL 2 (Z) 27 7 29 8 SL 2 (Z) 35 9 2 40 0 2 46 48 2 2 5 3 2 2 58 4 2 6 5 2 65 6 2 67 7 2 69 2 , a 0 + a + a 2 +... b b 2 b 3 () + b n a
2 2 MATHEMATICS.PDF 200-2-0 3 2 (p n ), ( ) 7 3 4 6 5 20 6 GL 2 (Z) SL 2 (Z) 27 7 29 8 SL 2 (Z) 35 9 2 40 0 2 46 48 2 2 5 3 2 2 58 4 2 6 5 2 65 6 2 67 7 2 69 2 , a 0 + a + a 2 +... b b 2 b 3 () + b n a
2011de.dvi
 211 ( 4 2 1. 3 1.1............................... 3 1.2 1- -......................... 13 1.3 2-1 -................... 19 1.4 3- -......................... 29 2. 37 2.1................................ 37
211 ( 4 2 1. 3 1.1............................... 3 1.2 1- -......................... 13 1.3 2-1 -................... 19 1.4 3- -......................... 29 2. 37 2.1................................ 37
all.dvi
 5,, Euclid.,..,... Euclid,.,.,, e i (i =,, ). 6 x a x e e e x.:,,. a,,. a a = a e + a e + a e = {e, e, e } a (.) = a i e i = a i e i (.) i= {a,a,a } T ( T ),.,,,,. (.),.,...,,. a 0 0 a = a 0 + a + a 0
5,, Euclid.,..,... Euclid,.,.,, e i (i =,, ). 6 x a x e e e x.:,,. a,,. a a = a e + a e + a e = {e, e, e } a (.) = a i e i = a i e i (.) i= {a,a,a } T ( T ),.,,,,. (.),.,...,,. a 0 0 a = a 0 + a + a 0
x, y x 3 y xy 3 x 2 y + xy 2 x 3 + y 3 = x 3 y xy 3 x 2 y + xy 2 x 3 + y 3 = 15 xy (x y) (x + y) xy (x y) (x y) ( x 2 + xy + y 2) = 15 (x y)
 x, y x 3 y xy 3 x 2 y + xy 2 x 3 + y 3 = 15 1 1977 x 3 y xy 3 x 2 y + xy 2 x 3 + y 3 = 15 xy (x y) (x + y) xy (x y) (x y) ( x 2 + xy + y 2) = 15 (x y) ( x 2 y + xy 2 x 2 2xy y 2) = 15 (x y) (x + y) (xy
x, y x 3 y xy 3 x 2 y + xy 2 x 3 + y 3 = 15 1 1977 x 3 y xy 3 x 2 y + xy 2 x 3 + y 3 = 15 xy (x y) (x + y) xy (x y) (x y) ( x 2 + xy + y 2) = 15 (x y) ( x 2 y + xy 2 x 2 2xy y 2) = 15 (x y) (x + y) (xy
meiji_resume_1.PDF
 β β β (q 1,q,..., q n ; p 1, p,..., p n ) H(q 1,q,..., q n ; p 1, p,..., p n ) Hψ = εψ ε k = k +1/ ε k = k(k 1) (x, y, z; p x, p y, p z ) (r; p r ), (θ; p θ ), (ϕ; p ϕ ) ε k = 1/ k p i dq i E total = E
β β β (q 1,q,..., q n ; p 1, p,..., p n ) H(q 1,q,..., q n ; p 1, p,..., p n ) Hψ = εψ ε k = k +1/ ε k = k(k 1) (x, y, z; p x, p y, p z ) (r; p r ), (θ; p θ ), (ϕ; p ϕ ) ε k = 1/ k p i dq i E total = E
ii
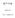 ii iii 1 1 1.1..................................... 1 1.2................................... 3 1.3........................... 4 2 9 2.1.................................. 9 2.2...............................
ii iii 1 1 1.1..................................... 1 1.2................................... 3 1.3........................... 4 2 9 2.1.................................. 9 2.2...............................
d ϕ i) t d )t0 d ϕi) ϕ i) t x j t d ) ϕ t0 t α dx j d ) ϕ i) t dx t0 j x j d ϕ i) ) t x j dx t0 j f i x j ξ j dx i + ξ i x j dx j f i ξ i x j dx j d )
 23 M R M ϕ : R M M ϕt, x) ϕ t x) ϕ s ϕ t ϕ s+t, ϕ 0 id M M ϕ t M ξ ξ ϕ t d ϕ tx) ξϕ t x)) U, x 1,...,x n )) ϕ t x) ϕ 1) t x),...,ϕ n) t x)), ξx) ξ i x) d ϕi) t x) ξ i ϕ t x)) M f ϕ t f)x) f ϕ t )x) fϕ
23 M R M ϕ : R M M ϕt, x) ϕ t x) ϕ s ϕ t ϕ s+t, ϕ 0 id M M ϕ t M ξ ξ ϕ t d ϕ tx) ξϕ t x)) U, x 1,...,x n )) ϕ t x) ϕ 1) t x),...,ϕ n) t x)), ξx) ξ i x) d ϕi) t x) ξ i ϕ t x)) M f ϕ t f)x) f ϕ t )x) fϕ
I A A441 : April 15, 2013 Version : 1.1 I Kawahira, Tomoki TA (Shigehiro, Yoshida )
 I013 00-1 : April 15, 013 Version : 1.1 I Kawahira, Tomoki TA (Shigehiro, Yoshida) http://www.math.nagoya-u.ac.jp/~kawahira/courses/13s-tenbou.html pdf * 4 15 4 5 13 e πi = 1 5 0 5 7 3 4 6 3 6 10 6 17
I013 00-1 : April 15, 013 Version : 1.1 I Kawahira, Tomoki TA (Shigehiro, Yoshida) http://www.math.nagoya-u.ac.jp/~kawahira/courses/13s-tenbou.html pdf * 4 15 4 5 13 e πi = 1 5 0 5 7 3 4 6 3 6 10 6 17
i Version 1.1, (2012/02/22 24),.,..,.,,. R-space,, ( R- space),, Kahler (Kähler C-space)., R-space,., R-space, Hermite,.
 R-space ( ) Version 1.1 (2012/02/29) i Version 1.1, (2012/02/22 24),.,..,.,,. R-space,, ( R- space),, Kahler (Kähler C-space)., R-space,., R-space, Hermite,. ii 1 Lie 1 1.1 Killing................................
R-space ( ) Version 1.1 (2012/02/29) i Version 1.1, (2012/02/22 24),.,..,.,,. R-space,, ( R- space),, Kahler (Kähler C-space)., R-space,., R-space, Hermite,. ii 1 Lie 1 1.1 Killing................................
LINEAR ALGEBRA I Hiroshi SUZUKI Department of Mathematics International Christian University
 LINEAR ALGEBRA I Hiroshi SUZUKI Department of Mathematics International Christian University 2002 2 2 2 2 22 2 3 3 3 3 3 4 4 5 5 6 6 7 7 8 8 9 Cramer 9 0 0 E-mail:hsuzuki@icuacjp 0 3x + y + 2z 4 x + y
LINEAR ALGEBRA I Hiroshi SUZUKI Department of Mathematics International Christian University 2002 2 2 2 2 22 2 3 3 3 3 3 4 4 5 5 6 6 7 7 8 8 9 Cramer 9 0 0 E-mail:hsuzuki@icuacjp 0 3x + y + 2z 4 x + y
2 7 V 7 {fx fx 3 } 8 P 3 {fx fx 3 } 9 V 9 {fx fx f x 2fx } V {fx fx f x 2fx + } V {{a n } {a n } a n+2 a n+ + a n n } 2 V 2 {{a n } {a n } a n+2 a n+
 R 3 R n C n V??,?? k, l K x, y, z K n, i x + y + z x + y + z iv x V, x + x o x V v kx + y kx + ky vi k + lx kx + lx vii klx klx viii x x ii x + y y + x, V iii o K n, x K n, x + o x iv x K n, x + x o x
R 3 R n C n V??,?? k, l K x, y, z K n, i x + y + z x + y + z iv x V, x + x o x V v kx + y kx + ky vi k + lx kx + lx vii klx klx viii x x ii x + y y + x, V iii o K n, x K n, x + o x iv x K n, x + x o x
Mazur [Ma1] Schlessinger [Sch] [SL] [Ma1] [Ma1] [Ma2] Galois [] 17 R m R R R M End R M) M R ut R M) M R R G R[G] R G Sets 1 Λ Noether Λ k Λ m Λ k C Λ
![Mazur [Ma1] Schlessinger [Sch] [SL] [Ma1] [Ma1] [Ma2] Galois [] 17 R m R R R M End R M) M R ut R M) M R R G R[G] R G Sets 1 Λ Noether Λ k Λ m Λ k C Λ Mazur [Ma1] Schlessinger [Sch] [SL] [Ma1] [Ma1] [Ma2] Galois [] 17 R m R R R M End R M) M R ut R M) M R R G R[G] R G Sets 1 Λ Noether Λ k Λ m Λ k C Λ](/thumbs/78/78261740.jpg) Galois ) 0 1 1 2 2 4 3 10 4 12 5 14 16 0 Galois Galois Galois TaylorWiles Fermat [W][TW] Galois Galois Galois 1 Noether 2 1 Mazur [Ma1] Schlessinger [Sch] [SL] [Ma1] [Ma1] [Ma2] Galois [] 17 R m R R R
Galois ) 0 1 1 2 2 4 3 10 4 12 5 14 16 0 Galois Galois Galois TaylorWiles Fermat [W][TW] Galois Galois Galois 1 Noether 2 1 Mazur [Ma1] Schlessinger [Sch] [SL] [Ma1] [Ma1] [Ma2] Galois [] 17 R m R R R
ad bc A A A = ad bc ( d ) b c a n A n A n A A det A A ( ) a b A = c d det A = ad bc σ {,,,, n} {,,, } {,,, } {,,, } ( ) σ = σ() = σ() = n sign σ sign(
 I n n A AX = I, YA = I () n XY A () X = IX = (YA)X = Y(AX) = YI = Y X Y () XY A A AB AB BA (AB)(B A ) = A(BB )A = AA = I (BA)(A B ) = B(AA )B = BB = I (AB) = B A (BA) = A B A B A = B = 5 5 A B AB BA A
I n n A AX = I, YA = I () n XY A () X = IX = (YA)X = Y(AX) = YI = Y X Y () XY A A AB AB BA (AB)(B A ) = A(BB )A = AA = I (BA)(A B ) = B(AA )B = BB = I (AB) = B A (BA) = A B A B A = B = 5 5 A B AB BA A
II R n k +1 v 0,, v k k v 1 v 0,, v k v v 0,, v k R n 1 a 0,, a k a 0 v 0 + a k v k v 0 v k k k v 0,, v k σ k σ dimσ = k 1.3. k
 II 231017 1 1.1. R n k +1 v 0,, v k k v 1 v 0,, v k v 0 1.2. v 0,, v k R n 1 a 0,, a k a 0 v 0 + a k v k v 0 v k k k v 0,, v k σ kσ dimσ = k 1.3. k σ {v 0,...,v k } {v i0,...,v il } l σ τ < τ τ σ 1.4.
II 231017 1 1.1. R n k +1 v 0,, v k k v 1 v 0,, v k v 0 1.2. v 0,, v k R n 1 a 0,, a k a 0 v 0 + a k v k v 0 v k k k v 0,, v k σ kσ dimσ = k 1.3. k σ {v 0,...,v k } {v i0,...,v il } l σ τ < τ τ σ 1.4.
II (Percolation) ( 3-4 ) 1. [ ],,,,,,,. 2. [ ],.. 3. [ ],. 4. [ ] [ ] G. Grimmett Percolation Springer-Verlag New-York [ ] 3
![II (Percolation) ( 3-4 ) 1. [ ],,,,,,,. 2. [ ],.. 3. [ ],. 4. [ ] [ ] G. Grimmett Percolation Springer-Verlag New-York [ ] 3 II (Percolation) ( 3-4 ) 1. [ ],,,,,,,. 2. [ ],.. 3. [ ],. 4. [ ] [ ] G. Grimmett Percolation Springer-Verlag New-York [ ] 3](/thumbs/94/121840826.jpg) II (Percolation) 12 9 27 ( 3-4 ) 1 [ ] 2 [ ] 3 [ ] 4 [ ] 1992 5 [ ] G Grimmett Percolation Springer-Verlag New-York 1989 6 [ ] 3 1 3 p H 2 3 2 FKG BK Russo 2 p H = p T (=: p c ) 3 2 Kesten p c =1/2 ( )
II (Percolation) 12 9 27 ( 3-4 ) 1 [ ] 2 [ ] 3 [ ] 4 [ ] 1992 5 [ ] G Grimmett Percolation Springer-Verlag New-York 1989 6 [ ] 3 1 3 p H 2 3 2 FKG BK Russo 2 p H = p T (=: p c ) 3 2 Kesten p c =1/2 ( )
y π π O π x 9 s94.5 y dy dx. y = x + 3 y = x logx + 9 s9.6 z z x, z y. z = xy + y 3 z = sinx y 9 s x dx π x cos xdx 9 s93.8 a, fx = e x ax,. a =
 [ ] 9 IC. dx = 3x 4y dt dy dt = x y u xt = expλt u yt λ u u t = u u u + u = xt yt 6 3. u = x, y, z = x + y + z u u 9 s9 grad u ux, y, z = c c : grad u = u x i + u y j + u k i, j, k z x, y, z grad u v =
[ ] 9 IC. dx = 3x 4y dt dy dt = x y u xt = expλt u yt λ u u t = u u u + u = xt yt 6 3. u = x, y, z = x + y + z u u 9 s9 grad u ux, y, z = c c : grad u = u x i + u y j + u k i, j, k z x, y, z grad u v =
 006 11 8 0 3 1 5 1.1..................... 5 1......................... 6 1.3.................... 6 1.4.................. 8 1.5................... 8 1.6................... 10 1.6.1......................
006 11 8 0 3 1 5 1.1..................... 5 1......................... 6 1.3.................... 6 1.4.................. 8 1.5................... 8 1.6................... 10 1.6.1......................
TOP URL 1
 TOP URL http://amonphys.web.fc.com/ 3.............................. 3.............................. 4.3 4................... 5.4........................ 6.5........................ 8.6...........................7
TOP URL http://amonphys.web.fc.com/ 3.............................. 3.............................. 4.3 4................... 5.4........................ 6.5........................ 8.6...........................7
chap1.dvi
 1 1 007 1 e iθ = cos θ + isin θ 1) θ = π e iπ + 1 = 0 1 ) 3 11 f 0 r 1 1 ) k f k = 1 + r) k f 0 f k k = 01) f k+1 = 1 + r)f k ) f k+1 f k = rf k 3) 1 ) ) ) 1+r/)f 0 1 1 + r/) f 0 = 1 + r + r /4)f 0 1 f
1 1 007 1 e iθ = cos θ + isin θ 1) θ = π e iπ + 1 = 0 1 ) 3 11 f 0 r 1 1 ) k f k = 1 + r) k f 0 f k k = 01) f k+1 = 1 + r)f k ) f k+1 f k = rf k 3) 1 ) ) ) 1+r/)f 0 1 1 + r/) f 0 = 1 + r + r /4)f 0 1 f
液晶の物理1:連続体理論(弾性,粘性)
 The Physics of Liquid Crystals P. G. de Gennes and J. Prost (Oxford University Press, 1993) Liquid crystals are beautiful and mysterious; I am fond of them for both reasons. My hope is that some readers
The Physics of Liquid Crystals P. G. de Gennes and J. Prost (Oxford University Press, 1993) Liquid crystals are beautiful and mysterious; I am fond of them for both reasons. My hope is that some readers
1 (Berry,1975) 2-6 p (S πr 2 )p πr 2 p 2πRγ p p = 2γ R (2.5).1-1 : : : : ( ).2 α, β α, β () X S = X X α X β (.1) 1 2
 2005 9/8-11 2 2.2 ( 2-5) γ ( ) γ cos θ 2πr πρhr 2 g h = 2γ cos θ ρgr (2.1) γ = ρgrh (2.2) 2 cos θ θ cos θ = 1 (2.2) γ = 1 ρgrh (2.) 2 2. p p ρgh p ( ) p p = p ρgh (2.) h p p = 2γ r 1 1 (Berry,1975) 2-6
2005 9/8-11 2 2.2 ( 2-5) γ ( ) γ cos θ 2πr πρhr 2 g h = 2γ cos θ ρgr (2.1) γ = ρgrh (2.2) 2 cos θ θ cos θ = 1 (2.2) γ = 1 ρgrh (2.) 2 2. p p ρgh p ( ) p p = p ρgh (2.) h p p = 2γ r 1 1 (Berry,1975) 2-6
1 8, : 8.1 1, 2 z = ax + by + c ax by + z c = a b +1 x y z c = 0, (0, 0, c), n = ( a, b, 1). f = n i=1 a ii x 2 i + i<j 2a ij x i x j = ( x, A x), f =
 1 8, : 8.1 1, z = ax + by + c ax by + z c = a b +1 x y z c = 0, (0, 0, c), n = ( a, b, 1). f = a ii x i + i
1 8, : 8.1 1, z = ax + by + c ax by + z c = a b +1 x y z c = 0, (0, 0, c), n = ( a, b, 1). f = a ii x i + i
II (10 4 ) 1. p (x, y) (a, b) ε(x, y; a, b) 0 f (x, y) f (a, b) A, B (6.5) y = b f (x, b) f (a, b) x a = A + ε(x, b; a, b) x a 2 x a 0 A = f x (
 II (1 4 ) 1. p.13 1 (x, y) (a, b) ε(x, y; a, b) f (x, y) f (a, b) A, B (6.5) y = b f (x, b) f (a, b) x a = A + ε(x, b; a, b) x a x a A = f x (a, b) y x 3 3y 3 (x, y) (, ) f (x, y) = x + y (x, y) = (, )
II (1 4 ) 1. p.13 1 (x, y) (a, b) ε(x, y; a, b) f (x, y) f (a, b) A, B (6.5) y = b f (x, b) f (a, b) x a = A + ε(x, b; a, b) x a x a A = f x (a, b) y x 3 3y 3 (x, y) (, ) f (x, y) = x + y (x, y) = (, )
1990 IMO 1990/1/15 1:00-4:00 1 N N N 1, N 1 N 2, N 2 N 3 N 3 2 x x + 52 = 3 x x , A, B, C 3,, A B, C 2,,,, 7, A, B, C
 0 9 (1990 1999 ) 10 (2000 ) 1900 1994 1995 1999 2 SAT ACT 1 1990 IMO 1990/1/15 1:00-4:00 1 N 1990 9 N N 1, N 1 N 2, N 2 N 3 N 3 2 x 2 + 25x + 52 = 3 x 2 + 25x + 80 3 2, 3 0 4 A, B, C 3,, A B, C 2,,,, 7,
0 9 (1990 1999 ) 10 (2000 ) 1900 1994 1995 1999 2 SAT ACT 1 1990 IMO 1990/1/15 1:00-4:00 1 N 1990 9 N N 1, N 1 N 2, N 2 N 3 N 3 2 x 2 + 25x + 52 = 3 x 2 + 25x + 80 3 2, 3 0 4 A, B, C 3,, A B, C 2,,,, 7,
2 1 1 α = a + bi(a, b R) α (conjugate) α = a bi α (absolute value) α = a 2 + b 2 α (norm) N(α) = a 2 + b 2 = αα = α 2 α (spure) (trace) 1 1. a R aα =
 1 1 α = a + bi(a, b R) α (conjugate) α = a bi α (absolute value) α = a + b α (norm) N(α) = a + b = αα = α α (spure) (trace) 1 1. a R aα = aα. α = α 3. α + β = α + β 4. αβ = αβ 5. β 0 6. α = α ( ) α = α
1 1 α = a + bi(a, b R) α (conjugate) α = a bi α (absolute value) α = a + b α (norm) N(α) = a + b = αα = α α (spure) (trace) 1 1. a R aα = aα. α = α 3. α + β = α + β 4. αβ = αβ 5. β 0 6. α = α ( ) α = α
73
 73 74 ( u w + bw) d = Ɣ t tw dɣ u = N u + N u + N 3 u 3 + N 4 u 4 + [K ] {u = {F 75 u δu L σ (L) σ dx σ + dσ x δu b δu + d(δu) ALW W = L b δu dv + Aσ (L)δu(L) δu = (= ) W = A L b δu dx + Aσ (L)δu(L) Aσ
73 74 ( u w + bw) d = Ɣ t tw dɣ u = N u + N u + N 3 u 3 + N 4 u 4 + [K ] {u = {F 75 u δu L σ (L) σ dx σ + dσ x δu b δu + d(δu) ALW W = L b δu dv + Aσ (L)δu(L) δu = (= ) W = A L b δu dx + Aσ (L)δu(L) Aσ
λ n numbering Num(λ) Young numbering T i j T ij Young T (content) cont T (row word) word T µ n S n µ C(µ) 0.2. Young λ, µ n Kostka K µλ K µλ def = #{T
 0 2 8 8 6 3 0 0 Young Young [F] 0.. Young λ n λ n λ = (λ,, λ l ) λ λ 2 λ l λ = ( m, 2 m 2, ) λ = n, l(λ) = l {λ n n 0} P λ = (λ, ), µ = (µ, ) n λ µ k k k λ i µ i λ µ λ = µ k i= i= i < k λ i = µ i λ k >
0 2 8 8 6 3 0 0 Young Young [F] 0.. Young λ n λ n λ = (λ,, λ l ) λ λ 2 λ l λ = ( m, 2 m 2, ) λ = n, l(λ) = l {λ n n 0} P λ = (λ, ), µ = (µ, ) n λ µ k k k λ i µ i λ µ λ = µ k i= i= i < k λ i = µ i λ k >
さくらの個別指導 ( さくら教育研究所 ) 1 φ = φ 1 : φ [ ] a [ ] 1 a : b a b b(a + b) b a 2 a 2 = b(a + b). b 2 ( a b ) 2 = a b a/b X 2 X 1 = 0 a/b > 0 2 a
![さくらの個別指導 ( さくら教育研究所 ) 1 φ = φ 1 : φ [ ] a [ ] 1 a : b a b b(a + b) b a 2 a 2 = b(a + b). b 2 ( a b ) 2 = a b a/b X 2 X 1 = 0 a/b > 0 2 a さくらの個別指導 ( さくら教育研究所 ) 1 φ = φ 1 : φ [ ] a [ ] 1 a : b a b b(a + b) b a 2 a 2 = b(a + b). b 2 ( a b ) 2 = a b a/b X 2 X 1 = 0 a/b > 0 2 a](/thumbs/97/131721994.jpg) φ + 5 2 φ : φ [ ] a [ ] a : b a b b(a + b) b a 2 a 2 b(a + b). b 2 ( a b ) 2 a b + a/b X 2 X 0 a/b > 0 2 a b + 5 2 φ φ : 2 5 5 [ ] [ ] x x x : x : x x : x x : x x 2 x 2 x 0 x ± 5 2 x x φ : φ 2 : φ ( )
φ + 5 2 φ : φ [ ] a [ ] a : b a b b(a + b) b a 2 a 2 b(a + b). b 2 ( a b ) 2 a b + a/b X 2 X 0 a/b > 0 2 a b + 5 2 φ φ : 2 5 5 [ ] [ ] x x x : x : x x : x x : x x 2 x 2 x 0 x ± 5 2 x x φ : φ 2 : φ ( )
ii p ϕ x, t = C ϕ xe i ħ E t +C ϕ xe i ħ E t ψ x,t ψ x,t p79 やは時間変化しないことに注意 振動 粒子はだいたい このあたりにいる 粒子はだいたい このあたりにいる p35 D.3 Aψ Cϕdx = aψ ψ C Aϕ dx
 i B5 7.8. p89 4. ψ x, tψx, t = ψ R x, t iψ I x, t ψ R x, t + iψ I x, t = ψ R x, t + ψ I x, t p 5.8 π π π F e ix + F e ix + F 3 e 3ix F e ix + F e ix + F 3 e 3ix dx πψ x πψx p39 7. AX = X A [ a b c d x
i B5 7.8. p89 4. ψ x, tψx, t = ψ R x, t iψ I x, t ψ R x, t + iψ I x, t = ψ R x, t + ψ I x, t p 5.8 π π π F e ix + F e ix + F 3 e 3ix F e ix + F e ix + F 3 e 3ix dx πψ x πψx p39 7. AX = X A [ a b c d x
Mathematical Logic I 12 Contents I Zorn
 Mathematical Logic I 12 Contents I 2 1 3 1.1............................. 3 1.2.......................... 5 1.3 Zorn.................. 5 2 6 2.1.............................. 6 2.2..............................
Mathematical Logic I 12 Contents I 2 1 3 1.1............................. 3 1.2.......................... 5 1.3 Zorn.................. 5 2 6 2.1.............................. 6 2.2..............................
5. [1 ] 1 [], u(x, t) t c u(x, t) x (5.3) ξ x + ct, η x ct (5.4),u(x, t) ξ, η u(ξ, η), ξ t,, ( u(ξ,η) ξ η u(x, t) t ) u(x, t) { ( u(ξ, η) c t ξ ξ { (
![5. [1 ] 1 [], u(x, t) t c u(x, t) x (5.3) ξ x + ct, η x ct (5.4),u(x, t) ξ, η u(ξ, η), ξ t,, ( u(ξ,η) ξ η u(x, t) t ) u(x, t) { ( u(ξ, η) c t ξ ξ { ( 5. [1 ] 1 [], u(x, t) t c u(x, t) x (5.3) ξ x + ct, η x ct (5.4),u(x, t) ξ, η u(ξ, η), ξ t,, ( u(ξ,η) ξ η u(x, t) t ) u(x, t) { ( u(ξ, η) c t ξ ξ { (](/thumbs/92/107866626.jpg) 5 5.1 [ ] ) d f(t) + a d f(t) + bf(t) : f(t) 1 dt dt ) u(x, t) c u(x, t) : u(x, t) t x : ( ) ) 1 : y + ay, : y + ay + by : ( ) 1 ) : y + ay, : yy + ay 3 ( ): ( ) ) : y + ay, : y + ay b [],,, [ ] au xx
5 5.1 [ ] ) d f(t) + a d f(t) + bf(t) : f(t) 1 dt dt ) u(x, t) c u(x, t) : u(x, t) t x : ( ) ) 1 : y + ay, : y + ay + by : ( ) 1 ) : y + ay, : yy + ay 3 ( ): ( ) ) : y + ay, : y + ay b [],,, [ ] au xx
Jacobi, Stieltjes, Gauss : :
 Jacobi, Stieltjes, Gauss : : 28 2 0 894 T. J. Stieltjes [St94a] Recherches sur les fractions continues Stieltjes 0 f(u)du, z + u f(u) > 0, z C z + + a a 2 z + a 3 +..., a p > 0 (a) Vitali (a) Stieltjes
Jacobi, Stieltjes, Gauss : : 28 2 0 894 T. J. Stieltjes [St94a] Recherches sur les fractions continues Stieltjes 0 f(u)du, z + u f(u) > 0, z C z + + a a 2 z + a 3 +..., a p > 0 (a) Vitali (a) Stieltjes
x = a 1 f (a r, a + r) f(a) r a f f(a) 2 2. (a, b) 2 f (a, b) r f(a, b) r (a, b) f f(a, b)
 2011 I 2 II III 17, 18, 19 7 7 1 2 2 2 1 2 1 1 1.1.............................. 2 1.2 : 1.................... 4 1.2.1 2............................... 5 1.3 : 2.................... 5 1.3.1 2.....................................
2011 I 2 II III 17, 18, 19 7 7 1 2 2 2 1 2 1 1 1.1.............................. 2 1.2 : 1.................... 4 1.2.1 2............................... 5 1.3 : 2.................... 5 1.3.1 2.....................................
koji07-01.dvi
 2007 I II III 1, 2, 3, 4, 5, 6, 7 5 10 19 (!) 1938 70 21? 1 1 2 1 2 2 1! 4, 5 1? 50 1 2 1 1 2 2 1?? 2 1 1, 2 1, 2 1, 2, 3,... 3 1, 2 1, 3? 2 1 3 1 2 1 1, 2 2, 3? 2 1 3 2 3 2 k,l m, n k,l m, n kn > ml...?
2007 I II III 1, 2, 3, 4, 5, 6, 7 5 10 19 (!) 1938 70 21? 1 1 2 1 2 2 1! 4, 5 1? 50 1 2 1 1 2 2 1?? 2 1 1, 2 1, 2 1, 2, 3,... 3 1, 2 1, 3? 2 1 3 1 2 1 1, 2 2, 3? 2 1 3 2 3 2 k,l m, n k,l m, n kn > ml...?
January 27, 2015
 e-mail : kigami@i.kyoto-u.ac.jp January 27, 205 Contents 2........................ 2.2....................... 3.3....................... 6.4......................... 2 6 2........................... 6
e-mail : kigami@i.kyoto-u.ac.jp January 27, 205 Contents 2........................ 2.2....................... 3.3....................... 6.4......................... 2 6 2........................... 6
Z: Q: R: C:
 0 Z: Q: R: C: 3 4 4 4................................ 4 4.................................. 7 5 3 5...................... 3 5......................... 40 5.3 snz) z)........................... 4 6 46 x
0 Z: Q: R: C: 3 4 4 4................................ 4 4.................................. 7 5 3 5...................... 3 5......................... 40 5.3 snz) z)........................... 4 6 46 x
1 1.1 R (ring) R1 R4 R1 R (commutative [abelian] group) R2 a, b, c R (ab)c = a(bc) (associative law) R3 a, b, c R a(b + c) = ab + ac, (a + b)c = ac +
![1 1.1 R (ring) R1 R4 R1 R (commutative [abelian] group) R2 a, b, c R (ab)c = a(bc) (associative law) R3 a, b, c R a(b + c) = ab + ac, (a + b)c = ac + 1 1.1 R (ring) R1 R4 R1 R (commutative [abelian] group) R2 a, b, c R (ab)c = a(bc) (associative law) R3 a, b, c R a(b + c) = ab + ac, (a + b)c = ac +](/thumbs/74/70287522.jpg) ALGEBRA II Hiroshi SUZUKI Department of Mathematics International Christian University 2004 1 1 1 2 2 1 3 3 1 4 4 1 5 5 1 6 6 1 7 7 1 7.1....................... 7 1 7.2........................... 7 4 8
ALGEBRA II Hiroshi SUZUKI Department of Mathematics International Christian University 2004 1 1 1 2 2 1 3 3 1 4 4 1 5 5 1 6 6 1 7 7 1 7.1....................... 7 1 7.2........................... 7 4 8
 2016 1. 2. 3. 4. 5. 6. 7. 8. 9. 10. 1 16 2 1 () X O 3 (O1) X O, O (O2) O O (O3) O O O X (X, O) O X X (O1), (O2), (O3) (O2) (O3) n (O2) U 1,..., U n O U k O k=1 (O3) U λ O( λ Λ) λ Λ U λ O 0 X 0 (O2) n =
2016 1. 2. 3. 4. 5. 6. 7. 8. 9. 10. 1 16 2 1 () X O 3 (O1) X O, O (O2) O O (O3) O O O X (X, O) O X X (O1), (O2), (O3) (O2) (O3) n (O2) U 1,..., U n O U k O k=1 (O3) U λ O( λ Λ) λ Λ U λ O 0 X 0 (O2) n =
1 1 n 0, 1, 2,, n n 2 a, b a n b n a, b n a b (mod n) 1 1. n = (mod 10) 2. n = (mod 9) n II Z n := {0, 1, 2,, n 1} 1.
 1 1 n 0, 1, 2,, n 1 1.1 n 2 a, b a n b n a, b n a b (mod n) 1 1. n = 10 1567 237 (mod 10) 2. n = 9 1567 1826578 (mod 9) n II Z n := {0, 1, 2,, n 1} 1.2 a b a = bq + r (0 r < b) q, r q a b r 2 1. a = 456,
1 1 n 0, 1, 2,, n 1 1.1 n 2 a, b a n b n a, b n a b (mod n) 1 1. n = 10 1567 237 (mod 10) 2. n = 9 1567 1826578 (mod 9) n II Z n := {0, 1, 2,, n 1} 1.2 a b a = bq + r (0 r < b) q, r q a b r 2 1. a = 456,
,2,4
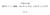 2005 12 2006 1,2,4 iii 1 Hilbert 14 1 1.............................................. 1 2............................................... 2 3............................................... 3 4.............................................
2005 12 2006 1,2,4 iii 1 Hilbert 14 1 1.............................................. 1 2............................................... 2 3............................................... 3 4.............................................
平成 15 年度 ( 第 25 回 ) 数学入門公開講座テキスト ( 京都大学数理解析研究所, 平成 ~8 15 月年 78 日開催月 4 日 ) X 2 = 1 ( ) f 1 (X 1,..., X n ) = 0,..., f r (X 1,..., X n ) = 0 X = (
 1 1.1 X 2 = 1 ( ) f 1 (X 1,..., X n ) = 0,..., f r (X 1,..., X n ) = 0 X = (X 1,..., X n ) ( ) X 1,..., X n f 1,..., f r A T X + XA XBR 1 B T X + C T QC = O X 1.2 X 1,..., X n X i X j X j X i = 0, P i
1 1.1 X 2 = 1 ( ) f 1 (X 1,..., X n ) = 0,..., f r (X 1,..., X n ) = 0 X = (X 1,..., X n ) ( ) X 1,..., X n f 1,..., f r A T X + XA XBR 1 B T X + C T QC = O X 1.2 X 1,..., X n X i X j X j X i = 0, P i
2000年度『数学展望 I』講義録
 2000 I I IV I II 2000 I I IV I-IV. i ii 3.10 (http://www.math.nagoya-u.ac.jp/ kanai/) 2000 A....1 B....4 C....10 D....13 E....17 Brouwer A....21 B....26 C....33 D....39 E. Sperner...45 F....48 A....53
2000 I I IV I II 2000 I I IV I-IV. i ii 3.10 (http://www.math.nagoya-u.ac.jp/ kanai/) 2000 A....1 B....4 C....10 D....13 E....17 Brouwer A....21 B....26 C....33 D....39 E. Sperner...45 F....48 A....53
) ] [ h m x + y + + V x) φ = Eφ 1) z E = i h t 13) x << 1) N n n= = N N + 1) 14) N n n= = N N + 1)N + 1) 6 15) N n 3 n= = 1 4 N N + 1) 16) N n 4
![) ] [ h m x + y + + V x) φ = Eφ 1) z E = i h t 13) x << 1) N n n= = N N + 1) 14) N n n= = N N + 1)N + 1) 6 15) N n 3 n= = 1 4 N N + 1) 16) N n 4 ) ] [ h m x + y + + V x) φ = Eφ 1) z E = i h t 13) x << 1) N n n= = N N + 1) 14) N n n= = N N + 1)N + 1) 6 15) N n 3 n= = 1 4 N N + 1) 16) N n 4](/thumbs/92/107866707.jpg) 1. k λ ν ω T v p v g k = π λ ω = πν = π T v p = λν = ω k v g = dω dk 1) ) 3) 4). p = hk = h λ 5) E = hν = hω 6) h = h π 7) h =6.6618 1 34 J sec) hc=197.3 MeV fm = 197.3 kev pm= 197.3 ev nm = 1.97 1 3 ev
1. k λ ν ω T v p v g k = π λ ω = πν = π T v p = λν = ω k v g = dω dk 1) ) 3) 4). p = hk = h λ 5) E = hν = hω 6) h = h π 7) h =6.6618 1 34 J sec) hc=197.3 MeV fm = 197.3 kev pm= 197.3 ev nm = 1.97 1 3 ev
24 I ( ) 1. R 3 (i) C : x 2 + y 2 1 = 0 (ii) C : y = ± 1 x 2 ( 1 x 1) (iii) C : x = cos t, y = sin t (0 t 2π) 1.1. γ : [a, b] R n ; t γ(t) = (x
![24 I ( ) 1. R 3 (i) C : x 2 + y 2 1 = 0 (ii) C : y = ± 1 x 2 ( 1 x 1) (iii) C : x = cos t, y = sin t (0 t 2π) 1.1. γ : [a, b] R n ; t γ(t) = (x 24 I ( ) 1. R 3 (i) C : x 2 + y 2 1 = 0 (ii) C : y = ± 1 x 2 ( 1 x 1) (iii) C : x = cos t, y = sin t (0 t 2π) 1.1. γ : [a, b] R n ; t γ(t) = (x](/thumbs/94/118744578.jpg) 24 I 1.1.. ( ) 1. R 3 (i) C : x 2 + y 2 1 = 0 (ii) C : y = ± 1 x 2 ( 1 x 1) (iii) C : x = cos t, y = sin t (0 t 2π) 1.1. γ : [a, b] R n ; t γ(t) = (x 1 (t), x 2 (t),, x n (t)) ( ) ( ), γ : (i) x 1 (t),
24 I 1.1.. ( ) 1. R 3 (i) C : x 2 + y 2 1 = 0 (ii) C : y = ± 1 x 2 ( 1 x 1) (iii) C : x = cos t, y = sin t (0 t 2π) 1.1. γ : [a, b] R n ; t γ(t) = (x 1 (t), x 2 (t),, x n (t)) ( ) ( ), γ : (i) x 1 (t),
 ( 9 1 ) 1 2 1.1................................... 2 1.2................................................. 3 1.3............................................... 4 1.4...........................................
( 9 1 ) 1 2 1.1................................... 2 1.2................................................. 3 1.3............................................... 4 1.4...........................................
微分積分 サンプルページ この本の定価 判型などは, 以下の URL からご覧いただけます. このサンプルページの内容は, 初版 1 刷発行時のものです.
 微分積分 サンプルページ この本の定価 判型などは, 以下の URL からご覧いただけます. ttp://www.morikita.co.jp/books/mid/00571 このサンプルページの内容は, 初版 1 刷発行時のものです. i ii 014 10 iii [note] 1 3 iv 4 5 3 6 4 x 0 sin x x 1 5 6 z = f(x, y) 1 y = f(x)
微分積分 サンプルページ この本の定価 判型などは, 以下の URL からご覧いただけます. ttp://www.morikita.co.jp/books/mid/00571 このサンプルページの内容は, 初版 1 刷発行時のものです. i ii 014 10 iii [note] 1 3 iv 4 5 3 6 4 x 0 sin x x 1 5 6 z = f(x, y) 1 y = f(x)
( )
 7..-8..8.......................................................................... 4.................................... 3...................................... 3..3.................................. 4.3....................................
7..-8..8.......................................................................... 4.................................... 3...................................... 3..3.................................. 4.3....................................
9. 05 L x P(x) P(0) P(x) u(x) u(x) (0 < = x < = L) P(x) E(x) A(x) P(L) f ( d EA du ) = 0 (9.) dx dx u(0) = 0 (9.2) E(L)A(L) du (L) = f (9.3) dx (9.) P
 9 (Finite Element Method; FEM) 9. 9. P(0) P(x) u(x) (a) P(L) f P(0) P(x) (b) 9. P(L) 9. 05 L x P(x) P(0) P(x) u(x) u(x) (0 < = x < = L) P(x) E(x) A(x) P(L) f ( d EA du ) = 0 (9.) dx dx u(0) = 0 (9.2) E(L)A(L)
9 (Finite Element Method; FEM) 9. 9. P(0) P(x) u(x) (a) P(L) f P(0) P(x) (b) 9. P(L) 9. 05 L x P(x) P(0) P(x) u(x) u(x) (0 < = x < = L) P(x) E(x) A(x) P(L) f ( d EA du ) = 0 (9.) dx dx u(0) = 0 (9.2) E(L)A(L)
dynamics-solution2.dvi
 1 1. (1) a + b = i +3i + k () a b =5i 5j +3k (3) a b =1 (4) a b = 7i j +1k. a = 14 l =/ 14, m=1/ 14, n=3/ 14 3. 4. 5. df (t) d [a(t)e(t)] =ti +9t j +4k, = d a(t) d[a(t)e(t)] e(t)+ da(t) d f (t) =i +18tj
1 1. (1) a + b = i +3i + k () a b =5i 5j +3k (3) a b =1 (4) a b = 7i j +1k. a = 14 l =/ 14, m=1/ 14, n=3/ 14 3. 4. 5. df (t) d [a(t)e(t)] =ti +9t j +4k, = d a(t) d[a(t)e(t)] e(t)+ da(t) d f (t) =i +18tj
振動と波動
 Report JS0.5 J Simplicity February 4, 2012 1 J Simplicity HOME http://www.jsimplicity.com/ Preface 2 Report 2 Contents I 5 1 6 1.1..................................... 6 1.2 1 1:................ 7 1.3
Report JS0.5 J Simplicity February 4, 2012 1 J Simplicity HOME http://www.jsimplicity.com/ Preface 2 Report 2 Contents I 5 1 6 1.1..................................... 6 1.2 1 1:................ 7 1.3
E1 (4/12)., ( )., 3,4 ( ). ( ) Allen Hatcher, Vector bundle and K-theory ( HP ) 1
 E1 (4/12)., ( )., 3,4 ( ). ( ) Allen Hatcher, Vector bundle and K-theory ( HP ) 1 (4/12) 1 1.. 2. F R C H P n F E n := {((x 0,..., x n ), [v 0 : : v n ]) F n+1 P n F n x i v i = 0 }. i=0 E n P n F P n
E1 (4/12)., ( )., 3,4 ( ). ( ) Allen Hatcher, Vector bundle and K-theory ( HP ) 1 (4/12) 1 1.. 2. F R C H P n F E n := {((x 0,..., x n ), [v 0 : : v n ]) F n+1 P n F n x i v i = 0 }. i=0 E n P n F P n
4. ϵ(ν, T ) = c 4 u(ν, T ) ϵ(ν, T ) T ν π4 Planck dx = 0 e x 1 15 U(T ) x 3 U(T ) = σt 4 Stefan-Boltzmann σ 2π5 k 4 15c 2 h 3 = W m 2 K 4 5.
 A 1. Boltzmann Planck u(ν, T )dν = 8πh ν 3 c 3 kt 1 dν h 6.63 10 34 J s Planck k 1.38 10 23 J K 1 Boltzmann u(ν, T ) T ν e hν c = 3 10 8 m s 1 2. Planck λ = c/ν Rayleigh-Jeans u(ν, T )dν = 8πν2 kt dν c
A 1. Boltzmann Planck u(ν, T )dν = 8πh ν 3 c 3 kt 1 dν h 6.63 10 34 J s Planck k 1.38 10 23 J K 1 Boltzmann u(ν, T ) T ν e hν c = 3 10 8 m s 1 2. Planck λ = c/ν Rayleigh-Jeans u(ν, T )dν = 8πν2 kt dν c
1W II K =25 A (1) office(a439) (2) A4 etc. 12:00-13:30 Cafe David 1 2 TA appointment Cafe D
 1W II K200 : October 6, 2004 Version : 1.2, kawahira@math.nagoa-u.ac.jp, http://www.math.nagoa-u.ac.jp/~kawahira/courses.htm TA M1, m0418c@math.nagoa-u.ac.jp TA Talor Jacobian 4 45 25 30 20 K2-1W04-00
1W II K200 : October 6, 2004 Version : 1.2, kawahira@math.nagoa-u.ac.jp, http://www.math.nagoa-u.ac.jp/~kawahira/courses.htm TA M1, m0418c@math.nagoa-u.ac.jp TA Talor Jacobian 4 45 25 30 20 K2-1W04-00
V(x) m e V 0 cos x π x π V(x) = x < π, x > π V 0 (i) x = 0 (V(x) V 0 (1 x 2 /2)) n n d 2 f dξ 2ξ d f 2 dξ + 2n f = 0 H n (ξ) (ii) H
 199 1 1 199 1 1. Vx) m e V cos x π x π Vx) = x < π, x > π V i) x = Vx) V 1 x /)) n n d f dξ ξ d f dξ + n f = H n ξ) ii) H n ξ) = 1) n expξ ) dn dξ n exp ξ )) H n ξ)h m ξ) exp ξ )dξ = π n n!δ n,m x = Vx)
199 1 1 199 1 1. Vx) m e V cos x π x π Vx) = x < π, x > π V i) x = Vx) V 1 x /)) n n d f dξ ξ d f dξ + n f = H n ξ) ii) H n ξ) = 1) n expξ ) dn dξ n exp ξ )) H n ξ)h m ξ) exp ξ )dξ = π n n!δ n,m x = Vx)
